Theorem isoun 29479

Description: Infer an isomorphism from a union of two isomorphisms. (Contributed by Thierry Arnoux, 30-Mar-2017.)

Hypotheses

Ref	Expression
isoun.1	|- ( ph -> H Isom R , S ( A , B ) )
isoun.2	|- ( ph -> G Isom R , S ( C , D ) )
isoun.3	|- ( ( ph /\ x e. A /\ y e. C ) -> x R y )
isoun.4	|- ( ( ph /\ z e. B /\ w e. D ) -> z S w )
isoun.5	|- ( ( ph /\ x e. C /\ y e. A ) -> -. x R y )
isoun.6	|- ( ( ph /\ z e. D /\ w e. B ) -> -. z S w )
isoun.7	|- ( ph -> ( A i^i C ) = (/) )
isoun.8	|- ( ph -> ( B i^i D ) = (/) )

Assertion

Ref	Expression
isoun	|- ( ph -> ( H u. G ) Isom R , S ( ( A u. C ) , ( B u. D ) ) )

Distinct variable groups:   x, y, A   x, w, y, z, B   x, C, y   w, D, x, y, z   w, G, x, y, z   w, H, x, y, z   x, R, y   w, S, x, y, z   ph, w, x, y, z

Allowed substitution hints:   A( z, w)   C( z, w)   R( z, w)

Proof of Theorem isoun

Step	Hyp	Ref	Expression
1		isoun.1	. . . 4 |- ( ph -> H Isom R , S ( A , B ) )
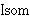
2		isof1o 6573	. . . 4 |- ( H Isom R , S ( A , B ) -> H : A -1-1-onto-> B )
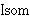3	1, 2	syl 17	. . 3 |- ( ph -> H : A -1-1-onto-> B )
4		isoun.2	. . . 4 |- ( ph -> G Isom R , S ( C , D ) )
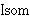
5		isof1o 6573	. . . 4 |- ( G Isom R , S ( C , D ) -> G : C -1-1-onto-> D )
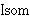6	4, 5	syl 17	. . 3 |- ( ph -> G : C -1-1-onto-> D )
7		isoun.7	. . 3 |- ( ph -> ( A i^i C ) = (/) )
8		isoun.8	. . 3 |- ( ph -> ( B i^i D ) = (/) )
9		f1oun 6156	. . 3 |- ( ( ( H : A -1-1-onto-> B /\ G : C -1-1-onto-> D ) /\ ( ( A i^i C ) = (/) /\ ( B i^i D ) = (/) ) ) -> ( H u. G ) : ( A u. C ) -1-1-onto-> ( B u. D ) )
10	3, 6, 7, 8, 9	syl22anc 1327	. 2 |- ( ph -> ( H u. G ) : ( A u. C ) -1-1-onto-> ( B u. D ) )
11		elun 3753	. . . . 5 |- ( x e. ( A u. C ) <-> ( x e. A \/ x e. C ) )
12		elun 3753	. . . . . . . 8 |- ( y e. ( A u. C ) <-> ( y e. A \/ y e. C ) )
13		isorel 6576	. . . . . . . . . . . 12 |- ( ( H Isom R , S ( A , B ) /\ ( x e. A /\ y e. A ) ) -> ( x R y <-> ( H ` x ) S ( H ` y ) ) )
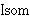
14	1, 13	sylan 488	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. A /\ y e. A ) ) -> ( x R y <-> ( H ` x ) S ( H ` y ) ) )
15		f1ofn 6138	. . . . . . . . . . . . . . . 16 |- ( H : A -1-1-onto-> B -> H Fn A )
16	3, 15	syl 17	. . . . . . . . . . . . . . 15 |- ( ph -> H Fn A )
17	16	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ x e. A ) -> H Fn A )
18		f1ofn 6138	. . . . . . . . . . . . . . . 16 |- ( G : C -1-1-onto-> D -> G Fn C )
19	6, 18	syl 17	. . . . . . . . . . . . . . 15 |- ( ph -> G Fn C )
20	19	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ x e. A ) -> G Fn C )
21	7	anim1i 592	. . . . . . . . . . . . . 14 |- ( ( ph /\ x e. A ) -> ( ( A i^i C ) = (/) /\ x e. A ) )
22		fvun1 6269	. . . . . . . . . . . . . 14 |- ( ( H Fn A /\ G Fn C /\ ( ( A i^i C ) = (/) /\ x e. A ) ) -> ( ( H u. G ) ` x ) = ( H ` x ) )
23	17, 20, 21, 22	syl3anc 1326	. . . . . . . . . . . . 13 |- ( ( ph /\ x e. A ) -> ( ( H u. G ) ` x ) = ( H ` x ) )
24	23	adantrr 753	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. A /\ y e. A ) ) -> ( ( H u. G ) ` x ) = ( H ` x ) )
25	16	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ y e. A ) -> H Fn A )
26	19	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ y e. A ) -> G Fn C )
27	7	anim1i 592	. . . . . . . . . . . . . 14 |- ( ( ph /\ y e. A ) -> ( ( A i^i C ) = (/) /\ y e. A ) )
28		fvun1 6269	. . . . . . . . . . . . . 14 |- ( ( H Fn A /\ G Fn C /\ ( ( A i^i C ) = (/) /\ y e. A ) ) -> ( ( H u. G ) ` y ) = ( H ` y ) )
29	25, 26, 27, 28	syl3anc 1326	. . . . . . . . . . . . 13 |- ( ( ph /\ y e. A ) -> ( ( H u. G ) ` y ) = ( H ` y ) )
30	29	adantrl 752	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. A /\ y e. A ) ) -> ( ( H u. G ) ` y ) = ( H ` y ) )
31	24, 30	breq12d 4666	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. A /\ y e. A ) ) -> ( ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) <-> ( H ` x ) S ( H ` y ) ) )
32	14, 31	bitr4d 271	. . . . . . . . . 10 |- ( ( ph /\ ( x e. A /\ y e. A ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
33	32	anassrs 680	. . . . . . . . 9 |- ( ( ( ph /\ x e. A ) /\ y e. A ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
34		isoun.3	. . . . . . . . . . . 12 |- ( ( ph /\ x e. A /\ y e. C ) -> x R y )
35	34	3expb 1266	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> x R y )
36		isoun.4	. . . . . . . . . . . . . . . . 17 |- ( ( ph /\ z e. B /\ w e. D ) -> z S w )
37	36	3expia 1267	. . . . . . . . . . . . . . . 16 |- ( ( ph /\ z e. B ) -> ( w e. D -> z S w ) )
38	37	ralrimiv 2965	. . . . . . . . . . . . . . 15 |- ( ( ph /\ z e. B ) -> A. w e. D z S w )
39	38	ralrimiva 2966	. . . . . . . . . . . . . 14 |- ( ph -> A. z e. B A. w e. D z S w )
40	39	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> A. z e. B A. w e. D z S w )
41		f1of 6137	. . . . . . . . . . . . . . . . 17 |- ( H : A -1-1-onto-> B -> H : A --> B )
42	3, 41	syl 17	. . . . . . . . . . . . . . . 16 |- ( ph -> H : A --> B )
43	42	ffvelrnda 6359	. . . . . . . . . . . . . . 15 |- ( ( ph /\ x e. A ) -> ( H ` x ) e. B )
44	43	adantrr 753	. . . . . . . . . . . . . 14 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( H ` x ) e. B )
45		f1of 6137	. . . . . . . . . . . . . . . . 17 |- ( G : C -1-1-onto-> D -> G : C --> D )
46	6, 45	syl 17	. . . . . . . . . . . . . . . 16 |- ( ph -> G : C --> D )
47	46	ffvelrnda 6359	. . . . . . . . . . . . . . 15 |- ( ( ph /\ y e. C ) -> ( G ` y ) e. D )
48	47	adantrl 752	. . . . . . . . . . . . . 14 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( G ` y ) e. D )
49		breq1 4656	. . . . . . . . . . . . . . 15 |- ( z = ( H ` x ) -> ( z S w <-> ( H ` x ) S w ) )
50		breq2 4657	. . . . . . . . . . . . . . 15 |- ( w = ( G ` y ) -> ( ( H ` x ) S w <-> ( H ` x ) S ( G ` y ) ) )
51	49, 50	rspc2v 3322	. . . . . . . . . . . . . 14 |- ( ( ( H ` x ) e. B /\ ( G ` y ) e. D ) -> ( A. z e. B A. w e. D z S w -> ( H ` x ) S ( G ` y ) ) )
52	44, 48, 51	syl2anc 693	. . . . . . . . . . . . 13 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( A. z e. B A. w e. D z S w -> ( H ` x ) S ( G ` y ) ) )
53	40, 52	mpd 15	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( H ` x ) S ( G ` y ) )
54	23	adantrr 753	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( ( H u. G ) ` x ) = ( H ` x ) )
55	16	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ y e. C ) -> H Fn A )
56	19	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ y e. C ) -> G Fn C )
57	7	anim1i 592	. . . . . . . . . . . . . 14 |- ( ( ph /\ y e. C ) -> ( ( A i^i C ) = (/) /\ y e. C ) )
58		fvun2 6270	. . . . . . . . . . . . . 14 |- ( ( H Fn A /\ G Fn C /\ ( ( A i^i C ) = (/) /\ y e. C ) ) -> ( ( H u. G ) ` y ) = ( G ` y ) )
59	55, 56, 57, 58	syl3anc 1326	. . . . . . . . . . . . 13 |- ( ( ph /\ y e. C ) -> ( ( H u. G ) ` y ) = ( G ` y ) )
60	59	adantrl 752	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( ( H u. G ) ` y ) = ( G ` y ) )
61	53, 54, 60	3brtr4d 4685	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) )
62	35, 61	2thd 255	. . . . . . . . . 10 |- ( ( ph /\ ( x e. A /\ y e. C ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
63	62	anassrs 680	. . . . . . . . 9 |- ( ( ( ph /\ x e. A ) /\ y e. C ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
64	33, 63	jaodan 826	. . . . . . . 8 |- ( ( ( ph /\ x e. A ) /\ ( y e. A \/ y e. C ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
65	12, 64	sylan2b 492	. . . . . . 7 |- ( ( ( ph /\ x e. A ) /\ y e. ( A u. C ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
66	65	ex 450	. . . . . 6 |- ( ( ph /\ x e. A ) -> ( y e. ( A u. C ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) ) )
67		isoun.5	. . . . . . . . . . . 12 |- ( ( ph /\ x e. C /\ y e. A ) -> -. x R y )
68	67	3expb 1266	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> -. x R y )
69		isoun.6	. . . . . . . . . . . . . . . . 17 |- ( ( ph /\ z e. D /\ w e. B ) -> -. z S w )
70	69	3expia 1267	. . . . . . . . . . . . . . . 16 |- ( ( ph /\ z e. D ) -> ( w e. B -> -. z S w ) )
71	70	ralrimiv 2965	. . . . . . . . . . . . . . 15 |- ( ( ph /\ z e. D ) -> A. w e. B -. z S w )
72	71	ralrimiva 2966	. . . . . . . . . . . . . 14 |- ( ph -> A. z e. D A. w e. B -. z S w )
73	72	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> A. z e. D A. w e. B -. z S w )
74	46	ffvelrnda 6359	. . . . . . . . . . . . . . 15 |- ( ( ph /\ x e. C ) -> ( G ` x ) e. D )
75	74	adantrr 753	. . . . . . . . . . . . . 14 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> ( G ` x ) e. D )
76	42	ffvelrnda 6359	. . . . . . . . . . . . . . 15 |- ( ( ph /\ y e. A ) -> ( H ` y ) e. B )
77	76	adantrl 752	. . . . . . . . . . . . . 14 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> ( H ` y ) e. B )
78		breq1 4656	. . . . . . . . . . . . . . . 16 |- ( z = ( G ` x ) -> ( z S w <-> ( G ` x ) S w ) )
79	78	notbid 308	. . . . . . . . . . . . . . 15 |- ( z = ( G ` x ) -> ( -. z S w <-> -. ( G ` x ) S w ) )
80		breq2 4657	. . . . . . . . . . . . . . . 16 |- ( w = ( H ` y ) -> ( ( G ` x ) S w <-> ( G ` x ) S ( H ` y ) ) )
81	80	notbid 308	. . . . . . . . . . . . . . 15 |- ( w = ( H ` y ) -> ( -. ( G ` x ) S w <-> -. ( G ` x ) S ( H ` y ) ) )
82	79, 81	rspc2v 3322	. . . . . . . . . . . . . 14 |- ( ( ( G ` x ) e. D /\ ( H ` y ) e. B ) -> ( A. z e. D A. w e. B -. z S w -> -. ( G ` x ) S ( H ` y ) ) )
83	75, 77, 82	syl2anc 693	. . . . . . . . . . . . 13 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> ( A. z e. D A. w e. B -. z S w -> -. ( G ` x ) S ( H ` y ) ) )
84	73, 83	mpd 15	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> -. ( G ` x ) S ( H ` y ) )
85	16	adantr 481	. . . . . . . . . . . . . . 15 |- ( ( ph /\ x e. C ) -> H Fn A )
86	19	adantr 481	. . . . . . . . . . . . . . 15 |- ( ( ph /\ x e. C ) -> G Fn C )
87	7	anim1i 592	. . . . . . . . . . . . . . 15 |- ( ( ph /\ x e. C ) -> ( ( A i^i C ) = (/) /\ x e. C ) )
88		fvun2 6270	. . . . . . . . . . . . . . 15 |- ( ( H Fn A /\ G Fn C /\ ( ( A i^i C ) = (/) /\ x e. C ) ) -> ( ( H u. G ) ` x ) = ( G ` x ) )
89	85, 86, 87, 88	syl3anc 1326	. . . . . . . . . . . . . 14 |- ( ( ph /\ x e. C ) -> ( ( H u. G ) ` x ) = ( G ` x ) )
90	89	adantrr 753	. . . . . . . . . . . . 13 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> ( ( H u. G ) ` x ) = ( G ` x ) )
91	29	adantrl 752	. . . . . . . . . . . . 13 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> ( ( H u. G ) ` y ) = ( H ` y ) )
92	90, 91	breq12d 4666	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> ( ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) <-> ( G ` x ) S ( H ` y ) ) )
93	84, 92	mtbird 315	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> -. ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) )
94	68, 93	2falsed 366	. . . . . . . . . 10 |- ( ( ph /\ ( x e. C /\ y e. A ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
95	94	anassrs 680	. . . . . . . . 9 |- ( ( ( ph /\ x e. C ) /\ y e. A ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
96		isorel 6576	. . . . . . . . . . . 12 |- ( ( G Isom R , S ( C , D ) /\ ( x e. C /\ y e. C ) ) -> ( x R y <-> ( G ` x ) S ( G ` y ) ) )
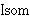
97	4, 96	sylan 488	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. C /\ y e. C ) ) -> ( x R y <-> ( G ` x ) S ( G ` y ) ) )
98	89	adantrr 753	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. C /\ y e. C ) ) -> ( ( H u. G ) ` x ) = ( G ` x ) )
99	59	adantrl 752	. . . . . . . . . . . 12 |- ( ( ph /\ ( x e. C /\ y e. C ) ) -> ( ( H u. G ) ` y ) = ( G ` y ) )
100	98, 99	breq12d 4666	. . . . . . . . . . 11 |- ( ( ph /\ ( x e. C /\ y e. C ) ) -> ( ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) <-> ( G ` x ) S ( G ` y ) ) )
101	97, 100	bitr4d 271	. . . . . . . . . 10 |- ( ( ph /\ ( x e. C /\ y e. C ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
102	101	anassrs 680	. . . . . . . . 9 |- ( ( ( ph /\ x e. C ) /\ y e. C ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
103	95, 102	jaodan 826	. . . . . . . 8 |- ( ( ( ph /\ x e. C ) /\ ( y e. A \/ y e. C ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
104	12, 103	sylan2b 492	. . . . . . 7 |- ( ( ( ph /\ x e. C ) /\ y e. ( A u. C ) ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
105	104	ex 450	. . . . . 6 |- ( ( ph /\ x e. C ) -> ( y e. ( A u. C ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) ) )
106	66, 105	jaodan 826	. . . . 5 |- ( ( ph /\ ( x e. A \/ x e. C ) ) -> ( y e. ( A u. C ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) ) )
107	11, 106	sylan2b 492	. . . 4 |- ( ( ph /\ x e. ( A u. C ) ) -> ( y e. ( A u. C ) -> ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) ) )
108	107	ralrimiv 2965	. . 3 |- ( ( ph /\ x e. ( A u. C ) ) -> A. y e. ( A u. C ) ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
109	108	ralrimiva 2966	. 2 |- ( ph -> A. x e. ( A u. C ) A. y e. ( A u. C ) ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) )
110		df-isom 5897	. 2 |- ( ( H u. G ) Isom R , S ( ( A u. C ) , ( B u. D ) ) <-> ( ( H u. G ) : ( A u. C ) -1-1-onto-> ( B u. D ) /\ A. x e. ( A u. C ) A. y e. ( A u. C ) ( x R y <-> ( ( H u. G ) ` x ) S ( ( H u. G ) ` y ) ) ) )
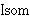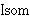
111	10, 109, 110	sylanbrc 698	1 |- ( ph -> ( H u. G ) Isom R , S ( ( A u. C ) , ( B u. D ) ) )

Syntax hints:   -. wn 3   -> wi 4   <-> wb 196   \/ wo 383   /\ wa 384   /\ w3a 1037   = wceq 1483   e. wcel 1990   A.wral 2912   u. cun 3572   i^i cin 3573   (/)c0 3915   class class class wbr 4653   Fn wfn 5883   -->wf 5884   -1-1-onto->wf1o 5887   ` cfv 5888   Isom wiso 5889

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-ral 2917  df-rex 2918  df-rab 2921  df-v 3202  df-sbc 3436  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-nul 3916  df-if 4087  df-sn 4178  df-pr 4180  df-op 4184  df-uni 4437  df-br 4654  df-opab 4713  df-id 5024  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-isom 5897

This theorem is referenced by: (None)