Theorem isoini 5477

Description: Isomorphisms preserve initial segments. Proposition 6.31(2) of [TakeutiZaring] p. 33. (Contributed by NM, 20-Apr-2004.)

Assertion

Ref	Expression
isoini	|- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( H " ( A i^i ( `' R " { D } ) ) ) = ( B i^i ( `' S " { ( H ` D ) } ) ) )

Proof of Theorem isoini

Dummy variables x y are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		elin 3155	. . . 4 |- ( y e. ( B i^i ( `' S " { ( H ` D ) } ) ) <-> ( y e. B /\ y e. ( `' S " { ( H ` D ) } ) ) )
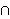
2		isof1o 5467	. . . . . . . . 9 |- ( H Isom R , S ( A , B ) -> H : A -1-1-onto-> B )
3		f1ofo 5153	. . . . . . . . 9 |- ( H : A -1-1-onto-> B -> H : A -onto-> B )
4		forn 5129	. . . . . . . . . 10 |- ( H : A -onto-> B -> ran H = B )
5	4	eleq2d 2148	. . . . . . . . 9 |- ( H : A -onto-> B -> ( y e. ran H <-> y e. B ) )
6	2, 3, 5	3syl 17	. . . . . . . 8 |- ( H Isom R , S ( A , B ) -> ( y e. ran H <-> y e. B ) )
7		f1ofn 5147	. . . . . . . . 9 |- ( H : A -1-1-onto-> B -> H Fn A )
8		fvelrnb 5242	. . . . . . . . 9 |- ( H Fn A -> ( y e. ran H <-> E. x e. A ( H ` x ) = y ) )
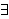9	2, 7, 8	3syl 17	. . . . . . . 8 |- ( H Isom R , S ( A , B ) -> ( y e. ran H <-> E. x e. A ( H ` x ) = y ) )
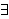10	6, 9	bitr3d 188	. . . . . . 7 |- ( H Isom R , S ( A , B ) -> ( y e. B <-> E. x e. A ( H ` x ) = y ) )
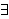11	10	adantr 270	. . . . . 6 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( y e. B <-> E. x e. A ( H ` x ) = y ) )
12	2, 7	syl 14	. . . . . . . 8 |- ( H Isom R , S ( A , B ) -> H Fn A )
13	12	anim1i 333	. . . . . . 7 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( H Fn A /\ D e. A ) )
14		funfvex 5212	. . . . . . . 8 |- ( ( Fun H /\ D e. dom H ) -> ( H ` D ) e. _V )
15	14	funfni 5019	. . . . . . 7 |- ( ( H Fn A /\ D e. A ) -> ( H ` D ) e. _V )
16		vex 2604	. . . . . . . 8 |- y e. _V
17	16	eliniseg 4715	. . . . . . 7 |- ( ( H ` D ) e. _V -> ( y e. ( `' S " { ( H ` D ) } ) <-> y S ( H ` D ) ) )
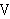
18	13, 15, 17	3syl 17	. . . . . 6 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( y e. ( `' S " { ( H ` D ) } ) <-> y S ( H ` D ) ) )
19	11, 18	anbi12d 456	. . . . 5 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( ( y e. B /\ y e. ( `' S " { ( H ` D ) } ) ) <-> ( E. x e. A ( H ` x ) = y /\ y S ( H ` D ) ) ) )
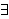
20		elin 3155	. . . . . . . . . . . 12 |- ( x e. ( A i^i ( `' R " { D } ) ) <-> ( x e. A /\ x e. ( `' R " { D } ) ) )
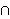
21		vex 2604	. . . . . . . . . . . . . 14 |- x e. _V
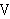22	21	eliniseg 4715	. . . . . . . . . . . . 13 |- ( D e. A -> ( x e. ( `' R " { D } ) <-> x R D ) )
23	22	anbi2d 451	. . . . . . . . . . . 12 |- ( D e. A -> ( ( x e. A /\ x e. ( `' R " { D } ) ) <-> ( x e. A /\ x R D ) ) )
24	20, 23	syl5bb 190	. . . . . . . . . . 11 |- ( D e. A -> ( x e. ( A i^i ( `' R " { D } ) ) <-> ( x e. A /\ x R D ) ) )
25	24	anbi1d 452	. . . . . . . . . 10 |- ( D e. A -> ( ( x e. ( A i^i ( `' R " { D } ) ) /\ x H y ) <-> ( ( x e. A /\ x R D ) /\ x H y ) ) )
26		anass 393	. . . . . . . . . 10 |- ( ( ( x e. A /\ x R D ) /\ x H y ) <-> ( x e. A /\ ( x R D /\ x H y ) ) )
27	25, 26	syl6bb 194	. . . . . . . . 9 |- ( D e. A -> ( ( x e. ( A i^i ( `' R " { D } ) ) /\ x H y ) <-> ( x e. A /\ ( x R D /\ x H y ) ) ) )
28	27	adantl 271	. . . . . . . 8 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( ( x e. ( A i^i ( `' R " { D } ) ) /\ x H y ) <-> ( x e. A /\ ( x R D /\ x H y ) ) ) )
29		isorel 5468	. . . . . . . . . . . . . 14 |- ( ( H Isom R , S ( A , B ) /\ ( x e. A /\ D e. A ) ) -> ( x R D <-> ( H ` x ) S ( H ` D ) ) )
30		fnbrfvb 5235	. . . . . . . . . . . . . . . . 17 |- ( ( H Fn A /\ x e. A ) -> ( ( H ` x ) = y <-> x H y ) )
31	30	bicomd 139	. . . . . . . . . . . . . . . 16 |- ( ( H Fn A /\ x e. A ) -> ( x H y <-> ( H ` x ) = y ) )
32	12, 31	sylan 277	. . . . . . . . . . . . . . 15 |- ( ( H Isom R , S ( A , B ) /\ x e. A ) -> ( x H y <-> ( H ` x ) = y ) )
33	32	adantrr 462	. . . . . . . . . . . . . 14 |- ( ( H Isom R , S ( A , B ) /\ ( x e. A /\ D e. A ) ) -> ( x H y <-> ( H ` x ) = y ) )
34	29, 33	anbi12d 456	. . . . . . . . . . . . 13 |- ( ( H Isom R , S ( A , B ) /\ ( x e. A /\ D e. A ) ) -> ( ( x R D /\ x H y ) <-> ( ( H ` x ) S ( H ` D ) /\ ( H ` x ) = y ) ) )
35		ancom 262	. . . . . . . . . . . . . 14 |- ( ( ( H ` x ) S ( H ` D ) /\ ( H ` x ) = y ) <-> ( ( H ` x ) = y /\ ( H ` x ) S ( H ` D ) ) )
36		breq1 3788	. . . . . . . . . . . . . . 15 |- ( ( H ` x ) = y -> ( ( H ` x ) S ( H ` D ) <-> y S ( H ` D ) ) )
37	36	pm5.32i 441	. . . . . . . . . . . . . 14 |- ( ( ( H ` x ) = y /\ ( H ` x ) S ( H ` D ) ) <-> ( ( H ` x ) = y /\ y S ( H ` D ) ) )
38	35, 37	bitri 182	. . . . . . . . . . . . 13 |- ( ( ( H ` x ) S ( H ` D ) /\ ( H ` x ) = y ) <-> ( ( H ` x ) = y /\ y S ( H ` D ) ) )
39	34, 38	syl6bb 194	. . . . . . . . . . . 12 |- ( ( H Isom R , S ( A , B ) /\ ( x e. A /\ D e. A ) ) -> ( ( x R D /\ x H y ) <-> ( ( H ` x ) = y /\ y S ( H ` D ) ) ) )
40	39	exp32 357	. . . . . . . . . . 11 |- ( H Isom R , S ( A , B ) -> ( x e. A -> ( D e. A -> ( ( x R D /\ x H y ) <-> ( ( H ` x ) = y /\ y S ( H ` D ) ) ) ) ) )
41	40	com23 77	. . . . . . . . . 10 |- ( H Isom R , S ( A , B ) -> ( D e. A -> ( x e. A -> ( ( x R D /\ x H y ) <-> ( ( H ` x ) = y /\ y S ( H ` D ) ) ) ) ) )
42	41	imp 122	. . . . . . . . 9 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( x e. A -> ( ( x R D /\ x H y ) <-> ( ( H ` x ) = y /\ y S ( H ` D ) ) ) ) )
43	42	pm5.32d 437	. . . . . . . 8 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( ( x e. A /\ ( x R D /\ x H y ) ) <-> ( x e. A /\ ( ( H ` x ) = y /\ y S ( H ` D ) ) ) ) )
44	28, 43	bitrd 186	. . . . . . 7 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( ( x e. ( A i^i ( `' R " { D } ) ) /\ x H y ) <-> ( x e. A /\ ( ( H ` x ) = y /\ y S ( H ` D ) ) ) ) )
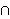
45	44	rexbidv2 2371	. . . . . 6 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( E. x e. ( A i^i ( `' R " { D } ) ) x H y <-> E. x e. A ( ( H ` x ) = y /\ y S ( H ` D ) ) ) )
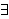
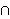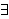
46		r19.41v 2510	. . . . . 6 |- ( E. x e. A ( ( H ` x ) = y /\ y S ( H ` D ) ) <-> ( E. x e. A ( H ` x ) = y /\ y S ( H ` D ) ) )
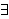
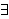
47	45, 46	syl6bb 194	. . . . 5 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( E. x e. ( A i^i ( `' R " { D } ) ) x H y <-> ( E. x e. A ( H ` x ) = y /\ y S ( H ` D ) ) ) )
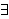
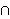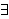48	19, 47	bitr4d 189	. . . 4 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( ( y e. B /\ y e. ( `' S " { ( H ` D ) } ) ) <-> E. x e. ( A i^i ( `' R " { D } ) ) x H y ) )
49	1, 48	syl5bb 190	. . 3 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( y e. ( B i^i ( `' S " { ( H ` D ) } ) ) <-> E. x e. ( A i^i ( `' R " { D } ) ) x H y ) )
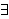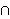50	49	abbi2dv 2197	. 2 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( B i^i ( `' S " { ( H ` D ) } ) ) = { y | E. x e. ( A i^i ( `' R " { D } ) ) x H y } )
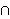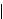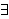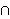51		dfima2 4690	. 2 |- ( H " ( A i^i ( `' R " { D } ) ) ) = { y | E. x e. ( A i^i ( `' R " { D } ) ) x H y }
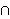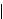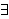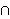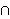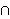52	50, 51	syl6reqr 2132	1 |- ( ( H Isom R , S ( A , B ) /\ D e. A ) -> ( H " ( A i^i ( `' R " { D } ) ) ) = ( B i^i ( `' S " { ( H ` D ) } ) ) )

Syntax hints:   -> wi 4   /\ wa 102   <-> wb 103   = wceq 1284   e. wcel 1433   {cab 2067   E.wrex 2349   _Vcvv 2601   i^i cin 2972   {csn 3398   class class class wbr 3785   `'ccnv 4362   ran crn 4364   "cima 4366   Fn wfn 4917   -onto->wfo 4920   -1-1-onto->wf1o 4921   ` cfv 4922   Isom wiso 4923

This theorem was proved from axioms:  ax-1 5  ax-2 6  ax-mp 7  ax-ia1 104  ax-ia2 105  ax-ia3 106  ax-io 662  ax-5 1376  ax-7 1377  ax-gen 1378  ax-ie1 1422  ax-ie2 1423  ax-8 1435  ax-10 1436  ax-11 1437  ax-i12 1438  ax-bndl 1439  ax-4 1440  ax-14 1445  ax-17 1459  ax-i9 1463  ax-ial 1467  ax-i5r 1468  ax-ext 2063  ax-sep 3896  ax-pow 3948  ax-pr 3964

This theorem depends on definitions:  df-bi 115  df-3an 921  df-tru 1287  df-nf 1390  df-sb 1686  df-eu 1944  df-mo 1945  df-clab 2068  df-cleq 2074  df-clel 2077  df-nfc 2208  df-ral 2353  df-rex 2354  df-v 2603  df-sbc 2816  df-un 2977  df-in 2979  df-ss 2986  df-pw 3384  df-sn 3404  df-pr 3405  df-op 3407  df-uni 3602  df-br 3786  df-opab 3840  df-mpt 3841  df-id 4048  df-xp 4369  df-rel 4370  df-cnv 4371  df-co 4372  df-dm 4373  df-rn 4374  df-res 4375  df-ima 4376  df-iota 4887  df-fun 4924  df-fn 4925  df-f 4926  df-f1 4927  df-fo 4928  df-f1o 4929  df-fv 4930  df-isom 4931

This theorem is referenced by:  isoini2  5478  isoselem  5479