Theorem crngohomfo 33805

Description: The image of a homomorphism from a commutative ring is commutative. (Contributed by Jeff Madsen, 4-Jan-2011.)

Hypotheses

Ref	Expression
crnghomfo.1	|- G = ( 1st ` R )
crnghomfo.2	|- X = ran G
crnghomfo.3	|- J = ( 1st ` S )
crnghomfo.4	|- Y = ran J

Assertion

Ref	Expression
crngohomfo	|- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> S e. CRingOps )

Proof of Theorem crngohomfo

Dummy variables w x y z are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		simplr 792	. 2 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> S e. RingOps )
2		foelrn 6378	. . . . . . . 8 |- ( ( F : X -onto-> Y /\ y e. Y ) -> E. w e. X y = ( F ` w ) )
3	2	ex 450	. . . . . . 7 |- ( F : X -onto-> Y -> ( y e. Y -> E. w e. X y = ( F ` w ) ) )
4		foelrn 6378	. . . . . . . 8 |- ( ( F : X -onto-> Y /\ z e. Y ) -> E. x e. X z = ( F ` x ) )
5	4	ex 450	. . . . . . 7 |- ( F : X -onto-> Y -> ( z e. Y -> E. x e. X z = ( F ` x ) ) )
6	3, 5	anim12d 586	. . . . . 6 |- ( F : X -onto-> Y -> ( ( y e. Y /\ z e. Y ) -> ( E. w e. X y = ( F ` w ) /\ E. x e. X z = ( F ` x ) ) ) )
7		reeanv 3107	. . . . . 6 |- ( E. w e. X E. x e. X ( y = ( F ` w ) /\ z = ( F ` x ) ) <-> ( E. w e. X y = ( F ` w ) /\ E. x e. X z = ( F ` x ) ) )
8	6, 7	syl6ibr 242	. . . . 5 |- ( F : X -onto-> Y -> ( ( y e. Y /\ z e. Y ) -> E. w e. X E. x e. X ( y = ( F ` w ) /\ z = ( F ` x ) ) ) )
9	8	ad2antll 765	. . . 4 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> ( ( y e. Y /\ z e. Y ) -> E. w e. X E. x e. X ( y = ( F ` w ) /\ z = ( F ` x ) ) ) )
10		crnghomfo.1	. . . . . . . . . . . . . 14 |- G = ( 1st ` R )
11		eqid 2622	. . . . . . . . . . . . . 14 |- ( 2nd ` R ) = ( 2nd ` R )
12		crnghomfo.2	. . . . . . . . . . . . . 14 |- X = ran G
13	10, 11, 12	crngocom 33800	. . . . . . . . . . . . 13 |- ( ( R e. CRingOps /\ w e. X /\ x e. X ) -> ( w ( 2nd ` R ) x ) = ( x ( 2nd ` R ) w ) )
14	13	3expb 1266	. . . . . . . . . . . 12 |- ( ( R e. CRingOps /\ ( w e. X /\ x e. X ) ) -> ( w ( 2nd ` R ) x ) = ( x ( 2nd ` R ) w ) )
15	14	3ad2antl1 1223	. . . . . . . . . . 11 |- ( ( ( R e. CRingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( w ( 2nd ` R ) x ) = ( x ( 2nd ` R ) w ) )
16	15	fveq2d 6195	. . . . . . . . . 10 |- ( ( ( R e. CRingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( F ` ( w ( 2nd ` R ) x ) ) = ( F ` ( x ( 2nd ` R ) w ) ) )
17		crngorngo 33799	. . . . . . . . . . 11 |- ( R e. CRingOps -> R e. RingOps )
18		eqid 2622	. . . . . . . . . . . 12 |- ( 2nd ` S ) = ( 2nd ` S )
19	10, 12, 11, 18	rngohommul 33769	. . . . . . . . . . 11 |- ( ( ( R e. RingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( F ` ( w ( 2nd ` R ) x ) ) = ( ( F ` w ) ( 2nd ` S ) ( F ` x ) ) )
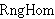20	17, 19	syl3anl1 1374	. . . . . . . . . 10 |- ( ( ( R e. CRingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( F ` ( w ( 2nd ` R ) x ) ) = ( ( F ` w ) ( 2nd ` S ) ( F ` x ) ) )
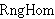21	10, 12, 11, 18	rngohommul 33769	. . . . . . . . . . . 12 |- ( ( ( R e. RingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( x e. X /\ w e. X ) ) -> ( F ` ( x ( 2nd ` R ) w ) ) = ( ( F ` x ) ( 2nd ` S ) ( F ` w ) ) )
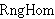22	21	ancom2s 844	. . . . . . . . . . 11 |- ( ( ( R e. RingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( F ` ( x ( 2nd ` R ) w ) ) = ( ( F ` x ) ( 2nd ` S ) ( F ` w ) ) )
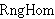23	17, 22	syl3anl1 1374	. . . . . . . . . 10 |- ( ( ( R e. CRingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( F ` ( x ( 2nd ` R ) w ) ) = ( ( F ` x ) ( 2nd ` S ) ( F ` w ) ) )
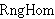24	16, 20, 23	3eqtr3d 2664	. . . . . . . . 9 |- ( ( ( R e. CRingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( ( F ` w ) ( 2nd ` S ) ( F ` x ) ) = ( ( F ` x ) ( 2nd ` S ) ( F ` w ) ) )
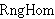25		oveq12 6659	. . . . . . . . . 10 |- ( ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( y ( 2nd ` S ) z ) = ( ( F ` w ) ( 2nd ` S ) ( F ` x ) ) )
26		oveq12 6659	. . . . . . . . . . 11 |- ( ( z = ( F ` x ) /\ y = ( F ` w ) ) -> ( z ( 2nd ` S ) y ) = ( ( F ` x ) ( 2nd ` S ) ( F ` w ) ) )
27	26	ancoms 469	. . . . . . . . . 10 |- ( ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( z ( 2nd ` S ) y ) = ( ( F ` x ) ( 2nd ` S ) ( F ` w ) ) )
28	25, 27	eqeq12d 2637	. . . . . . . . 9 |- ( ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) <-> ( ( F ` w ) ( 2nd ` S ) ( F ` x ) ) = ( ( F ` x ) ( 2nd ` S ) ( F ` w ) ) ) )
29	24, 28	syl5ibrcom 237	. . . . . . . 8 |- ( ( ( R e. CRingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) /\ ( w e. X /\ x e. X ) ) -> ( ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) ) )
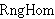
30	29	ex 450	. . . . . . 7 |- ( ( R e. CRingOps /\ S e. RingOps /\ F e. ( R RngHom S ) ) -> ( ( w e. X /\ x e. X ) -> ( ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) ) ) )
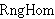
31	30	3expa 1265	. . . . . 6 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ F e. ( R RngHom S ) ) -> ( ( w e. X /\ x e. X ) -> ( ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) ) ) )
32	31	adantrr 753	. . . . 5 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> ( ( w e. X /\ x e. X ) -> ( ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) ) ) )
33	32	rexlimdvv 3037	. . . 4 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> ( E. w e. X E. x e. X ( y = ( F ` w ) /\ z = ( F ` x ) ) -> ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) ) )
34	9, 33	syld 47	. . 3 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> ( ( y e. Y /\ z e. Y ) -> ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) ) )
35	34	ralrimivv 2970	. 2 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> A. y e. Y A. z e. Y ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) )
36		crnghomfo.3	. . 3 |- J = ( 1st ` S )
37		crnghomfo.4	. . 3 |- Y = ran J
38	36, 18, 37	iscrngo2 33796	. 2 |- ( S e. CRingOps <-> ( S e. RingOps /\ A. y e. Y A. z e. Y ( y ( 2nd ` S ) z ) = ( z ( 2nd ` S ) y ) ) )
39	1, 35, 38	sylanbrc 698	1 |- ( ( ( R e. CRingOps /\ S e. RingOps ) /\ ( F e. ( R RngHom S ) /\ F : X -onto-> Y ) ) -> S e. CRingOps )

Syntax hints:   -> wi 4   /\ wa 384   /\ w3a 1037   = wceq 1483   e. wcel 1990   A.wral 2912   E.wrex 2913   ran crn 5115   -onto->wfo 5886   ` cfv 5888  (class class class)co 6650   1stc1st 7166   2ndc2nd 7167   RingOpscrngo 33693   RngHom crnghom 33759  CRingOpsccring 33792

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ral 2917  df-rex 2918  df-rab 2921  df-v 3202  df-sbc 3436  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-op 4184  df-uni 4437  df-br 4654  df-opab 4713  df-mpt 4730  df-id 5024  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-fo 5894  df-fv 5896  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-1st 7168  df-2nd 7169  df-map 7859  df-rngo 33694  df-rngohom 33762  df-com2 33789  df-crngo 33793

This theorem is referenced by: (None)