Proof of Theorem suppeqfsuppbi
| Step | Hyp | Ref
| Expression |
|---|
| 1 | | simprlr 803 |
. . . . . 6
                
   
          |
| 2 | | simprll 802 |
. . . . . 6
                
   
        
  |
| 3 | | simpl 473 |
. . . . . 6
                
   
        
  |
| 4 | | funisfsupp 8280 |
. . . . . 6
        
        finSupp finSupp     supp supp       |
| 5 | 1, 2, 3, 4 | syl3anc 1326 |
. . . . 5
                
   
         finSupp finSupp
 
  supp supp       |
| 6 | 5 | adantr 481 |
. . . 4
                 
   
         supp
supp      supp supp       finSupp finSupp     supp supp       |
| 7 | | simpr 477 |
. . . . . . . . . 10
              |
| 8 | 7 | adantr 481 |
. . . . . . . . 9
             
      |
| 9 | | simpl 473 |
. . . . . . . . . 10
               |
| 10 | 9 | adantr 481 |
. . . . . . . . 9
             
       |
| 11 | | simpr 477 |
. . . . . . . . 9
             
       |
| 12 | | funisfsupp 8280 |
. . . . . . . . 9
        
        finSupp finSupp     supp supp       |
| 13 | 8, 10, 11, 12 | syl3anc 1326 |
. . . . . . . 8
             
     finSupp finSupp
 
  supp supp       |
| 14 | 13 | ex 450 |
. . . . . . 7
             
    finSupp finSupp 
   supp supp        |
| 15 | 14 | adantl 482 |
. . . . . 6
             
               finSupp finSupp     supp supp        |
| 16 | 15 | impcom 446 |
. . . . 5
                
   
         finSupp finSupp
 
  supp supp       |
| 17 | | eleq1 2689 |
. . . . . 6
    supp supp      supp supp       supp supp        supp supp       |
| 18 | 17 | bicomd 213 |
. . . . 5
    supp supp      supp supp       supp supp        supp supp       |
| 19 | 16, 18 | sylan9bb 736 |
. . . 4
                 
   
         supp
supp      supp supp       finSupp finSupp     supp supp       |
| 20 | 6, 19 | bitr4d 271 |
. . 3
                 
   
         supp
supp      supp supp       finSupp finSupp    finSupp
finSupp    |
| 21 | 20 | exp31 630 |
. 2
                
   
         supp supp
     supp supp   
  finSupp finSupp    finSupp finSupp      |
| 22 | | relfsupp 8277 |
. . . . 5
 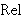 finSupp finSupp |
| 23 | 22 | brrelex2i 5159 |
. . . 4
   finSupp finSupp       |
| 24 | 22 | brrelex2i 5159 |
. . . 4
   finSupp finSupp       |
| 25 | 23, 24 | pm5.21ni 367 |
. . 3
         finSupp finSupp 
  finSupp
finSupp    |
| 26 | 25 | 2a1d 26 |
. 2
                 
   
         supp supp
     supp supp   
  finSupp finSupp    finSupp finSupp      |
| 27 | 21, 26 | pm2.61i 176 |
1
             
           supp supp      supp supp      finSupp finSupp
 
 finSupp finSupp     |