| Step | Hyp | Ref
| Expression |
|---|
| 1 | | elex 3212 |
. . . . 5
              SubGrp SubGrp                          
                
        
                     |
| 2 | 1 | a1i 11 |
. . . 4
                          SubGrp SubGrp                          
                
        
                      |
| 3 | | fex 6490 |
. . . . . . 7
        SubGrp SubGrp             |
| 4 | 3 | expcom 451 |
. . . . . 6
            SubGrp SubGrp         |
| 5 | 4 | adantr 481 |
. . . . 5
                   SubGrp SubGrp         |
| 6 | 5 | adantrd 484 |
. . . 4
                    SubGrp SubGrp      
   
   
                                                        |
| 7 | | df-sbc 3436 |
. . . . . 6
      ![]. ].](_drbrack.gif)        SubGrp SubGrp                          
                
        
               
            SubGrp SubGrp      
                                    
        
                 |
| 8 | | simpr 477 |
. . . . . . 7
                       |
| 9 | | simpr 477 |
. . . . . . . . . 10
                       |
| 10 | 9 | dmeqd 5326 |
. . . . . . . . . . 11
                     
   |
| 11 | | simplr 792 |
. . . . . . . . . . 11
                        |
| 12 | 10, 11 | eqtrd 2656 |
. . . . . . . . . 10
                     
  |
| 13 | 9, 12 | feq12d 6033 |
. . . . . . . . 9
                          SubGrp SubGrp   
     SubGrp SubGrp     |
| 14 | 12 | difeq1d 3727 |
. . . . . . . . . . . 12
                                    |
| 15 | 9 | fveq1d 6193 |
. . . . . . . . . . . . 13
                               |
| 16 | 9 | fveq1d 6193 |
. . . . . . . . . . . . . 14
                               |
| 17 | 16 | fveq2d 6195 |
. . . . . . . . . . . . 13
                                       |
| 18 | 15, 17 | sseq12d 3634 |
. . . . . . . . . . . 12
                         
         
                 |
| 19 | 14, 18 | raleqbidv 3152 |
. . . . . . . . . . 11
                     
                          
   
                     |
| 20 | 9, 14 | imaeq12d 5467 |
. . . . . . . . . . . . . . 15
                                     
      |
| 21 | 20 | unieqd 4446 |
. . . . . . . . . . . . . 14
                                              |
| 22 | 21 | fveq2d 6195 |
. . . . . . . . . . . . 13
                                                      |
| 23 | 15, 22 | ineq12d 3815 |
. . . . . . . . . . . 12
                         
        
                
                  |
| 24 | 23 | eqeq1d 2624 |
. . . . . . . . . . 11
                                                 
                              |
| 25 | 19, 24 | anbi12d 747 |
. . . . . . . . . 10
                                     
                
        
              
   
            
                
                        |
| 26 | 12, 25 | raleqbidv 3152 |
. . . . . . . . 9
                     
                    
                
        
              
   
   
            
                
                        |
| 27 | 13, 26 | anbi12d 747 |
. . . . . . . 8
                           SubGrp SubGrp                          
                
        
               
      SubGrp SubGrp      
   
   
                                                    |
| 28 | 27 | adantlr 751 |
. . . . . . 7
                                 SubGrp SubGrp                          
                
        
               
      SubGrp SubGrp      
   
   
                                                    |
| 29 | 8, 28 | sbcied 3472 |
. . . . . 6
                     
  ![]. ].](_drbrack.gif)        SubGrp SubGrp                          
                
        
               
      SubGrp SubGrp      
   
   
                                                    |
| 30 | 7, 29 | syl5bbr 274 |
. . . . 5
                     
          SubGrp SubGrp      
                                    
        
                       SubGrp SubGrp                                                   
               |
| 31 | 30 | ex 450 |
. . . 4
                               SubGrp SubGrp                          
                
        
                       SubGrp SubGrp                                                   
                |
| 32 | 2, 6, 31 | pm5.21ndd 369 |
. . 3
                          SubGrp SubGrp                          
                
        
                       SubGrp SubGrp                                                   
               |
| 33 | 32 | anbi2d 740 |
. 2
                               SubGrp SubGrp                          
                
        
                      
      SubGrp SubGrp      
   
   
                                                     |
| 34 | | df-br 4654 |
. . 3
    DProd DProd 
        DProd
DProd  |
| 35 | | fvex 6201 |
. . . . . . . . . . 11
        |
| 36 | 35 | rgenw 2924 |
. . . . . . . . . 10
             |
| 37 | | ixpexg 7932 |
. . . . . . . . . 10
    
          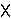             |
| 38 | 36, 37 | ax-mp 5 |
. . . . . . . . 9
 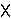            |
| 39 | 38 | mptrabex 6488 |
. . . . . . . 8
       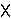 
          finSupp finSupp           g g      |
| 40 | 39 | rnex 7100 |
. . . . . . 7
        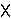            finSupp finSupp           g g      |
| 41 | 40 | rgen2w 2925 |
. . . . . 6
                  SubGrp SubGrp                          
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                      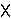  
         finSupp finSupp
          g g      |
| 42 | | df-dprd 18394 |
. . . . . . 7
 DProd DProd                   SubGrp SubGrp      
                      Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                       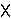  
         finSupp finSupp
          g g     |
| 43 | 42 | fmpt2x 7236 |
. . . . . 6
    
              SubGrp SubGrp                          
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                      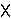  
         finSupp finSupp
          g g       DProd DProd            
        SubGrp SubGrp                          
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                   |
| 44 | 41, 43 | mpbi 220 |
. . . . 5
 DProd DProd    
                SubGrp SubGrp      
                      Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                  |
| 45 | 44 | fdmi 6052 |
. . . 4
  DProd DProd    
                SubGrp SubGrp      
                      Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                |
| 46 | 45 | eleq2i 2693 |
. . 3
    
    DProd DProd                  
        SubGrp SubGrp                          
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                 |
| 47 | | fveq2 6191 |
. . . . . . 7
       SubGrp SubGrp     SubGrp SubGrp    |
| 48 | 47 | feq3d 6032 |
. . . . . 6
             SubGrp SubGrp   
      SubGrp SubGrp     |
| 49 | | fveq2 6191 |
. . . . . . . . . . . 12
       Cntz Cntz     Cntz Cntz    |
| 50 | | dmdprd.z |
. . . . . . . . . . . 12
    Cntz Cntz   |
| 51 | 49, 50 | syl6eqr 2674 |
. . . . . . . . . . 11
       Cntz Cntz      |
| 52 | 51 | fveq1d 6193 |
. . . . . . . . . 10
        Cntz Cntz                     |
| 53 | 52 | sseq2d 3633 |
. . . . . . . . 9
            
  Cntz Cntz                            |
| 54 | 53 | ralbidv 2986 |
. . . . . . . 8
        
                 Cntz Cntz          
                
           |
| 55 | 47 | fveq2d 6195 |
. . . . . . . . . . . 12
       mrCls mrCls  SubGrp SubGrp      mrCls mrCls  SubGrp SubGrp     |
| 56 | | dmdprd.k |
. . . . . . . . . . . 12
    mrCls mrCls  SubGrp SubGrp    |
| 57 | 55, 56 | syl6eqr 2674 |
. . . . . . . . . . 11
       mrCls mrCls  SubGrp SubGrp       |
| 58 | 57 | fveq1d 6193 |
. . . . . . . . . 10
        mrCls mrCls  SubGrp SubGrp                                      |
| 59 | 58 | ineq2d 3814 |
. . . . . . . . 9
            
  mrCls mrCls  SubGrp SubGrp                                               |
| 60 | | fveq2 6191 |
. . . . . . . . . . 11
                  |
| 61 | | dmdprd.0 |
. . . . . . . . . . 11
        |
| 62 | 60, 61 | syl6eqr 2674 |
. . . . . . . . . 10
              |
| 63 | 62 | sneqd 4189 |
. . . . . . . . 9
                  |
| 64 | 59, 63 | eqeq12d 2637 |
. . . . . . . 8
                mrCls mrCls  SubGrp SubGrp                                                            |
| 65 | 54, 64 | anbi12d 747 |
. . . . . . 7
                        
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                             
   
                                                        |
| 66 | 65 | ralbidv 2986 |
. . . . . 6
        
                    
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                             
        
                                                        |
| 67 | 48, 66 | anbi12d 747 |
. . . . 5
              SubGrp SubGrp                          
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                              
       SubGrp SubGrp      
                                    
        
                 |
| 68 | 67 | abbidv 2741 |
. . . 4
                SubGrp SubGrp      
                      Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                          SubGrp SubGrp                          
                
        
                 |
| 69 | 68 | opeliunxp2 5260 |
. . 3
    
             
        SubGrp SubGrp                          
  Cntz Cntz          
         mrCls mrCls  SubGrp SubGrp                                     
            SubGrp SubGrp      
                                    
        
                  |
| 70 | 34, 46, 69 | 3bitri 286 |
. 2
    DProd DProd 
     
            SubGrp SubGrp      
                                    
        
                  |
| 71 | | 3anass 1042 |
. 2
            SubGrp SubGrp      
   
   
                                                  
           SubGrp SubGrp      
   
   
                                                    |
| 72 | 33, 70, 71 | 3bitr4g 303 |
1
                DProd DProd             SubGrp SubGrp      
   
   
                                                    |