Theorem ssenen 8134

Description: Equinumerosity of equinumerous subsets of a set. (Contributed by NM, 30-Sep-2004.) (Revised by Mario Carneiro, 16-Nov-2014.)

Assertion

Ref	Expression
ssenen	|- ( A ~~ B -> { x | ( x C_ A /\ x ~~ C ) } ~~ { x | ( x C_ B /\ x ~~ C ) } )

Distinct variable groups:   x, A   x, B   x, C

Proof of Theorem ssenen

Dummy variables y z f are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		bren 7964	. . 3 |- ( A ~~ B <-> E. f f : A -1-1-onto-> B )
2		f1odm 6141	. . . . . . 7 |- ( f : A -1-1-onto-> B -> dom f = A )
3		vex 3203	. . . . . . . 8 |- f e. _V
4	3	dmex 7099	. . . . . . 7 |- dom f e. _V
5	2, 4	syl6eqelr 2710	. . . . . 6 |- ( f : A -1-1-onto-> B -> A e. _V )
6		pwexg 4850	. . . . . 6 |- ( A e. _V -> ~P A e. _V )
7		inex1g 4801	. . . . . 6 |- ( ~P A e. _V -> ( ~P A i^i { x | x ~~ C } ) e. _V )
8	5, 6, 7	3syl 18	. . . . 5 |- ( f : A -1-1-onto-> B -> ( ~P A i^i { x | x ~~ C } ) e. _V )
9		f1ofo 6144	. . . . . . . 8 |- ( f : A -1-1-onto-> B -> f : A -onto-> B )
10		forn 6118	. . . . . . . 8 |- ( f : A -onto-> B -> ran f = B )
11	9, 10	syl 17	. . . . . . 7 |- ( f : A -1-1-onto-> B -> ran f = B )
12	3	rnex 7100	. . . . . . 7 |- ran f e. _V
13	11, 12	syl6eqelr 2710	. . . . . 6 |- ( f : A -1-1-onto-> B -> B e. _V )
14		pwexg 4850	. . . . . 6 |- ( B e. _V -> ~P B e. _V )
15		inex1g 4801	. . . . . 6 |- ( ~P B e. _V -> ( ~P B i^i { x | x ~~ C } ) e. _V )
16	13, 14, 15	3syl 18	. . . . 5 |- ( f : A -1-1-onto-> B -> ( ~P B i^i { x | x ~~ C } ) e. _V )
17		f1of1 6136	. . . . . . . . . . 11 |- ( f : A -1-1-onto-> B -> f : A -1-1-> B )
18	17	adantr 481	. . . . . . . . . 10 |- ( ( f : A -1-1-onto-> B /\ y C_ A ) -> f : A -1-1-> B )
19	13	adantr 481	. . . . . . . . . 10 |- ( ( f : A -1-1-onto-> B /\ y C_ A ) -> B e. _V )
20		simpr 477	. . . . . . . . . 10 |- ( ( f : A -1-1-onto-> B /\ y C_ A ) -> y C_ A )
21		vex 3203	. . . . . . . . . . 11 |- y e. _V
22	21	a1i 11	. . . . . . . . . 10 |- ( ( f : A -1-1-onto-> B /\ y C_ A ) -> y e. _V )
23		f1imaen2g 8017	. . . . . . . . . 10 |- ( ( ( f : A -1-1-> B /\ B e. _V ) /\ ( y C_ A /\ y e. _V ) ) -> ( f " y ) ~~ y )
24	18, 19, 20, 22, 23	syl22anc 1327	. . . . . . . . 9 |- ( ( f : A -1-1-onto-> B /\ y C_ A ) -> ( f " y ) ~~ y )
25		entr 8008	. . . . . . . . 9 |- ( ( ( f " y ) ~~ y /\ y ~~ C ) -> ( f " y ) ~~ C )
26	24, 25	sylan 488	. . . . . . . 8 |- ( ( ( f : A -1-1-onto-> B /\ y C_ A ) /\ y ~~ C ) -> ( f " y ) ~~ C )
27	26	expl 648	. . . . . . 7 |- ( f : A -1-1-onto-> B -> ( ( y C_ A /\ y ~~ C ) -> ( f " y ) ~~ C ) )
28		imassrn 5477	. . . . . . . . 9 |- ( f " y ) C_ ran f
29	28, 10	syl5sseq 3653	. . . . . . . 8 |- ( f : A -onto-> B -> ( f " y ) C_ B )
30	9, 29	syl 17	. . . . . . 7 |- ( f : A -1-1-onto-> B -> ( f " y ) C_ B )
31	27, 30	jctild 566	. . . . . 6 |- ( f : A -1-1-onto-> B -> ( ( y C_ A /\ y ~~ C ) -> ( ( f " y ) C_ B /\ ( f " y ) ~~ C ) ) )
32		elin 3796	. . . . . . 7 |- ( y e. ( ~P A i^i { x | x ~~ C } ) <-> ( y e. ~P A /\ y e. { x | x ~~ C } ) )
33	21	elpw 4164	. . . . . . . 8 |- ( y e. ~P A <-> y C_ A )
34		breq1 4656	. . . . . . . . 9 |- ( x = y -> ( x ~~ C <-> y ~~ C ) )
35	21, 34	elab 3350	. . . . . . . 8 |- ( y e. { x | x ~~ C } <-> y ~~ C )
36	33, 35	anbi12i 733	. . . . . . 7 |- ( ( y e. ~P A /\ y e. { x | x ~~ C } ) <-> ( y C_ A /\ y ~~ C ) )
37	32, 36	bitri 264	. . . . . 6 |- ( y e. ( ~P A i^i { x | x ~~ C } ) <-> ( y C_ A /\ y ~~ C ) )
38		elin 3796	. . . . . . 7 |- ( ( f " y ) e. ( ~P B i^i { x | x ~~ C } ) <-> ( ( f " y ) e. ~P B /\ ( f " y ) e. { x | x ~~ C } ) )
39	3	imaex 7104	. . . . . . . . 9 |- ( f " y ) e. _V
40	39	elpw 4164	. . . . . . . 8 |- ( ( f " y ) e. ~P B <-> ( f " y ) C_ B )
41		breq1 4656	. . . . . . . . 9 |- ( x = ( f " y ) -> ( x ~~ C <-> ( f " y ) ~~ C ) )
42	39, 41	elab 3350	. . . . . . . 8 |- ( ( f " y ) e. { x | x ~~ C } <-> ( f " y ) ~~ C )
43	40, 42	anbi12i 733	. . . . . . 7 |- ( ( ( f " y ) e. ~P B /\ ( f " y ) e. { x | x ~~ C } ) <-> ( ( f " y ) C_ B /\ ( f " y ) ~~ C ) )
44	38, 43	bitri 264	. . . . . 6 |- ( ( f " y ) e. ( ~P B i^i { x | x ~~ C } ) <-> ( ( f " y ) C_ B /\ ( f " y ) ~~ C ) )
45	31, 37, 44	3imtr4g 285	. . . . 5 |- ( f : A -1-1-onto-> B -> ( y e. ( ~P A i^i { x | x ~~ C } ) -> ( f " y ) e. ( ~P B i^i { x | x ~~ C } ) ) )
46		f1ocnv 6149	. . . . . . 7 |- ( f : A -1-1-onto-> B -> `' f : B -1-1-onto-> A )
47		f1of1 6136	. . . . . . . . . . . 12 |- ( `' f : B -1-1-onto-> A -> `' f : B -1-1-> A )
48		f1f1orn 6148	. . . . . . . . . . . 12 |- ( `' f : B -1-1-> A -> `' f : B -1-1-onto-> ran `' f )
49		f1of1 6136	. . . . . . . . . . . 12 |- ( `' f : B -1-1-onto-> ran `' f -> `' f : B -1-1-> ran `' f )
50	47, 48, 49	3syl 18	. . . . . . . . . . 11 |- ( `' f : B -1-1-onto-> A -> `' f : B -1-1-> ran `' f )
51		vex 3203	. . . . . . . . . . . 12 |- z e. _V
52	51	f1imaen 8018	. . . . . . . . . . 11 |- ( ( `' f : B -1-1-> ran `' f /\ z C_ B ) -> ( `' f " z ) ~~ z )
53	50, 52	sylan 488	. . . . . . . . . 10 |- ( ( `' f : B -1-1-onto-> A /\ z C_ B ) -> ( `' f " z ) ~~ z )
54		entr 8008	. . . . . . . . . 10 |- ( ( ( `' f " z ) ~~ z /\ z ~~ C ) -> ( `' f " z ) ~~ C )
55	53, 54	sylan 488	. . . . . . . . 9 |- ( ( ( `' f : B -1-1-onto-> A /\ z C_ B ) /\ z ~~ C ) -> ( `' f " z ) ~~ C )
56	55	expl 648	. . . . . . . 8 |- ( `' f : B -1-1-onto-> A -> ( ( z C_ B /\ z ~~ C ) -> ( `' f " z ) ~~ C ) )
57		f1ofo 6144	. . . . . . . . 9 |- ( `' f : B -1-1-onto-> A -> `' f : B -onto-> A )
58		imassrn 5477	. . . . . . . . . 10 |- ( `' f " z ) C_ ran `' f
59		forn 6118	. . . . . . . . . 10 |- ( `' f : B -onto-> A -> ran `' f = A )
60	58, 59	syl5sseq 3653	. . . . . . . . 9 |- ( `' f : B -onto-> A -> ( `' f " z ) C_ A )
61	57, 60	syl 17	. . . . . . . 8 |- ( `' f : B -1-1-onto-> A -> ( `' f " z ) C_ A )
62	56, 61	jctild 566	. . . . . . 7 |- ( `' f : B -1-1-onto-> A -> ( ( z C_ B /\ z ~~ C ) -> ( ( `' f " z ) C_ A /\ ( `' f " z ) ~~ C ) ) )
63	46, 62	syl 17	. . . . . 6 |- ( f : A -1-1-onto-> B -> ( ( z C_ B /\ z ~~ C ) -> ( ( `' f " z ) C_ A /\ ( `' f " z ) ~~ C ) ) )
64		elin 3796	. . . . . . 7 |- ( z e. ( ~P B i^i { x | x ~~ C } ) <-> ( z e. ~P B /\ z e. { x | x ~~ C } ) )
65	51	elpw 4164	. . . . . . . 8 |- ( z e. ~P B <-> z C_ B )
66		breq1 4656	. . . . . . . . 9 |- ( x = z -> ( x ~~ C <-> z ~~ C ) )
67	51, 66	elab 3350	. . . . . . . 8 |- ( z e. { x | x ~~ C } <-> z ~~ C )
68	65, 67	anbi12i 733	. . . . . . 7 |- ( ( z e. ~P B /\ z e. { x | x ~~ C } ) <-> ( z C_ B /\ z ~~ C ) )
69	64, 68	bitri 264	. . . . . 6 |- ( z e. ( ~P B i^i { x | x ~~ C } ) <-> ( z C_ B /\ z ~~ C ) )
70		elin 3796	. . . . . . 7 |- ( ( `' f " z ) e. ( ~P A i^i { x | x ~~ C } ) <-> ( ( `' f " z ) e. ~P A /\ ( `' f " z ) e. { x | x ~~ C } ) )
71	3	cnvex 7113	. . . . . . . . . 10 |- `' f e. _V
72	71	imaex 7104	. . . . . . . . 9 |- ( `' f " z ) e. _V
73	72	elpw 4164	. . . . . . . 8 |- ( ( `' f " z ) e. ~P A <-> ( `' f " z ) C_ A )
74		breq1 4656	. . . . . . . . 9 |- ( x = ( `' f " z ) -> ( x ~~ C <-> ( `' f " z ) ~~ C ) )
75	72, 74	elab 3350	. . . . . . . 8 |- ( ( `' f " z ) e. { x | x ~~ C } <-> ( `' f " z ) ~~ C )
76	73, 75	anbi12i 733	. . . . . . 7 |- ( ( ( `' f " z ) e. ~P A /\ ( `' f " z ) e. { x | x ~~ C } ) <-> ( ( `' f " z ) C_ A /\ ( `' f " z ) ~~ C ) )
77	70, 76	bitri 264	. . . . . 6 |- ( ( `' f " z ) e. ( ~P A i^i { x | x ~~ C } ) <-> ( ( `' f " z ) C_ A /\ ( `' f " z ) ~~ C ) )
78	63, 69, 77	3imtr4g 285	. . . . 5 |- ( f : A -1-1-onto-> B -> ( z e. ( ~P B i^i { x | x ~~ C } ) -> ( `' f " z ) e. ( ~P A i^i { x | x ~~ C } ) ) )
79		simpl 473	. . . . . . . . . . 11 |- ( ( z e. ~P B /\ z e. { x | x ~~ C } ) -> z e. ~P B )
80	79	elpwid 4170	. . . . . . . . . 10 |- ( ( z e. ~P B /\ z e. { x | x ~~ C } ) -> z C_ B )
81	64, 80	sylbi 207	. . . . . . . . 9 |- ( z e. ( ~P B i^i { x | x ~~ C } ) -> z C_ B )
82		imaeq2 5462	. . . . . . . . . . . 12 |- ( y = ( `' f " z ) -> ( f " y ) = ( f " ( `' f " z ) ) )
83		f1orel 6140	. . . . . . . . . . . . . . . 16 |- ( f : A -1-1-onto-> B -> Rel f )
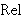84		dfrel2 5583	. . . . . . . . . . . . . . . 16 |- ( Rel f <-> `' `' f = f )
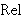
85	83, 84	sylib 208	. . . . . . . . . . . . . . 15 |- ( f : A -1-1-onto-> B -> `' `' f = f )
86	85	imaeq1d 5465	. . . . . . . . . . . . . 14 |- ( f : A -1-1-onto-> B -> ( `' `' f " ( `' f " z ) ) = ( f " ( `' f " z ) ) )
87	86	adantr 481	. . . . . . . . . . . . 13 |- ( ( f : A -1-1-onto-> B /\ z C_ B ) -> ( `' `' f " ( `' f " z ) ) = ( f " ( `' f " z ) ) )
88	46, 47	syl 17	. . . . . . . . . . . . . 14 |- ( f : A -1-1-onto-> B -> `' f : B -1-1-> A )
89		f1imacnv 6153	. . . . . . . . . . . . . 14 |- ( ( `' f : B -1-1-> A /\ z C_ B ) -> ( `' `' f " ( `' f " z ) ) = z )
90	88, 89	sylan 488	. . . . . . . . . . . . 13 |- ( ( f : A -1-1-onto-> B /\ z C_ B ) -> ( `' `' f " ( `' f " z ) ) = z )
91	87, 90	eqtr3d 2658	. . . . . . . . . . . 12 |- ( ( f : A -1-1-onto-> B /\ z C_ B ) -> ( f " ( `' f " z ) ) = z )
92	82, 91	sylan9eqr 2678	. . . . . . . . . . 11 |- ( ( ( f : A -1-1-onto-> B /\ z C_ B ) /\ y = ( `' f " z ) ) -> ( f " y ) = z )
93	92	eqcomd 2628	. . . . . . . . . 10 |- ( ( ( f : A -1-1-onto-> B /\ z C_ B ) /\ y = ( `' f " z ) ) -> z = ( f " y ) )
94	93	ex 450	. . . . . . . . 9 |- ( ( f : A -1-1-onto-> B /\ z C_ B ) -> ( y = ( `' f " z ) -> z = ( f " y ) ) )
95	81, 94	sylan2 491	. . . . . . . 8 |- ( ( f : A -1-1-onto-> B /\ z e. ( ~P B i^i { x | x ~~ C } ) ) -> ( y = ( `' f " z ) -> z = ( f " y ) ) )
96	95	adantrl 752	. . . . . . 7 |- ( ( f : A -1-1-onto-> B /\ ( y e. ( ~P A i^i { x | x ~~ C } ) /\ z e. ( ~P B i^i { x | x ~~ C } ) ) ) -> ( y = ( `' f " z ) -> z = ( f " y ) ) )
97		simpl 473	. . . . . . . . . . 11 |- ( ( y e. ~P A /\ y e. { x | x ~~ C } ) -> y e. ~P A )
98	97	elpwid 4170	. . . . . . . . . 10 |- ( ( y e. ~P A /\ y e. { x | x ~~ C } ) -> y C_ A )
99	32, 98	sylbi 207	. . . . . . . . 9 |- ( y e. ( ~P A i^i { x | x ~~ C } ) -> y C_ A )
100		imaeq2 5462	. . . . . . . . . . . 12 |- ( z = ( f " y ) -> ( `' f " z ) = ( `' f " ( f " y ) ) )
101		f1imacnv 6153	. . . . . . . . . . . . 13 |- ( ( f : A -1-1-> B /\ y C_ A ) -> ( `' f " ( f " y ) ) = y )
102	17, 101	sylan 488	. . . . . . . . . . . 12 |- ( ( f : A -1-1-onto-> B /\ y C_ A ) -> ( `' f " ( f " y ) ) = y )
103	100, 102	sylan9eqr 2678	. . . . . . . . . . 11 |- ( ( ( f : A -1-1-onto-> B /\ y C_ A ) /\ z = ( f " y ) ) -> ( `' f " z ) = y )
104	103	eqcomd 2628	. . . . . . . . . 10 |- ( ( ( f : A -1-1-onto-> B /\ y C_ A ) /\ z = ( f " y ) ) -> y = ( `' f " z ) )
105	104	ex 450	. . . . . . . . 9 |- ( ( f : A -1-1-onto-> B /\ y C_ A ) -> ( z = ( f " y ) -> y = ( `' f " z ) ) )
106	99, 105	sylan2 491	. . . . . . . 8 |- ( ( f : A -1-1-onto-> B /\ y e. ( ~P A i^i { x | x ~~ C } ) ) -> ( z = ( f " y ) -> y = ( `' f " z ) ) )
107	106	adantrr 753	. . . . . . 7 |- ( ( f : A -1-1-onto-> B /\ ( y e. ( ~P A i^i { x | x ~~ C } ) /\ z e. ( ~P B i^i { x | x ~~ C } ) ) ) -> ( z = ( f " y ) -> y = ( `' f " z ) ) )
108	96, 107	impbid 202	. . . . . 6 |- ( ( f : A -1-1-onto-> B /\ ( y e. ( ~P A i^i { x | x ~~ C } ) /\ z e. ( ~P B i^i { x | x ~~ C } ) ) ) -> ( y = ( `' f " z ) <-> z = ( f " y ) ) )
109	108	ex 450	. . . . 5 |- ( f : A -1-1-onto-> B -> ( ( y e. ( ~P A i^i { x | x ~~ C } ) /\ z e. ( ~P B i^i { x | x ~~ C } ) ) -> ( y = ( `' f " z ) <-> z = ( f " y ) ) ) )
110	8, 16, 45, 78, 109	en3d 7992	. . . 4 |- ( f : A -1-1-onto-> B -> ( ~P A i^i { x | x ~~ C } ) ~~ ( ~P B i^i { x | x ~~ C } ) )
111	110	exlimiv 1858	. . 3 |- ( E. f f : A -1-1-onto-> B -> ( ~P A i^i { x | x ~~ C } ) ~~ ( ~P B i^i { x | x ~~ C } ) )
112	1, 111	sylbi 207	. 2 |- ( A ~~ B -> ( ~P A i^i { x | x ~~ C } ) ~~ ( ~P B i^i { x | x ~~ C } ) )
113		df-pw 4160	. . . 4 |- ~P A = { x | x C_ A }
114	113	ineq1i 3810	. . 3 |- ( ~P A i^i { x | x ~~ C } ) = ( { x | x C_ A } i^i { x | x ~~ C } )
115		inab 3895	. . 3 |- ( { x | x C_ A } i^i { x | x ~~ C } ) = { x | ( x C_ A /\ x ~~ C ) }
116	114, 115	eqtri 2644	. 2 |- ( ~P A i^i { x | x ~~ C } ) = { x | ( x C_ A /\ x ~~ C ) }
117		df-pw 4160	. . . 4 |- ~P B = { x | x C_ B }
118	117	ineq1i 3810	. . 3 |- ( ~P B i^i { x | x ~~ C } ) = ( { x | x C_ B } i^i { x | x ~~ C } )
119		inab 3895	. . 3 |- ( { x | x C_ B } i^i { x | x ~~ C } ) = { x | ( x C_ B /\ x ~~ C ) }
120	118, 119	eqtri 2644	. 2 |- ( ~P B i^i { x | x ~~ C } ) = { x | ( x C_ B /\ x ~~ C ) }
121	112, 116, 120	3brtr3g 4686	1 |- ( A ~~ B -> { x | ( x C_ A /\ x ~~ C ) } ~~ { x | ( x C_ B /\ x ~~ C ) } )

Syntax hints:   -> wi 4   <-> wb 196   /\ wa 384   = wceq 1483   E.wex 1704   e. wcel 1990   {cab 2608   _Vcvv 3200   i^i cin 3573   C_ wss 3574   ~Pcpw 4158   class class class wbr 4653   `'ccnv 5113   dom cdm 5114   ran crn 5115   "cima 5117   Rel wrel 5119   -1-1->wf1 5885   -onto->wfo 5886   -1-1-onto->wf1o 5887   ~~ cen 7952

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-rep 4771  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-ral 2917  df-rex 2918  df-reu 2919  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-op 4184  df-uni 4437  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-id 5024  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-er 7742  df-en 7956

This theorem is referenced by:  infmap2  9040