Theorem wefrc 5108

Description: A nonempty (possibly proper) subclass of a class well-ordered by _E has a minimal element. Special case of Proposition 6.26 of [TakeutiZaring] p. 31. (Contributed by NM, 17-Feb-2004.)

Assertion

Ref	Expression
wefrc	|- ( ( _E We A /\ B C_ A /\ B =/= (/) ) -> E. x e. B ( B i^i x ) = (/) )

Distinct variable group:   x, B

Allowed substitution hint:   A( x)

Proof of Theorem wefrc

Dummy variables y z are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		wess 5101	. . 3 |- ( B C_ A -> ( _E We A -> _E We B ) )
2		n0 3931	. . . 4 |- ( B =/= (/) <-> E. y y e. B )
3		ineq2 3808	. . . . . . . . . . 11 |- ( x = y -> ( B i^i x ) = ( B i^i y ) )
4	3	eqeq1d 2624	. . . . . . . . . 10 |- ( x = y -> ( ( B i^i x ) = (/) <-> ( B i^i y ) = (/) ) )
5	4	rspcev 3309	. . . . . . . . 9 |- ( ( y e. B /\ ( B i^i y ) = (/) ) -> E. x e. B ( B i^i x ) = (/) )
6	5	ex 450	. . . . . . . 8 |- ( y e. B -> ( ( B i^i y ) = (/) -> E. x e. B ( B i^i x ) = (/) ) )
7	6	adantl 482	. . . . . . 7 |- ( ( _E We B /\ y e. B ) -> ( ( B i^i y ) = (/) -> E. x e. B ( B i^i x ) = (/) ) )
8		inss1 3833	. . . . . . . . . . 11 |- ( B i^i y ) C_ B
9		wefr 5104	. . . . . . . . . . . . 13 |- ( _E We B -> _E Fr B )
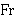10		vex 3203	. . . . . . . . . . . . . . 15 |- y e. _V
11	10	inex2 4800	. . . . . . . . . . . . . 14 |- ( B i^i y ) e. _V
12	11	epfrc 5100	. . . . . . . . . . . . 13 |- ( ( _E Fr B /\ ( B i^i y ) C_ B /\ ( B i^i y ) =/= (/) ) -> E. x e. ( B i^i y ) ( ( B i^i y ) i^i x ) = (/) )
13	9, 12	syl3an1 1359	. . . . . . . . . . . 12 |- ( ( _E We B /\ ( B i^i y ) C_ B /\ ( B i^i y ) =/= (/) ) -> E. x e. ( B i^i y ) ( ( B i^i y ) i^i x ) = (/) )
14	13	3exp 1264	. . . . . . . . . . 11 |- ( _E We B -> ( ( B i^i y ) C_ B -> ( ( B i^i y ) =/= (/) -> E. x e. ( B i^i y ) ( ( B i^i y ) i^i x ) = (/) ) ) )
15	8, 14	mpi 20	. . . . . . . . . 10 |- ( _E We B -> ( ( B i^i y ) =/= (/) -> E. x e. ( B i^i y ) ( ( B i^i y ) i^i x ) = (/) ) )
16		elin 3796	. . . . . . . . . . . . 13 |- ( x e. ( B i^i y ) <-> ( x e. B /\ x e. y ) )
17	16	anbi1i 731	. . . . . . . . . . . 12 |- ( ( x e. ( B i^i y ) /\ ( ( B i^i y ) i^i x ) = (/) ) <-> ( ( x e. B /\ x e. y ) /\ ( ( B i^i y ) i^i x ) = (/) ) )
18		anass 681	. . . . . . . . . . . 12 |- ( ( ( x e. B /\ x e. y ) /\ ( ( B i^i y ) i^i x ) = (/) ) <-> ( x e. B /\ ( x e. y /\ ( ( B i^i y ) i^i x ) = (/) ) ) )
19	17, 18	bitri 264	. . . . . . . . . . 11 |- ( ( x e. ( B i^i y ) /\ ( ( B i^i y ) i^i x ) = (/) ) <-> ( x e. B /\ ( x e. y /\ ( ( B i^i y ) i^i x ) = (/) ) ) )
20	19	rexbii2 3039	. . . . . . . . . 10 |- ( E. x e. ( B i^i y ) ( ( B i^i y ) i^i x ) = (/) <-> E. x e. B ( x e. y /\ ( ( B i^i y ) i^i x ) = (/) ) )
21	15, 20	syl6ib 241	. . . . . . . . 9 |- ( _E We B -> ( ( B i^i y ) =/= (/) -> E. x e. B ( x e. y /\ ( ( B i^i y ) i^i x ) = (/) ) ) )
22	21	adantr 481	. . . . . . . 8 |- ( ( _E We B /\ y e. B ) -> ( ( B i^i y ) =/= (/) -> E. x e. B ( x e. y /\ ( ( B i^i y ) i^i x ) = (/) ) ) )
23		elin 3796	. . . . . . . . . . . . . . . . 17 |- ( z e. ( B i^i x ) <-> ( z e. B /\ z e. x ) )
24		df-3an 1039	. . . . . . . . . . . . . . . . . . . . . . 23 |- ( ( y e. B /\ z e. B /\ x e. B ) <-> ( ( y e. B /\ z e. B ) /\ x e. B ) )
25		3anrot 1043	. . . . . . . . . . . . . . . . . . . . . . 23 |- ( ( y e. B /\ z e. B /\ x e. B ) <-> ( z e. B /\ x e. B /\ y e. B ) )
26	24, 25	bitr3i 266	. . . . . . . . . . . . . . . . . . . . . 22 |- ( ( ( y e. B /\ z e. B ) /\ x e. B ) <-> ( z e. B /\ x e. B /\ y e. B ) )
27		wetrep 5107	. . . . . . . . . . . . . . . . . . . . . . 23 |- ( ( _E We B /\ ( z e. B /\ x e. B /\ y e. B ) ) -> ( ( z e. x /\ x e. y ) -> z e. y ) )
28	27	expd 452	. . . . . . . . . . . . . . . . . . . . . 22 |- ( ( _E We B /\ ( z e. B /\ x e. B /\ y e. B ) ) -> ( z e. x -> ( x e. y -> z e. y ) ) )
29	26, 28	sylan2b 492	. . . . . . . . . . . . . . . . . . . . 21 |- ( ( _E We B /\ ( ( y e. B /\ z e. B ) /\ x e. B ) ) -> ( z e. x -> ( x e. y -> z e. y ) ) )
30	29	exp44 641	. . . . . . . . . . . . . . . . . . . 20 |- ( _E We B -> ( y e. B -> ( z e. B -> ( x e. B -> ( z e. x -> ( x e. y -> z e. y ) ) ) ) ) )
31	30	imp 445	. . . . . . . . . . . . . . . . . . 19 |- ( ( _E We B /\ y e. B ) -> ( z e. B -> ( x e. B -> ( z e. x -> ( x e. y -> z e. y ) ) ) ) )
32	31	com34 91	. . . . . . . . . . . . . . . . . 18 |- ( ( _E We B /\ y e. B ) -> ( z e. B -> ( z e. x -> ( x e. B -> ( x e. y -> z e. y ) ) ) ) )
33	32	impd 447	. . . . . . . . . . . . . . . . 17 |- ( ( _E We B /\ y e. B ) -> ( ( z e. B /\ z e. x ) -> ( x e. B -> ( x e. y -> z e. y ) ) ) )
34	23, 33	syl5bi 232	. . . . . . . . . . . . . . . 16 |- ( ( _E We B /\ y e. B ) -> ( z e. ( B i^i x ) -> ( x e. B -> ( x e. y -> z e. y ) ) ) )
35	34	imp4a 614	. . . . . . . . . . . . . . 15 |- ( ( _E We B /\ y e. B ) -> ( z e. ( B i^i x ) -> ( ( x e. B /\ x e. y ) -> z e. y ) ) )
36	35	com23 86	. . . . . . . . . . . . . 14 |- ( ( _E We B /\ y e. B ) -> ( ( x e. B /\ x e. y ) -> ( z e. ( B i^i x ) -> z e. y ) ) )
37	36	ralrimdv 2968	. . . . . . . . . . . . 13 |- ( ( _E We B /\ y e. B ) -> ( ( x e. B /\ x e. y ) -> A. z e. ( B i^i x ) z e. y ) )
38		dfss3 3592	. . . . . . . . . . . . 13 |- ( ( B i^i x ) C_ y <-> A. z e. ( B i^i x ) z e. y )
39	37, 38	syl6ibr 242	. . . . . . . . . . . 12 |- ( ( _E We B /\ y e. B ) -> ( ( x e. B /\ x e. y ) -> ( B i^i x ) C_ y ) )
40		dfss 3589	. . . . . . . . . . . . . . 15 |- ( ( B i^i x ) C_ y <-> ( B i^i x ) = ( ( B i^i x ) i^i y ) )
41		in32 3825	. . . . . . . . . . . . . . . 16 |- ( ( B i^i x ) i^i y ) = ( ( B i^i y ) i^i x )
42	41	eqeq2i 2634	. . . . . . . . . . . . . . 15 |- ( ( B i^i x ) = ( ( B i^i x ) i^i y ) <-> ( B i^i x ) = ( ( B i^i y ) i^i x ) )
43	40, 42	sylbb 209	. . . . . . . . . . . . . 14 |- ( ( B i^i x ) C_ y -> ( B i^i x ) = ( ( B i^i y ) i^i x ) )
44	43	eqeq1d 2624	. . . . . . . . . . . . 13 |- ( ( B i^i x ) C_ y -> ( ( B i^i x ) = (/) <-> ( ( B i^i y ) i^i x ) = (/) ) )
45	44	biimprd 238	. . . . . . . . . . . 12 |- ( ( B i^i x ) C_ y -> ( ( ( B i^i y ) i^i x ) = (/) -> ( B i^i x ) = (/) ) )
46	39, 45	syl6 35	. . . . . . . . . . 11 |- ( ( _E We B /\ y e. B ) -> ( ( x e. B /\ x e. y ) -> ( ( ( B i^i y ) i^i x ) = (/) -> ( B i^i x ) = (/) ) ) )
47	46	expd 452	. . . . . . . . . 10 |- ( ( _E We B /\ y e. B ) -> ( x e. B -> ( x e. y -> ( ( ( B i^i y ) i^i x ) = (/) -> ( B i^i x ) = (/) ) ) ) )
48	47	imp4a 614	. . . . . . . . 9 |- ( ( _E We B /\ y e. B ) -> ( x e. B -> ( ( x e. y /\ ( ( B i^i y ) i^i x ) = (/) ) -> ( B i^i x ) = (/) ) ) )
49	48	reximdvai 3015	. . . . . . . 8 |- ( ( _E We B /\ y e. B ) -> ( E. x e. B ( x e. y /\ ( ( B i^i y ) i^i x ) = (/) ) -> E. x e. B ( B i^i x ) = (/) ) )
50	22, 49	syld 47	. . . . . . 7 |- ( ( _E We B /\ y e. B ) -> ( ( B i^i y ) =/= (/) -> E. x e. B ( B i^i x ) = (/) ) )
51	7, 50	pm2.61dne 2880	. . . . . 6 |- ( ( _E We B /\ y e. B ) -> E. x e. B ( B i^i x ) = (/) )
52	51	ex 450	. . . . 5 |- ( _E We B -> ( y e. B -> E. x e. B ( B i^i x ) = (/) ) )
53	52	exlimdv 1861	. . . 4 |- ( _E We B -> ( E. y y e. B -> E. x e. B ( B i^i x ) = (/) ) )
54	2, 53	syl5bi 232	. . 3 |- ( _E We B -> ( B =/= (/) -> E. x e. B ( B i^i x ) = (/) ) )
55	1, 54	syl6com 37	. 2 |- ( _E We A -> ( B C_ A -> ( B =/= (/) -> E. x e. B ( B i^i x ) = (/) ) ) )
56	55	3imp 1256	1 |- ( ( _E We A /\ B C_ A /\ B =/= (/) ) -> E. x e. B ( B i^i x ) = (/) )

Syntax hints:   -> wi 4   /\ wa 384   /\ w3a 1037   = wceq 1483   E.wex 1704   e. wcel 1990   =/= wne 2794   A.wral 2912   E.wrex 2913   i^i cin 3573   C_ wss 3574   (/)c0 3915   _E cep 5028   Fr wfr 5070   We wwe 5072

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-sep 4781  ax-nul 4789  ax-pr 4906

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-ral 2917  df-rex 2918  df-rab 2921  df-v 3202  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-nul 3916  df-if 4087  df-sn 4178  df-pr 4180  df-op 4184  df-br 4654  df-opab 4713  df-eprel 5029  df-po 5035  df-so 5036  df-fr 5073  df-we 5075

This theorem is referenced by:  tz7.5  5744  onnseq  7441  finminlem  32312