| Step | Hyp | Ref
| Expression |
|---|
| 1 | | ne0i 3921 |
. . 3
        evalSub evalSub      
    evalSub evalSub         |
| 2 | | mpfrcl.q |
. . 3
       evalSub evalSub      |
| 3 | 1, 2 | eleq2s 2719 |
. 2
          evalSub evalSub         |
| 4 | | rneq 5351 |
. . . 4
     evalSub evalSub         
   evalSub evalSub          |
| 5 | | rn0 5377 |
. . . 4
     |
| 6 | 4, 5 | syl6eq 2672 |
. . 3
     evalSub evalSub         
   evalSub evalSub         |
| 7 | 6 | necon3i 2826 |
. 2
      evalSub evalSub        
   evalSub evalSub         |
| 8 | | fveq1 6190 |
. . . . . . 7
    evalSub evalSub         evalSub evalSub             |
| 9 | | 0fv 6227 |
. . . . . . 7
        |
| 10 | 8, 9 | syl6eq 2672 |
. . . . . 6
    evalSub evalSub         evalSub evalSub         |
| 11 | 10 | necon3i 2826 |
. . . . 5
     evalSub evalSub           evalSub evalSub      |
| 12 | | reldmevls 19517 |
. . . . . . . 8
 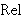  evalSub evalSub |
| 13 | 12 | ovprc1 6684 |
. . . . . . 7
         evalSub evalSub      |
| 14 | 13 | necon1ai 2821 |
. . . . . 6
    evalSub evalSub          |
| 15 | | n0 3931 |
. . . . . . 7
    evalSub evalSub     
      evalSub evalSub    |
| 16 | | df-evls 19506 |
. . . . . . . . . 10
 evalSub evalSub                   ![]_ ]_](_urbrack.gif)     SubRing SubRing       mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                               |
| 17 | 16 | elmpt2cl2 6878 |
. . . . . . . . 9
      evalSub evalSub   
    |
| 18 | 17 | a1d 25 |
. . . . . . . 8
      evalSub evalSub   
    
     |
| 19 | 18 | exlimiv 1858 |
. . . . . . 7
        evalSub evalSub              |
| 20 | 15, 19 | sylbi 207 |
. . . . . 6
    evalSub evalSub                |
| 21 | 14, 20 | jcai 559 |
. . . . 5
    evalSub evalSub            
   |
| 22 | 11, 21 | syl 17 |
. . . 4
     evalSub evalSub             
     |
| 23 | | fvex 6201 |
. . . . . . . . . . . . 13
        |
| 24 | | nfcv 2764 |
. . . . . . . . . . . . . 14
    SubRing SubRing   |
| 25 | | nfcsb1v 3549 |
. . . . . . . . . . . . . 14
           ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                             |
| 26 | 24, 25 | nfmpt 4746 |
. . . . . . . . . . . . 13
       SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s
↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                              |
| 27 | | csbeq1a 3542 |
. . . . . . . . . . . . . 14
         
   mPoly mPoly   ↾s
↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                                      ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                              |
| 28 | 27 | mpteq2dv 4745 |
. . . . . . . . . . . . 13
         
    SubRing SubRing       mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                                   SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                               |
| 29 | 23, 26, 28 | csbief 3558 |
. . . . . . . . . . . 12
         ![]_ ]_](_urbrack.gif)     SubRing SubRing       mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                                   SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                              |
| 30 | | fveq2 6191 |
. . . . . . . . . . . . . 14
       SubRing SubRing     SubRing SubRing    |
| 31 | 30 | adantl 482 |
. . . . . . . . . . . . 13
        
    SubRing SubRing     SubRing SubRing    |
| 32 | | fveq2 6191 |
. . . . . . . . . . . . . . . 16
                  |
| 33 | 32 | adantl 482 |
. . . . . . . . . . . . . . 15
        
               |
| 34 | 33 | csbeq1d 3540 |
. . . . . . . . . . . . . 14
        
           ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                      ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s
↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                              |
| 35 | | id 22 |
. . . . . . . . . . . . . . . . . 18
          |
| 36 | | oveq1 6657 |
. . . . . . . . . . . . . . . . . 18
        ↾s ↾s      ↾s ↾s    |
| 37 | 35, 36 | oveqan12d 6669 |
. . . . . . . . . . . . . . . . 17
        
     mPoly mPoly   ↾s ↾s       mPoly mPoly   ↾s ↾s     |
| 38 | 37 | csbeq1d 3540 |
. . . . . . . . . . . . . . . 16
        
      mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                                 mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                              |
| 39 | | id 22 |
. . . . . . . . . . . . . . . . . . . 20
          |
| 40 | | oveq2 6658 |
. . . . . . . . . . . . . . . . . . . 20
        
         |
| 41 | 39, 40 | oveqan12rd 6670 |
. . . . . . . . . . . . . . . . . . 19
        
      s s           s s   
    |
| 42 | 41 | oveq2d 6666 |
. . . . . . . . . . . . . . . . . 18
        
     RingHom RingHom    s s           RingHom RingHom    s s         |
| 43 | 40 | adantr 481 |
. . . . . . . . . . . . . . . . . . . . . 22
        
               |
| 44 | 43 | xpeq1d 5138 |
. . . . . . . . . . . . . . . . . . . . 21
        
                           |
| 45 | 44 | mpteq2dv 4745 |
. . . . . . . . . . . . . . . . . . . 20
        
                                       |
| 46 | 45 | eqeq2d 2632 |
. . . . . . . . . . . . . . . . . . 19
        
        algSc algSc                      
    algSc algSc                        |
| 47 | 35, 36 | oveqan12d 6669 |
. . . . . . . . . . . . . . . . . . . . 21
        
     mVar mVar   ↾s ↾s       mVar mVar   ↾s ↾s     |
| 48 | 47 | coeq2d 5284 |
. . . . . . . . . . . . . . . . . . . 20
        
        mVar mVar   ↾s ↾s           mVar mVar   ↾s
↾s      |
| 49 | | simpl 473 |
. . . . . . . . . . . . . . . . . . . . 21
        
       |
| 50 | 43 | mpteq1d 4738 |
. . . . . . . . . . . . . . . . . . . . 21
        
                                   |
| 51 | 49, 50 | mpteq12dv 4733 |
. . . . . . . . . . . . . . . . . . . 20
        
                                               |
| 52 | 48, 51 | eqeq12d 2637 |
. . . . . . . . . . . . . . . . . . 19
        
         mVar mVar   ↾s ↾s                           
     mVar mVar   ↾s ↾s                             |
| 53 | 46, 52 | anbi12d 747 |
. . . . . . . . . . . . . . . . . 18
        
         algSc algSc                            mVar mVar   ↾s ↾s                            
     algSc algSc                            mVar mVar   ↾s ↾s                              |
| 54 | 42, 53 | riotaeqbidv 6614 |
. . . . . . . . . . . . . . . . 17
        
         RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                    RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                              |
| 55 | 54 | csbeq2dv 3992 |
. . . . . . . . . . . . . . . 16
        
      mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                                 mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                              |
| 56 | 38, 55 | eqtrd 2656 |
. . . . . . . . . . . . . . 15
        
      mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                                 mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                              |
| 57 | 56 | csbeq2dv 3992 |
. . . . . . . . . . . . . 14
        
           ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                      ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s
↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                              |
| 58 | 34, 57 | eqtrd 2656 |
. . . . . . . . . . . . 13
        
           ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                      ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s
↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                              |
| 59 | 31, 58 | mpteq12dv 4733 |
. . . . . . . . . . . 12
        
       SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s
↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s             algSc algSc                            mVar mVar   ↾s ↾s                                   SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                               |
| 60 | 29, 59 | syl5eq 2668 |
. . . . . . . . . . 11
        
           ![]_ ]_](_urbrack.gif)     SubRing SubRing       mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                   SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                               |
| 61 | | fvex 6201 |
. . . . . . . . . . . 12
  SubRing SubRing     |
| 62 | 61 | mptex 6486 |
. . . . . . . . . . 11
     SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                |
| 63 | 60, 16, 62 | ovmpt2a 6791 |
. . . . . . . . . 10
        
     evalSub evalSub
       SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                               |
| 64 | 63 | dmeqd 5326 |
. . . . . . . . 9
        
      evalSub evalSub         SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                               |
| 65 | | eqid 2622 |
. . . . . . . . . 10
     SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                   SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                              |
| 66 | 65 | dmmptss 5631 |
. . . . . . . . 9
      SubRing SubRing            ![]_ ]_](_urbrack.gif)    mPoly mPoly   ↾s ↾s      ![]_ ]_](_urbrack.gif)       RingHom RingHom    s s   
         algSc algSc                            mVar mVar   ↾s ↾s                                SubRing SubRing   |
| 67 | 64, 66 | syl6eqss 3655 |
. . . . . . . 8
        
      evalSub evalSub     SubRing SubRing    |
| 68 | 67 | ssneld 3605 |
. . . . . . 7
        
        SubRing SubRing          evalSub
evalSub     |
| 69 | | ndmfv 6218 |
. . . . . . 7
        evalSub evalSub       evalSub evalSub
        |
| 70 | 68, 69 | syl6 35 |
. . . . . 6
        
        SubRing SubRing       evalSub evalSub          |
| 71 | 70 | necon1ad 2811 |
. . . . 5
        
       evalSub evalSub            SubRing SubRing     |
| 72 | 71 | com12 32 |
. . . 4
     evalSub evalSub                       SubRing SubRing     |
| 73 | 22, 72 | jcai 559 |
. . 3
     evalSub evalSub                       SubRing SubRing     |
| 74 | | df-3an 1039 |
. . 3
        
     SubRing SubRing                   SubRing SubRing     |
| 75 | 73, 74 | sylibr 224 |
. 2
     evalSub evalSub             
    
  SubRing SubRing     |
| 76 | 3, 7, 75 | 3syl 18 |
1
            
     SubRing SubRing     |