Theorem tgphaus 21920

Description: A topological group is Hausdorff iff the identity subgroup is closed. (Contributed by Mario Carneiro, 18-Sep-2015.)

Hypotheses

Ref	Expression
tgphaus.1	|- .0. = ( 0g ` G )
tgphaus.j	|- J = ( TopOpen ` G )

Assertion

Ref	Expression
tgphaus	|- ( G e. TopGrp -> ( J e. Haus <-> { .0. } e. ( Clsd ` J ) ) )

Proof of Theorem tgphaus

Dummy variables x y are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		tgpgrp 21882	. . . . 5 |- ( G e. TopGrp -> G e. Grp )
2		eqid 2622	. . . . . 6 |- ( Base ` G ) = ( Base ` G )
3		tgphaus.1	. . . . . 6 |- .0. = ( 0g ` G )
4	2, 3	grpidcl 17450	. . . . 5 |- ( G e. Grp -> .0. e. ( Base ` G ) )
5	1, 4	syl 17	. . . 4 |- ( G e. TopGrp -> .0. e. ( Base ` G ) )
6		tgphaus.j	. . . . . 6 |- J = ( TopOpen ` G )
7	6, 2	tgptopon 21886	. . . . 5 |- ( G e. TopGrp -> J e. (TopOn ` ( Base ` G ) ) )
8		toponuni 20719	. . . . 5 |- ( J e. (TopOn ` ( Base ` G ) ) -> ( Base ` G ) = U. J )
9	7, 8	syl 17	. . . 4 |- ( G e. TopGrp -> ( Base ` G ) = U. J )
10	5, 9	eleqtrd 2703	. . 3 |- ( G e. TopGrp -> .0. e. U. J )
11		eqid 2622	. . . . 5 |- U. J = U. J
12	11	sncld 21175	. . . 4 |- ( ( J e. Haus /\ .0. e. U. J ) -> { .0. } e. ( Clsd ` J ) )
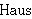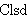13	12	expcom 451	. . 3 |- ( .0. e. U. J -> ( J e. Haus -> { .0. } e. ( Clsd ` J ) ) )
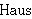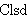14	10, 13	syl 17	. 2 |- ( G e. TopGrp -> ( J e. Haus -> { .0. } e. ( Clsd ` J ) ) )
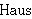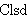15		eqid 2622	. . . . . 6 |- ( -g ` G ) = ( -g ` G )
16	6, 15	tgpsubcn 21894	. . . . 5 |- ( G e. TopGrp -> ( -g ` G ) e. ( ( J tX J ) Cn J ) )
17		cnclima 21072	. . . . . 6 |- ( ( ( -g ` G ) e. ( ( J tX J ) Cn J ) /\ { .0. } e. ( Clsd ` J ) ) -> ( `' ( -g ` G ) " { .0. } ) e. ( Clsd ` ( J tX J ) ) )
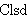
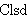18	17	ex 450	. . . . 5 |- ( ( -g ` G ) e. ( ( J tX J ) Cn J ) -> ( { .0. } e. ( Clsd ` J ) -> ( `' ( -g ` G ) " { .0. } ) e. ( Clsd ` ( J tX J ) ) ) )
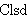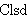19	16, 18	syl 17	. . . 4 |- ( G e. TopGrp -> ( { .0. } e. ( Clsd ` J ) -> ( `' ( -g ` G ) " { .0. } ) e. ( Clsd ` ( J tX J ) ) ) )
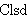
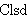20		cnvimass 5485	. . . . . . . . 9 |- ( `' ( -g ` G ) " { .0. } ) C_ dom ( -g ` G )
21	2, 15	grpsubf 17494	. . . . . . . . . . 11 |- ( G e. Grp -> ( -g ` G ) : ( ( Base ` G ) X. ( Base ` G ) ) --> ( Base ` G ) )
22	1, 21	syl 17	. . . . . . . . . 10 |- ( G e. TopGrp -> ( -g ` G ) : ( ( Base ` G ) X. ( Base ` G ) ) --> ( Base ` G ) )
23		fdm 6051	. . . . . . . . . 10 |- ( ( -g ` G ) : ( ( Base ` G ) X. ( Base ` G ) ) --> ( Base ` G ) -> dom ( -g ` G ) = ( ( Base ` G ) X. ( Base ` G ) ) )
24	22, 23	syl 17	. . . . . . . . 9 |- ( G e. TopGrp -> dom ( -g ` G ) = ( ( Base ` G ) X. ( Base ` G ) ) )
25	20, 24	syl5sseq 3653	. . . . . . . 8 |- ( G e. TopGrp -> ( `' ( -g ` G ) " { .0. } ) C_ ( ( Base ` G ) X. ( Base ` G ) ) )
26		relxp 5227	. . . . . . . 8 |- Rel ( ( Base ` G ) X. ( Base ` G ) )
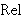27		relss 5206	. . . . . . . 8 |- ( ( `' ( -g ` G ) " { .0. } ) C_ ( ( Base ` G ) X. ( Base ` G ) ) -> ( Rel ( ( Base ` G ) X. ( Base ` G ) ) -> Rel ( `' ( -g ` G ) " { .0. } ) ) )
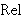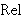28	25, 26, 27	mpisyl 21	. . . . . . 7 |- ( G e. TopGrp -> Rel ( `' ( -g ` G ) " { .0. } ) )
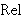
29		dfrel4v 5584	. . . . . . 7 |- ( Rel ( `' ( -g ` G ) " { .0. } ) <-> ( `' ( -g ` G ) " { .0. } ) = { <. x , y >. | x ( `' ( -g ` G ) " { .0. } ) y } )
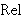
30	28, 29	sylib 208	. . . . . 6 |- ( G e. TopGrp -> ( `' ( -g ` G ) " { .0. } ) = { <. x , y >. | x ( `' ( -g ` G ) " { .0. } ) y } )
31		ffn 6045	. . . . . . . . . . . 12 |- ( ( -g ` G ) : ( ( Base ` G ) X. ( Base ` G ) ) --> ( Base ` G ) -> ( -g ` G ) Fn ( ( Base ` G ) X. ( Base ` G ) ) )
32	22, 31	syl 17	. . . . . . . . . . 11 |- ( G e. TopGrp -> ( -g ` G ) Fn ( ( Base ` G ) X. ( Base ` G ) ) )
33		elpreima 6337	. . . . . . . . . . 11 |- ( ( -g ` G ) Fn ( ( Base ` G ) X. ( Base ` G ) ) -> ( <. x , y >. e. ( `' ( -g ` G ) " { .0. } ) <-> ( <. x , y >. e. ( ( Base ` G ) X. ( Base ` G ) ) /\ ( ( -g ` G ) ` <. x , y >. ) e. { .0. } ) ) )
34	32, 33	syl 17	. . . . . . . . . 10 |- ( G e. TopGrp -> ( <. x , y >. e. ( `' ( -g ` G ) " { .0. } ) <-> ( <. x , y >. e. ( ( Base ` G ) X. ( Base ` G ) ) /\ ( ( -g ` G ) ` <. x , y >. ) e. { .0. } ) ) )
35		opelxp 5146	. . . . . . . . . . . 12 |- ( <. x , y >. e. ( ( Base ` G ) X. ( Base ` G ) ) <-> ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) )
36	35	anbi1i 731	. . . . . . . . . . 11 |- ( ( <. x , y >. e. ( ( Base ` G ) X. ( Base ` G ) ) /\ ( ( -g ` G ) ` <. x , y >. ) e. { .0. } ) <-> ( ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) /\ ( ( -g ` G ) ` <. x , y >. ) e. { .0. } ) )
37	2, 3, 15	grpsubeq0 17501	. . . . . . . . . . . . . . 15 |- ( ( G e. Grp /\ x e. ( Base ` G ) /\ y e. ( Base ` G ) ) -> ( ( x ( -g ` G ) y ) = .0. <-> x = y ) )
38	37	3expb 1266	. . . . . . . . . . . . . 14 |- ( ( G e. Grp /\ ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) ) -> ( ( x ( -g ` G ) y ) = .0. <-> x = y ) )
39	1, 38	sylan 488	. . . . . . . . . . . . 13 |- ( ( G e. TopGrp /\ ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) ) -> ( ( x ( -g ` G ) y ) = .0. <-> x = y ) )
40		df-ov 6653	. . . . . . . . . . . . . . 15 |- ( x ( -g ` G ) y ) = ( ( -g ` G ) ` <. x , y >. )
41	40	eleq1i 2692	. . . . . . . . . . . . . 14 |- ( ( x ( -g ` G ) y ) e. { .0. } <-> ( ( -g ` G ) ` <. x , y >. ) e. { .0. } )
42		ovex 6678	. . . . . . . . . . . . . . 15 |- ( x ( -g ` G ) y ) e. _V
43	42	elsn 4192	. . . . . . . . . . . . . 14 |- ( ( x ( -g ` G ) y ) e. { .0. } <-> ( x ( -g ` G ) y ) = .0. )
44	41, 43	bitr3i 266	. . . . . . . . . . . . 13 |- ( ( ( -g ` G ) ` <. x , y >. ) e. { .0. } <-> ( x ( -g ` G ) y ) = .0. )
45		equcom 1945	. . . . . . . . . . . . 13 |- ( y = x <-> x = y )
46	39, 44, 45	3bitr4g 303	. . . . . . . . . . . 12 |- ( ( G e. TopGrp /\ ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) ) -> ( ( ( -g ` G ) ` <. x , y >. ) e. { .0. } <-> y = x ) )
47	46	pm5.32da 673	. . . . . . . . . . 11 |- ( G e. TopGrp -> ( ( ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) /\ ( ( -g ` G ) ` <. x , y >. ) e. { .0. } ) <-> ( ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) /\ y = x ) ) )
48	36, 47	syl5bb 272	. . . . . . . . . 10 |- ( G e. TopGrp -> ( ( <. x , y >. e. ( ( Base ` G ) X. ( Base ` G ) ) /\ ( ( -g ` G ) ` <. x , y >. ) e. { .0. } ) <-> ( ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) /\ y = x ) ) )
49	34, 48	bitrd 268	. . . . . . . . 9 |- ( G e. TopGrp -> ( <. x , y >. e. ( `' ( -g ` G ) " { .0. } ) <-> ( ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) /\ y = x ) ) )
50		df-br 4654	. . . . . . . . 9 |- ( x ( `' ( -g ` G ) " { .0. } ) y <-> <. x , y >. e. ( `' ( -g ` G ) " { .0. } ) )
51		eleq1 2689	. . . . . . . . . . . 12 |- ( y = x -> ( y e. ( Base ` G ) <-> x e. ( Base ` G ) ) )
52	51	biimparc 504	. . . . . . . . . . 11 |- ( ( x e. ( Base ` G ) /\ y = x ) -> y e. ( Base ` G ) )
53	52	pm4.71i 664	. . . . . . . . . 10 |- ( ( x e. ( Base ` G ) /\ y = x ) <-> ( ( x e. ( Base ` G ) /\ y = x ) /\ y e. ( Base ` G ) ) )
54		an32 839	. . . . . . . . . 10 |- ( ( ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) /\ y = x ) <-> ( ( x e. ( Base ` G ) /\ y = x ) /\ y e. ( Base ` G ) ) )
55	53, 54	bitr4i 267	. . . . . . . . 9 |- ( ( x e. ( Base ` G ) /\ y = x ) <-> ( ( x e. ( Base ` G ) /\ y e. ( Base ` G ) ) /\ y = x ) )
56	49, 50, 55	3bitr4g 303	. . . . . . . 8 |- ( G e. TopGrp -> ( x ( `' ( -g ` G ) " { .0. } ) y <-> ( x e. ( Base ` G ) /\ y = x ) ) )
57	56	opabbidv 4716	. . . . . . 7 |- ( G e. TopGrp -> { <. x , y >. | x ( `' ( -g ` G ) " { .0. } ) y } = { <. x , y >. | ( x e. ( Base ` G ) /\ y = x ) } )
58		opabresid 5455	. . . . . . 7 |- { <. x , y >. | ( x e. ( Base ` G ) /\ y = x ) } = ( _I |` ( Base ` G ) )
59	57, 58	syl6eq 2672	. . . . . 6 |- ( G e. TopGrp -> { <. x , y >. | x ( `' ( -g ` G ) " { .0. } ) y } = ( _I |` ( Base ` G ) ) )
60	9	reseq2d 5396	. . . . . 6 |- ( G e. TopGrp -> ( _I |` ( Base ` G ) ) = ( _I |` U. J ) )
61	30, 59, 60	3eqtrd 2660	. . . . 5 |- ( G e. TopGrp -> ( `' ( -g ` G ) " { .0. } ) = ( _I |` U. J ) )
62	61	eleq1d 2686	. . . 4 |- ( G e. TopGrp -> ( ( `' ( -g ` G ) " { .0. } ) e. ( Clsd ` ( J tX J ) ) <-> ( _I |` U. J ) e. ( Clsd ` ( J tX J ) ) ) )
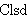
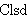63	19, 62	sylibd 229	. . 3 |- ( G e. TopGrp -> ( { .0. } e. ( Clsd ` J ) -> ( _I |` U. J ) e. ( Clsd ` ( J tX J ) ) ) )
64		topontop 20718	. . . . 5 |- ( J e. (TopOn ` ( Base ` G ) ) -> J e. Top )
65	7, 64	syl 17	. . . 4 |- ( G e. TopGrp -> J e. Top )
66	11	hausdiag 21448	. . . . 5 |- ( J e. Haus <-> ( J e. Top /\ ( _I |` U. J ) e. ( Clsd ` ( J tX J ) ) ) )
67	66	baib 944	. . . 4 |- ( J e. Top -> ( J e. Haus <-> ( _I |` U. J ) e. ( Clsd ` ( J tX J ) ) ) )
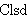68	65, 67	syl 17	. . 3 |- ( G e. TopGrp -> ( J e. Haus <-> ( _I |` U. J ) e. ( Clsd ` ( J tX J ) ) ) )
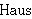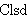69	63, 68	sylibrd 249	. 2 |- ( G e. TopGrp -> ( { .0. } e. ( Clsd ` J ) -> J e. Haus ) )
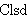
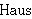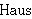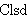70	14, 69	impbid 202	1 |- ( G e. TopGrp -> ( J e. Haus <-> { .0. } e. ( Clsd ` J ) ) )

Syntax hints:   -> wi 4   <-> wb 196   /\ wa 384   = wceq 1483   e. wcel 1990   C_ wss 3574   {csn 4177   <.cop 4183   U.cuni 4436   class class class wbr 4653   {copab 4712   _I cid 5023   X. cxp 5112   `'ccnv 5113   dom cdm 5114   |` cres 5116   "cima 5117   Rel wrel 5119   Fn wfn 5883   -->wf 5884   ` cfv 5888  (class class class)co 6650   Basecbs 15857   TopOpenctopn 16082   0gc0g 16100   Grpcgrp 17422   -gcsg 17424   Topctop 20698  TopOnctopon 20715   Clsdccld 20820   Cn ccn 21028   Hauscha 21112   tX ctx 21363   TopGrpctgp 21875

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-rep 4771  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-ral 2917  df-rex 2918  df-reu 2919  df-rmo 2920  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-op 4184  df-uni 4437  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-id 5024  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-riota 6611  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-1st 7168  df-2nd 7169  df-map 7859  df-0g 16102  df-topgen 16104  df-plusf 17241  df-mgm 17242  df-sgrp 17284  df-mnd 17295  df-grp 17425  df-minusg 17426  df-sbg 17427  df-top 20699  df-topon 20716  df-topsp 20737  df-bases 20750  df-cld 20823  df-cn 21031  df-t1 21118  df-haus 21119  df-tx 21365  df-tmd 21876  df-tgp 21877

This theorem is referenced by:  tgpt1  21921  qustgphaus  21926