Theorem isf32lem9 9183

Description: Lemma for isfin3-2 9189. Construction of the onto function. (Contributed by Stefan O'Rear, 5-Nov-2014.) (Revised by Mario Carneiro, 2-Oct-2015.)

Hypotheses

Ref	Expression
isf32lem.a	|- ( ph -> F : om --> ~P G )
isf32lem.b	|- ( ph -> A. x e. om ( F ` suc x ) C_ ( F ` x ) )
isf32lem.c	|- ( ph -> -. |^| ran F e. ran F )
isf32lem.d	|- S = { y e. om | ( F ` suc y ) C. ( F ` y ) }
isf32lem.e	|- J = ( u e. om |-> ( iota_ v e. S ( v i^i S ) ~~ u ) )
isf32lem.f	|- K = ( ( w e. S |-> ( ( F ` w ) \ ( F ` suc w ) ) ) o. J )
isf32lem.g	|- L = ( t e. G |-> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) )

Assertion

Ref	Expression
isf32lem9	|- ( ph -> L : G -onto-> om )

Distinct variable groups:   x, w   t, G   x, L   t, s, u, v, w, x, y, ph   w, F, x, y   S, s, t, u, v, w, x, y   J, s, t, w, x, y   K, s, t, x, y

Allowed substitution hints:   F( v, u, t, s)   G( x, y, w, v, u, s)   J( v, u)   K( w, v, u)   L( y, w, v, u, t, s)

Proof of Theorem isf32lem9

Dummy variables a b are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		isf32lem.g	. . . 4 |- L = ( t e. G |-> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) )
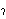
2		ssab2 3686	. . . . . . 7 |- { s | ( s e. om /\ t e. ( K ` s ) ) } C_ om
3		iotacl 5874	. . . . . . 7 |- ( E! s ( s e. om /\ t e. ( K ` s ) ) -> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) e. { s | ( s e. om /\ t e. ( K ` s ) ) } )
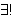
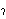
4	2, 3	sseldi 3601	. . . . . 6 |- ( E! s ( s e. om /\ t e. ( K ` s ) ) -> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) e. om )
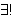
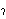
5		iotanul 5866	. . . . . . 7 |- ( -. E! s ( s e. om /\ t e. ( K ` s ) ) -> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) = (/) )
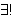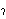
6		peano1 7085	. . . . . . 7 |- (/) e. om
7	5, 6	syl6eqel 2709	. . . . . 6 |- ( -. E! s ( s e. om /\ t e. ( K ` s ) ) -> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) e. om )
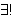
8	4, 7	pm2.61i 176	. . . . 5 |- ( iota s ( s e. om /\ t e. ( K ` s ) ) ) e. om
9	8	a1i 11	. . . 4 |- ( t e. G -> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) e. om )
10	1, 9	fmpti 6383	. . 3 |- L : G --> om
11	10	a1i 11	. 2 |- ( ph -> L : G --> om )
12		isf32lem.a	. . . . . 6 |- ( ph -> F : om --> ~P G )
13		isf32lem.b	. . . . . 6 |- ( ph -> A. x e. om ( F ` suc x ) C_ ( F ` x ) )
14		isf32lem.c	. . . . . 6 |- ( ph -> -. |^| ran F e. ran F )
15		isf32lem.d	. . . . . 6 |- S = { y e. om | ( F ` suc y ) C. ( F ` y ) }
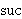16		isf32lem.e	. . . . . 6 |- J = ( u e. om |-> ( iota_ v e. S ( v i^i S ) ~~ u ) )
17		isf32lem.f	. . . . . 6 |- K = ( ( w e. S |-> ( ( F ` w ) \ ( F ` suc w ) ) ) o. J )
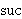18	12, 13, 14, 15, 16, 17	isf32lem6 9180	. . . . 5 |- ( ( ph /\ a e. om ) -> ( K ` a ) =/= (/) )
19		n0 3931	. . . . 5 |- ( ( K ` a ) =/= (/) <-> E. b b e. ( K ` a ) )
20	18, 19	sylib 208	. . . 4 |- ( ( ph /\ a e. om ) -> E. b b e. ( K ` a ) )
21	12, 13, 14, 15, 16, 17	isf32lem8 9182	. . . . . . . . 9 |- ( ( ph /\ a e. om ) -> ( K ` a ) C_ G )
22	21	sselda 3603	. . . . . . . 8 |- ( ( ( ph /\ a e. om ) /\ b e. ( K ` a ) ) -> b e. G )
23		eleq1 2689	. . . . . . . . . . . . 13 |- ( t = b -> ( t e. ( K ` s ) <-> b e. ( K ` s ) ) )
24	23	anbi2d 740	. . . . . . . . . . . 12 |- ( t = b -> ( ( s e. om /\ t e. ( K ` s ) ) <-> ( s e. om /\ b e. ( K ` s ) ) ) )
25	24	iotabidv 5872	. . . . . . . . . . 11 |- ( t = b -> ( iota s ( s e. om /\ t e. ( K ` s ) ) ) = ( iota s ( s e. om /\ b e. ( K ` s ) ) ) )
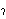
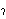26		iotaex 5868	. . . . . . . . . . 11 |- ( iota s ( s e. om /\ t e. ( K ` s ) ) ) e. _V
27	25, 1, 26	fvmpt3i 6287	. . . . . . . . . 10 |- ( b e. G -> ( L ` b ) = ( iota s ( s e. om /\ b e. ( K ` s ) ) ) )
28	22, 27	syl 17	. . . . . . . . 9 |- ( ( ( ph /\ a e. om ) /\ b e. ( K ` a ) ) -> ( L ` b ) = ( iota s ( s e. om /\ b e. ( K ` s ) ) ) )
29		simp1r 1086	. . . . . . . . . . . . . . . 16 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om /\ s e. om ) -> b e. ( K ` a ) )
30		simpl1 1064	. . . . . . . . . . . . . . . . . . . . . 22 |- ( ( ( ph /\ a e. om /\ s e. om ) /\ s =/= a ) -> ph )
31		simpr 477	. . . . . . . . . . . . . . . . . . . . . . 23 |- ( ( ( ph /\ a e. om /\ s e. om ) /\ s =/= a ) -> s =/= a )
32	31	necomd 2849	. . . . . . . . . . . . . . . . . . . . . 22 |- ( ( ( ph /\ a e. om /\ s e. om ) /\ s =/= a ) -> a =/= s )
33		simpl2 1065	. . . . . . . . . . . . . . . . . . . . . 22 |- ( ( ( ph /\ a e. om /\ s e. om ) /\ s =/= a ) -> a e. om )
34		simpl3 1066	. . . . . . . . . . . . . . . . . . . . . 22 |- ( ( ( ph /\ a e. om /\ s e. om ) /\ s =/= a ) -> s e. om )
35	12, 13, 14, 15, 16, 17	isf32lem7 9181	. . . . . . . . . . . . . . . . . . . . . 22 |- ( ( ( ph /\ a =/= s ) /\ ( a e. om /\ s e. om ) ) -> ( ( K ` a ) i^i ( K ` s ) ) = (/) )
36	30, 32, 33, 34, 35	syl22anc 1327	. . . . . . . . . . . . . . . . . . . . 21 |- ( ( ( ph /\ a e. om /\ s e. om ) /\ s =/= a ) -> ( ( K ` a ) i^i ( K ` s ) ) = (/) )
37		disj1 4019	. . . . . . . . . . . . . . . . . . . . 21 |- ( ( ( K ` a ) i^i ( K ` s ) ) = (/) <-> A. b ( b e. ( K ` a ) -> -. b e. ( K ` s ) ) )
38	36, 37	sylib 208	. . . . . . . . . . . . . . . . . . . 20 |- ( ( ( ph /\ a e. om /\ s e. om ) /\ s =/= a ) -> A. b ( b e. ( K ` a ) -> -. b e. ( K ` s ) ) )
39	38	ex 450	. . . . . . . . . . . . . . . . . . 19 |- ( ( ph /\ a e. om /\ s e. om ) -> ( s =/= a -> A. b ( b e. ( K ` a ) -> -. b e. ( K ` s ) ) ) )
40		sp 2053	. . . . . . . . . . . . . . . . . . 19 |- ( A. b ( b e. ( K ` a ) -> -. b e. ( K ` s ) ) -> ( b e. ( K ` a ) -> -. b e. ( K ` s ) ) )
41	39, 40	syl6 35	. . . . . . . . . . . . . . . . . 18 |- ( ( ph /\ a e. om /\ s e. om ) -> ( s =/= a -> ( b e. ( K ` a ) -> -. b e. ( K ` s ) ) ) )
42	41	com23 86	. . . . . . . . . . . . . . . . 17 |- ( ( ph /\ a e. om /\ s e. om ) -> ( b e. ( K ` a ) -> ( s =/= a -> -. b e. ( K ` s ) ) ) )
43	42	3adant1r 1319	. . . . . . . . . . . . . . . 16 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om /\ s e. om ) -> ( b e. ( K ` a ) -> ( s =/= a -> -. b e. ( K ` s ) ) ) )
44	29, 43	mpd 15	. . . . . . . . . . . . . . 15 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om /\ s e. om ) -> ( s =/= a -> -. b e. ( K ` s ) ) )
45	44	necon4ad 2813	. . . . . . . . . . . . . 14 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om /\ s e. om ) -> ( b e. ( K ` s ) -> s = a ) )
46	45	3expia 1267	. . . . . . . . . . . . 13 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om ) -> ( s e. om -> ( b e. ( K ` s ) -> s = a ) ) )
47	46	impd 447	. . . . . . . . . . . 12 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om ) -> ( ( s e. om /\ b e. ( K ` s ) ) -> s = a ) )
48		eleq1 2689	. . . . . . . . . . . . . . . 16 |- ( s = a -> ( s e. om <-> a e. om ) )
49		fveq2 6191	. . . . . . . . . . . . . . . . 17 |- ( s = a -> ( K ` s ) = ( K ` a ) )
50	49	eleq2d 2687	. . . . . . . . . . . . . . . 16 |- ( s = a -> ( b e. ( K ` s ) <-> b e. ( K ` a ) ) )
51	48, 50	anbi12d 747	. . . . . . . . . . . . . . 15 |- ( s = a -> ( ( s e. om /\ b e. ( K ` s ) ) <-> ( a e. om /\ b e. ( K ` a ) ) ) )
52	51	biimprcd 240	. . . . . . . . . . . . . 14 |- ( ( a e. om /\ b e. ( K ` a ) ) -> ( s = a -> ( s e. om /\ b e. ( K ` s ) ) ) )
53	52	ancoms 469	. . . . . . . . . . . . 13 |- ( ( b e. ( K ` a ) /\ a e. om ) -> ( s = a -> ( s e. om /\ b e. ( K ` s ) ) ) )
54	53	adantll 750	. . . . . . . . . . . 12 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om ) -> ( s = a -> ( s e. om /\ b e. ( K ` s ) ) ) )
55	47, 54	impbid 202	. . . . . . . . . . 11 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om ) -> ( ( s e. om /\ b e. ( K ` s ) ) <-> s = a ) )
56	55	iota5 5871	. . . . . . . . . 10 |- ( ( ( ph /\ b e. ( K ` a ) ) /\ a e. om ) -> ( iota s ( s e. om /\ b e. ( K ` s ) ) ) = a )
57	56	an32s 846	. . . . . . . . 9 |- ( ( ( ph /\ a e. om ) /\ b e. ( K ` a ) ) -> ( iota s ( s e. om /\ b e. ( K ` s ) ) ) = a )
58	28, 57	eqtr2d 2657	. . . . . . . 8 |- ( ( ( ph /\ a e. om ) /\ b e. ( K ` a ) ) -> a = ( L ` b ) )
59	22, 58	jca 554	. . . . . . 7 |- ( ( ( ph /\ a e. om ) /\ b e. ( K ` a ) ) -> ( b e. G /\ a = ( L ` b ) ) )
60	59	ex 450	. . . . . 6 |- ( ( ph /\ a e. om ) -> ( b e. ( K ` a ) -> ( b e. G /\ a = ( L ` b ) ) ) )
61	60	eximdv 1846	. . . . 5 |- ( ( ph /\ a e. om ) -> ( E. b b e. ( K ` a ) -> E. b ( b e. G /\ a = ( L ` b ) ) ) )
62		df-rex 2918	. . . . 5 |- ( E. b e. G a = ( L ` b ) <-> E. b ( b e. G /\ a = ( L ` b ) ) )
63	61, 62	syl6ibr 242	. . . 4 |- ( ( ph /\ a e. om ) -> ( E. b b e. ( K ` a ) -> E. b e. G a = ( L ` b ) ) )
64	20, 63	mpd 15	. . 3 |- ( ( ph /\ a e. om ) -> E. b e. G a = ( L ` b ) )
65	64	ralrimiva 2966	. 2 |- ( ph -> A. a e. om E. b e. G a = ( L ` b ) )
66		dffo3 6374	. 2 |- ( L : G -onto-> om <-> ( L : G --> om /\ A. a e. om E. b e. G a = ( L ` b ) ) )
67	11, 65, 66	sylanbrc 698	1 |- ( ph -> L : G -onto-> om )

Syntax hints:   -. wn 3   -> wi 4   /\ wa 384   /\ w3a 1037   A.wal 1481   = wceq 1483   E.wex 1704   e. wcel 1990   E!weu 2470   {cab 2608   =/= wne 2794   A.wral 2912   E.wrex 2913   {crab 2916   \ cdif 3571   i^i cin 3573   C_ wss 3574   C. wpss 3575   (/)c0 3915   ~Pcpw 4158   |^|cint 4475   class class class wbr 4653   |-> cmpt 4729   ran crn 5115   o. ccom 5118   suc csuc 5725   iotacio 5849   -->wf 5884   -onto->wfo 5886   ` cfv 5888   iota_crio 6610   omcom 7065   ~~ cen 7952

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-rep 4771  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3or 1038  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-ral 2917  df-rex 2918  df-reu 2919  df-rmo 2920  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-pss 3590  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-tp 4182  df-op 4184  df-uni 4437  df-int 4476  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-tr 4753  df-id 5024  df-eprel 5029  df-po 5035  df-so 5036  df-fr 5073  df-se 5074  df-we 5075  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-pred 5680  df-ord 5726  df-on 5727  df-lim 5728  df-suc 5729  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-isom 5897  df-riota 6611  df-om 7066  df-wrecs 7407  df-recs 7468  df-1o 7560  df-er 7742  df-en 7956  df-dom 7957  df-sdom 7958  df-fin 7959  df-card 8765

This theorem is referenced by:  isf32lem10  9184