Theorem isbnd2 33582

Description: The predicate "is a bounded metric space". Uses a single point instead of an arbitrary point in the space. (Contributed by Jeff Madsen, 2-Sep-2009.)

Assertion

Ref	Expression
isbnd2	|- ( ( M e. ( Bnd ` X ) /\ X =/= (/) ) <-> ( M e. ( *Met ` X ) /\ E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )

Distinct variable groups:   x, r, M   X, r, x

Proof of Theorem isbnd2

Dummy variables s y are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		isbndx 33581	. . 3 |- ( M e. ( Bnd ` X ) <-> ( M e. ( *Met ` X ) /\ A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )
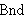
2	1	anbi1i 731	. 2 |- ( ( M e. ( Bnd ` X ) /\ X =/= (/) ) <-> ( ( M e. ( *Met ` X ) /\ A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) /\ X =/= (/) ) )
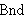
3		anass 681	. 2 |- ( ( ( M e. ( *Met ` X ) /\ A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) /\ X =/= (/) ) <-> ( M e. ( *Met ` X ) /\ ( A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) /\ X =/= (/) ) ) )
4		r19.2z 4060	. . . . 5 |- ( ( X =/= (/) /\ A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) -> E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) )
5	4	ancoms 469	. . . 4 |- ( ( A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) /\ X =/= (/) ) -> E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) )
6		oveq1 6657	. . . . . . . 8 |- ( x = y -> ( x ( ball ` M ) r ) = ( y ( ball ` M ) r ) )
7	6	eqeq2d 2632	. . . . . . 7 |- ( x = y -> ( X = ( x ( ball ` M ) r ) <-> X = ( y ( ball ` M ) r ) ) )
8		oveq2 6658	. . . . . . . 8 |- ( r = s -> ( y ( ball ` M ) r ) = ( y ( ball ` M ) s ) )
9	8	eqeq2d 2632	. . . . . . 7 |- ( r = s -> ( X = ( y ( ball ` M ) r ) <-> X = ( y ( ball ` M ) s ) ) )
10	7, 9	cbvrex2v 3180	. . . . . 6 |- ( E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) <-> E. y e. X E. s e. RR+ X = ( y ( ball ` M ) s ) )
11		2rp 11837	. . . . . . . . . . . . 13 |- 2 e. RR+
12		rpmulcl 11855	. . . . . . . . . . . . 13 |- ( ( 2 e. RR+ /\ s e. RR+ ) -> ( 2 x. s ) e. RR+ )
13	11, 12	mpan 706	. . . . . . . . . . . 12 |- ( s e. RR+ -> ( 2 x. s ) e. RR+ )
14	13	ad2antll 765	. . . . . . . . . . 11 |- ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) -> ( 2 x. s ) e. RR+ )
15	14	ad2antrr 762	. . . . . . . . . 10 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) s ) ) -> ( 2 x. s ) e. RR+ )
16		rpcn 11841	. . . . . . . . . . . . . . . . . . 19 |- ( s e. RR+ -> s e. CC )
17		2cnd 11093	. . . . . . . . . . . . . . . . . . 19 |- ( s e. RR+ -> 2 e. CC )
18		2ne0 11113	. . . . . . . . . . . . . . . . . . . 20 |- 2 =/= 0
19	18	a1i 11	. . . . . . . . . . . . . . . . . . 19 |- ( s e. RR+ -> 2 =/= 0 )
20		divcan3 10711	. . . . . . . . . . . . . . . . . . . 20 |- ( ( s e. CC /\ 2 e. CC /\ 2 =/= 0 ) -> ( ( 2 x. s ) / 2 ) = s )
21	20	eqcomd 2628	. . . . . . . . . . . . . . . . . . 19 |- ( ( s e. CC /\ 2 e. CC /\ 2 =/= 0 ) -> s = ( ( 2 x. s ) / 2 ) )
22	16, 17, 19, 21	syl3anc 1326	. . . . . . . . . . . . . . . . . 18 |- ( s e. RR+ -> s = ( ( 2 x. s ) / 2 ) )
23	22	oveq2d 6666	. . . . . . . . . . . . . . . . 17 |- ( s e. RR+ -> ( y ( ball ` M ) s ) = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) )
24	23	eqeq2d 2632	. . . . . . . . . . . . . . . 16 |- ( s e. RR+ -> ( X = ( y ( ball ` M ) s ) <-> X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) )
25	24	biimpd 219	. . . . . . . . . . . . . . 15 |- ( s e. RR+ -> ( X = ( y ( ball ` M ) s ) -> X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) )
26	25	ad2antll 765	. . . . . . . . . . . . . 14 |- ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) -> ( X = ( y ( ball ` M ) s ) -> X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) )
27	26	adantr 481	. . . . . . . . . . . . 13 |- ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) -> ( X = ( y ( ball ` M ) s ) -> X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) )
28	27	imp 445	. . . . . . . . . . . 12 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) s ) ) -> X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) )
29		simpr 477	. . . . . . . . . . . . 13 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) -> X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) )
30		eleq2 2690	. . . . . . . . . . . . . . . 16 |- ( X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) -> ( x e. X <-> x e. ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) )
31	30	biimpac 503	. . . . . . . . . . . . . . 15 |- ( ( x e. X /\ X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) -> x e. ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) )
32		2re 11090	. . . . . . . . . . . . . . . . . . 19 |- 2 e. RR
33		rpre 11839	. . . . . . . . . . . . . . . . . . 19 |- ( s e. RR+ -> s e. RR )
34		remulcl 10021	. . . . . . . . . . . . . . . . . . 19 |- ( ( 2 e. RR /\ s e. RR ) -> ( 2 x. s ) e. RR )
35	32, 33, 34	sylancr 695	. . . . . . . . . . . . . . . . . 18 |- ( s e. RR+ -> ( 2 x. s ) e. RR )
36		blhalf 22210	. . . . . . . . . . . . . . . . . . 19 |- ( ( ( M e. ( *Met ` X ) /\ y e. X ) /\ ( ( 2 x. s ) e. RR /\ x e. ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) ) -> ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) C_ ( x ( ball ` M ) ( 2 x. s ) ) )
37	36	expr 643	. . . . . . . . . . . . . . . . . 18 |- ( ( ( M e. ( *Met ` X ) /\ y e. X ) /\ ( 2 x. s ) e. RR ) -> ( x e. ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) -> ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) C_ ( x ( ball ` M ) ( 2 x. s ) ) ) )
38	35, 37	sylan2 491	. . . . . . . . . . . . . . . . 17 |- ( ( ( M e. ( *Met ` X ) /\ y e. X ) /\ s e. RR+ ) -> ( x e. ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) -> ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) C_ ( x ( ball ` M ) ( 2 x. s ) ) ) )
39	38	anasss 679	. . . . . . . . . . . . . . . 16 |- ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) -> ( x e. ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) -> ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) C_ ( x ( ball ` M ) ( 2 x. s ) ) ) )
40	39	imp 445	. . . . . . . . . . . . . . 15 |- ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) -> ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) C_ ( x ( ball ` M ) ( 2 x. s ) ) )
41	31, 40	sylan2 491	. . . . . . . . . . . . . 14 |- ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ ( x e. X /\ X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) ) -> ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) C_ ( x ( ball ` M ) ( 2 x. s ) ) )
42	41	anassrs 680	. . . . . . . . . . . . 13 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) -> ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) C_ ( x ( ball ` M ) ( 2 x. s ) ) )
43	29, 42	eqsstrd 3639	. . . . . . . . . . . 12 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) ( ( 2 x. s ) / 2 ) ) ) -> X C_ ( x ( ball ` M ) ( 2 x. s ) ) )
44	28, 43	syldan 487	. . . . . . . . . . 11 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) s ) ) -> X C_ ( x ( ball ` M ) ( 2 x. s ) ) )
45	13	adantl 482	. . . . . . . . . . . . . 14 |- ( ( y e. X /\ s e. RR+ ) -> ( 2 x. s ) e. RR+ )
46		rpxr 11840	. . . . . . . . . . . . . . . 16 |- ( ( 2 x. s ) e. RR+ -> ( 2 x. s ) e. RR* )
47		blssm 22223	. . . . . . . . . . . . . . . 16 |- ( ( M e. ( *Met ` X ) /\ x e. X /\ ( 2 x. s ) e. RR* ) -> ( x ( ball ` M ) ( 2 x. s ) ) C_ X )
48	46, 47	syl3an3 1361	. . . . . . . . . . . . . . 15 |- ( ( M e. ( *Met ` X ) /\ x e. X /\ ( 2 x. s ) e. RR+ ) -> ( x ( ball ` M ) ( 2 x. s ) ) C_ X )
49	48	3expa 1265	. . . . . . . . . . . . . 14 |- ( ( ( M e. ( *Met ` X ) /\ x e. X ) /\ ( 2 x. s ) e. RR+ ) -> ( x ( ball ` M ) ( 2 x. s ) ) C_ X )
50	45, 49	sylan2 491	. . . . . . . . . . . . 13 |- ( ( ( M e. ( *Met ` X ) /\ x e. X ) /\ ( y e. X /\ s e. RR+ ) ) -> ( x ( ball ` M ) ( 2 x. s ) ) C_ X )
51	50	an32s 846	. . . . . . . . . . . 12 |- ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) -> ( x ( ball ` M ) ( 2 x. s ) ) C_ X )
52	51	adantr 481	. . . . . . . . . . 11 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) s ) ) -> ( x ( ball ` M ) ( 2 x. s ) ) C_ X )
53	44, 52	eqssd 3620	. . . . . . . . . 10 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) s ) ) -> X = ( x ( ball ` M ) ( 2 x. s ) ) )
54		oveq2 6658	. . . . . . . . . . . 12 |- ( r = ( 2 x. s ) -> ( x ( ball ` M ) r ) = ( x ( ball ` M ) ( 2 x. s ) ) )
55	54	eqeq2d 2632	. . . . . . . . . . 11 |- ( r = ( 2 x. s ) -> ( X = ( x ( ball ` M ) r ) <-> X = ( x ( ball ` M ) ( 2 x. s ) ) ) )
56	55	rspcev 3309	. . . . . . . . . 10 |- ( ( ( 2 x. s ) e. RR+ /\ X = ( x ( ball ` M ) ( 2 x. s ) ) ) -> E. r e. RR+ X = ( x ( ball ` M ) r ) )
57	15, 53, 56	syl2anc 693	. . . . . . . . 9 |- ( ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) /\ X = ( y ( ball ` M ) s ) ) -> E. r e. RR+ X = ( x ( ball ` M ) r ) )
58	57	ex 450	. . . . . . . 8 |- ( ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) /\ x e. X ) -> ( X = ( y ( ball ` M ) s ) -> E. r e. RR+ X = ( x ( ball ` M ) r ) ) )
59	58	ralrimdva 2969	. . . . . . 7 |- ( ( M e. ( *Met ` X ) /\ ( y e. X /\ s e. RR+ ) ) -> ( X = ( y ( ball ` M ) s ) -> A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )
60	59	rexlimdvva 3038	. . . . . 6 |- ( M e. ( *Met ` X ) -> ( E. y e. X E. s e. RR+ X = ( y ( ball ` M ) s ) -> A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )
61	10, 60	syl5bi 232	. . . . 5 |- ( M e. ( *Met ` X ) -> ( E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) -> A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )
62		rexn0 4074	. . . . . 6 |- ( E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) -> X =/= (/) )
63	62	a1i 11	. . . . 5 |- ( M e. ( *Met ` X ) -> ( E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) -> X =/= (/) ) )
64	61, 63	jcad 555	. . . 4 |- ( M e. ( *Met ` X ) -> ( E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) -> ( A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) /\ X =/= (/) ) ) )
65	5, 64	impbid2 216	. . 3 |- ( M e. ( *Met ` X ) -> ( ( A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) /\ X =/= (/) ) <-> E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )
66	65	pm5.32i 669	. 2 |- ( ( M e. ( *Met ` X ) /\ ( A. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) /\ X =/= (/) ) ) <-> ( M e. ( *Met ` X ) /\ E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )
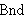
67	2, 3, 66	3bitri 286	1 |- ( ( M e. ( Bnd ` X ) /\ X =/= (/) ) <-> ( M e. ( *Met ` X ) /\ E. x e. X E. r e. RR+ X = ( x ( ball ` M ) r ) ) )

Syntax hints:   -> wi 4   <-> wb 196   /\ wa 384   /\ w3a 1037   = wceq 1483   e. wcel 1990   =/= wne 2794   A.wral 2912   E.wrex 2913   C_ wss 3574   (/)c0 3915   ` cfv 5888  (class class class)co 6650   CCcc 9934   RRcr 9935   0cc0 9936   x. cmul 9941   RR*cxr 10073   / cdiv 10684   2c2 11070   RR+crp 11832   *Metcxmt 19731   ballcbl 19733   Bndcbnd 33566

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949  ax-cnex 9992  ax-resscn 9993  ax-1cn 9994  ax-icn 9995  ax-addcl 9996  ax-addrcl 9997  ax-mulcl 9998  ax-mulrcl 9999  ax-mulcom 10000  ax-addass 10001  ax-mulass 10002  ax-distr 10003  ax-i2m1 10004  ax-1ne0 10005  ax-1rid 10006  ax-rnegex 10007  ax-rrecex 10008  ax-cnre 10009  ax-pre-lttri 10010  ax-pre-lttrn 10011  ax-pre-ltadd 10012  ax-pre-mulgt0 10013

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3or 1038  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-nel 2898  df-ral 2917  df-rex 2918  df-reu 2919  df-rmo 2920  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-op 4184  df-uni 4437  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-id 5024  df-po 5035  df-so 5036  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-riota 6611  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-1st 7168  df-2nd 7169  df-er 7742  df-ec 7744  df-map 7859  df-en 7956  df-dom 7957  df-sdom 7958  df-pnf 10076  df-mnf 10077  df-xr 10078  df-ltxr 10079  df-le 10080  df-sub 10268  df-neg 10269  df-div 10685  df-2 11079  df-rp 11833  df-xneg 11946  df-xadd 11947  df-xmul 11948  df-psmet 19738  df-xmet 19739  df-met 19740  df-bl 19741  df-bnd 33578

This theorem is referenced by:  isbnd3  33583  blbnd  33586  ssbnd  33587