Theorem fmul01lt1lem1 39816

Description: Given a finite multiplication of values betweeen 0 and 1, a value larger than its frist element is larger the whole multiplication. (Contributed by Glauco Siliprandi, 20-Apr-2017.)

Hypotheses

Ref	Expression
fmul01lt1lem1.1	|- F/_ i B
fmul01lt1lem1.2	|- F/ i ph
fmul01lt1lem1.3	|- A = seq L ( x. , B )
fmul01lt1lem1.4	|- ( ph -> L e. ZZ )
fmul01lt1lem1.5	|- ( ph -> M e. ( ZZ>= ` L ) )
fmul01lt1lem1.6	|- ( ( ph /\ i e. ( L ... M ) ) -> ( B ` i ) e. RR )
fmul01lt1lem1.7	|- ( ( ph /\ i e. ( L ... M ) ) -> 0 <_ ( B ` i ) )
fmul01lt1lem1.8	|- ( ( ph /\ i e. ( L ... M ) ) -> ( B ` i ) <_ 1 )
fmul01lt1lem1.9	|- ( ph -> E e. RR+ )
fmul01lt1lem1.10	|- ( ph -> ( B ` L ) < E )

Assertion

Ref	Expression
fmul01lt1lem1	|- ( ph -> ( A ` M ) < E )

Distinct variable groups:   i, L   i, M

Allowed substitution hints:   ph( i)   A( i)   B( i)   E( i)

Proof of Theorem fmul01lt1lem1

Dummy variables j k l are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		simpr 477	. . . . 5 |- ( ( ph /\ M = L ) -> M = L )
2	1	fveq2d 6195	. . . 4 |- ( ( ph /\ M = L ) -> ( A ` M ) = ( A ` L ) )
3		fmul01lt1lem1.3	. . . . . 6 |- A = seq L ( x. , B )
4	3	a1i 11	. . . . 5 |- ( ( ph /\ M = L ) -> A = seq L ( x. , B ) )
5	4	fveq1d 6193	. . . 4 |- ( ( ph /\ M = L ) -> ( A ` L ) = ( seq L ( x. , B ) ` L ) )
6		fmul01lt1lem1.4	. . . . . 6 |- ( ph -> L e. ZZ )
7		seq1 12814	. . . . . 6 |- ( L e. ZZ -> ( seq L ( x. , B ) ` L ) = ( B ` L ) )
8	6, 7	syl 17	. . . . 5 |- ( ph -> ( seq L ( x. , B ) ` L ) = ( B ` L ) )
9	8	adantr 481	. . . 4 |- ( ( ph /\ M = L ) -> ( seq L ( x. , B ) ` L ) = ( B ` L ) )
10	2, 5, 9	3eqtrd 2660	. . 3 |- ( ( ph /\ M = L ) -> ( A ` M ) = ( B ` L ) )
11		fmul01lt1lem1.10	. . . 4 |- ( ph -> ( B ` L ) < E )
12	11	adantr 481	. . 3 |- ( ( ph /\ M = L ) -> ( B ` L ) < E )
13	10, 12	eqbrtrd 4675	. 2 |- ( ( ph /\ M = L ) -> ( A ` M ) < E )
14		simpr 477	. . . . 5 |- ( ( ph /\ -. M = L ) -> -. M = L )
15	14	neqned 2801	. . . 4 |- ( ( ph /\ -. M = L ) -> M =/= L )
16	6	zred 11482	. . . . . . 7 |- ( ph -> L e. RR )
17		fmul01lt1lem1.5	. . . . . . . . 9 |- ( ph -> M e. ( ZZ>= ` L ) )
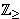18		eluzelz 11697	. . . . . . . . 9 |- ( M e. ( ZZ>= ` L ) -> M e. ZZ )
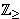
19	17, 18	syl 17	. . . . . . . 8 |- ( ph -> M e. ZZ )
20	19	zred 11482	. . . . . . 7 |- ( ph -> M e. RR )
21		eluzle 11700	. . . . . . . 8 |- ( M e. ( ZZ>= ` L ) -> L <_ M )
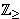
22	17, 21	syl 17	. . . . . . 7 |- ( ph -> L <_ M )
23	16, 20, 22	3jca 1242	. . . . . 6 |- ( ph -> ( L e. RR /\ M e. RR /\ L <_ M ) )
24	23	adantr 481	. . . . 5 |- ( ( ph /\ -. M = L ) -> ( L e. RR /\ M e. RR /\ L <_ M ) )
25		leltne 10127	. . . . 5 |- ( ( L e. RR /\ M e. RR /\ L <_ M ) -> ( L < M <-> M =/= L ) )
26	24, 25	syl 17	. . . 4 |- ( ( ph /\ -. M = L ) -> ( L < M <-> M =/= L ) )
27	15, 26	mpbird 247	. . 3 |- ( ( ph /\ -. M = L ) -> L < M )
28	3	fveq1i 6192	. . . 4 |- ( A ` M ) = ( seq L ( x. , B ) ` M )
29		remulcl 10021	. . . . . . 7 |- ( ( j e. RR /\ k e. RR ) -> ( j x. k ) e. RR )
30	29	adantl 482	. . . . . 6 |- ( ( ( ph /\ L < M ) /\ ( j e. RR /\ k e. RR ) ) -> ( j x. k ) e. RR )
31		recn 10026	. . . . . . . . 9 |- ( j e. RR -> j e. CC )
32	31	3ad2ant1 1082	. . . . . . . 8 |- ( ( j e. RR /\ k e. RR /\ l e. RR ) -> j e. CC )
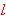33		recn 10026	. . . . . . . . 9 |- ( k e. RR -> k e. CC )
34	33	3ad2ant2 1083	. . . . . . . 8 |- ( ( j e. RR /\ k e. RR /\ l e. RR ) -> k e. CC )
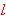35		recn 10026	. . . . . . . . 9 |- ( l e. RR -> l e. CC )
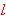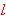36	35	3ad2ant3 1084	. . . . . . . 8 |- ( ( j e. RR /\ k e. RR /\ l e. RR ) -> l e. CC )
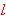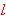37	32, 34, 36	mulassd 10063	. . . . . . 7 |- ( ( j e. RR /\ k e. RR /\ l e. RR ) -> ( ( j x. k ) x. l ) = ( j x. ( k x. l ) ) )
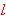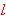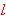38	37	adantl 482	. . . . . 6 |- ( ( ( ph /\ L < M ) /\ ( j e. RR /\ k e. RR /\ l e. RR ) ) -> ( ( j x. k ) x. l ) = ( j x. ( k x. l ) ) )
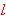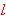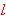39		simpr 477	. . . . . . . . . 10 |- ( ( ph /\ L < M ) -> L < M )
40	39	olcd 408	. . . . . . . . 9 |- ( ( ph /\ L < M ) -> ( M < L \/ L < M ) )
41	20, 16	jca 554	. . . . . . . . . . 11 |- ( ph -> ( M e. RR /\ L e. RR ) )
42	41	adantr 481	. . . . . . . . . 10 |- ( ( ph /\ L < M ) -> ( M e. RR /\ L e. RR ) )
43		lttri2 10120	. . . . . . . . . 10 |- ( ( M e. RR /\ L e. RR ) -> ( M =/= L <-> ( M < L \/ L < M ) ) )
44	42, 43	syl 17	. . . . . . . . 9 |- ( ( ph /\ L < M ) -> ( M =/= L <-> ( M < L \/ L < M ) ) )
45	40, 44	mpbird 247	. . . . . . . 8 |- ( ( ph /\ L < M ) -> M =/= L )
46	45	neneqd 2799	. . . . . . 7 |- ( ( ph /\ L < M ) -> -. M = L )
47		uzp1 11721	. . . . . . . . . 10 |- ( M e. ( ZZ>= ` L ) -> ( M = L \/ M e. ( ZZ>= ` ( L + 1 ) ) ) )
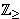
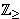48	17, 47	syl 17	. . . . . . . . 9 |- ( ph -> ( M = L \/ M e. ( ZZ>= ` ( L + 1 ) ) ) )
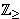49	48	adantr 481	. . . . . . . 8 |- ( ( ph /\ L < M ) -> ( M = L \/ M e. ( ZZ>= ` ( L + 1 ) ) ) )
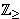50	49	ord 392	. . . . . . 7 |- ( ( ph /\ L < M ) -> ( -. M = L -> M e. ( ZZ>= ` ( L + 1 ) ) ) )
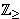
51	46, 50	mpd 15	. . . . . 6 |- ( ( ph /\ L < M ) -> M e. ( ZZ>= ` ( L + 1 ) ) )
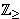52	6	adantr 481	. . . . . . 7 |- ( ( ph /\ L < M ) -> L e. ZZ )
53		uzid 11702	. . . . . . 7 |- ( L e. ZZ -> L e. ( ZZ>= ` L ) )
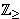54	52, 53	syl 17	. . . . . 6 |- ( ( ph /\ L < M ) -> L e. ( ZZ>= ` L ) )
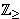55		fmul01lt1lem1.2	. . . . . . . . . 10 |- F/ i ph
56		nfv 1843	. . . . . . . . . 10 |- F/ i j e. ( L ... M )
57	55, 56	nfan 1828	. . . . . . . . 9 |- F/ i ( ph /\ j e. ( L ... M ) )
58		fmul01lt1lem1.1	. . . . . . . . . . 11 |- F/_ i B
59		nfcv 2764	. . . . . . . . . . 11 |- F/_ i j
60	58, 59	nffv 6198	. . . . . . . . . 10 |- F/_ i ( B ` j )
61	60	nfel1 2779	. . . . . . . . 9 |- F/ i ( B ` j ) e. RR
62	57, 61	nfim 1825	. . . . . . . 8 |- F/ i ( ( ph /\ j e. ( L ... M ) ) -> ( B ` j ) e. RR )
63		eleq1 2689	. . . . . . . . . 10 |- ( i = j -> ( i e. ( L ... M ) <-> j e. ( L ... M ) ) )
64	63	anbi2d 740	. . . . . . . . 9 |- ( i = j -> ( ( ph /\ i e. ( L ... M ) ) <-> ( ph /\ j e. ( L ... M ) ) ) )
65		fveq2 6191	. . . . . . . . . 10 |- ( i = j -> ( B ` i ) = ( B ` j ) )
66	65	eleq1d 2686	. . . . . . . . 9 |- ( i = j -> ( ( B ` i ) e. RR <-> ( B ` j ) e. RR ) )
67	64, 66	imbi12d 334	. . . . . . . 8 |- ( i = j -> ( ( ( ph /\ i e. ( L ... M ) ) -> ( B ` i ) e. RR ) <-> ( ( ph /\ j e. ( L ... M ) ) -> ( B ` j ) e. RR ) ) )
68		fmul01lt1lem1.6	. . . . . . . 8 |- ( ( ph /\ i e. ( L ... M ) ) -> ( B ` i ) e. RR )
69	62, 67, 68	chvar 2262	. . . . . . 7 |- ( ( ph /\ j e. ( L ... M ) ) -> ( B ` j ) e. RR )
70	69	adantlr 751	. . . . . 6 |- ( ( ( ph /\ L < M ) /\ j e. ( L ... M ) ) -> ( B ` j ) e. RR )
71	30, 38, 51, 54, 70	seqsplit 12834	. . . . 5 |- ( ( ph /\ L < M ) -> ( seq L ( x. , B ) ` M ) = ( ( seq L ( x. , B ) ` L ) x. ( seq ( L + 1 ) ( x. , B ) ` M ) ) )
72		eluzfz1 12348	. . . . . . . . . . 11 |- ( M e. ( ZZ>= ` L ) -> L e. ( L ... M ) )
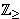
73	17, 72	syl 17	. . . . . . . . . 10 |- ( ph -> L e. ( L ... M ) )
74	73	ancli 574	. . . . . . . . . 10 |- ( ph -> ( ph /\ L e. ( L ... M ) ) )
75		nfv 1843	. . . . . . . . . . . . 13 |- F/ i L e. ( L ... M )
76	55, 75	nfan 1828	. . . . . . . . . . . 12 |- F/ i ( ph /\ L e. ( L ... M ) )
77		nfcv 2764	. . . . . . . . . . . . . 14 |- F/_ i L
78	58, 77	nffv 6198	. . . . . . . . . . . . 13 |- F/_ i ( B ` L )
79	78	nfel1 2779	. . . . . . . . . . . 12 |- F/ i ( B ` L ) e. RR
80	76, 79	nfim 1825	. . . . . . . . . . 11 |- F/ i ( ( ph /\ L e. ( L ... M ) ) -> ( B ` L ) e. RR )
81		eleq1 2689	. . . . . . . . . . . . 13 |- ( i = L -> ( i e. ( L ... M ) <-> L e. ( L ... M ) ) )
82	81	anbi2d 740	. . . . . . . . . . . 12 |- ( i = L -> ( ( ph /\ i e. ( L ... M ) ) <-> ( ph /\ L e. ( L ... M ) ) ) )
83		fveq2 6191	. . . . . . . . . . . . 13 |- ( i = L -> ( B ` i ) = ( B ` L ) )
84	83	eleq1d 2686	. . . . . . . . . . . 12 |- ( i = L -> ( ( B ` i ) e. RR <-> ( B ` L ) e. RR ) )
85	82, 84	imbi12d 334	. . . . . . . . . . 11 |- ( i = L -> ( ( ( ph /\ i e. ( L ... M ) ) -> ( B ` i ) e. RR ) <-> ( ( ph /\ L e. ( L ... M ) ) -> ( B ` L ) e. RR ) ) )
86	80, 85, 68	vtoclg1f 3265	. . . . . . . . . 10 |- ( L e. ( L ... M ) -> ( ( ph /\ L e. ( L ... M ) ) -> ( B ` L ) e. RR ) )
87	73, 74, 86	sylc 65	. . . . . . . . 9 |- ( ph -> ( B ` L ) e. RR )
88	8, 87	eqeltrd 2701	. . . . . . . 8 |- ( ph -> ( seq L ( x. , B ) ` L ) e. RR )
89	88	adantr 481	. . . . . . 7 |- ( ( ph /\ L < M ) -> ( seq L ( x. , B ) ` L ) e. RR )
90	6	adantr 481	. . . . . . . . . . . 12 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> L e. ZZ )
91	19	adantr 481	. . . . . . . . . . . 12 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> M e. ZZ )
92		elfzelz 12342	. . . . . . . . . . . . 13 |- ( j e. ( ( L + 1 ) ... M ) -> j e. ZZ )
93	92	adantl 482	. . . . . . . . . . . 12 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> j e. ZZ )
94	90, 91, 93	3jca 1242	. . . . . . . . . . 11 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> ( L e. ZZ /\ M e. ZZ /\ j e. ZZ ) )
95	16	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> L e. RR )
96		peano2re 10209	. . . . . . . . . . . . . . 15 |- ( L e. RR -> ( L + 1 ) e. RR )
97	16, 96	syl 17	. . . . . . . . . . . . . 14 |- ( ph -> ( L + 1 ) e. RR )
98	97	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> ( L + 1 ) e. RR )
99	92	zred 11482	. . . . . . . . . . . . . 14 |- ( j e. ( ( L + 1 ) ... M ) -> j e. RR )
100	99	adantl 482	. . . . . . . . . . . . 13 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> j e. RR )
101	16	lep1d 10955	. . . . . . . . . . . . . 14 |- ( ph -> L <_ ( L + 1 ) )
102	101	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> L <_ ( L + 1 ) )
103		elfzle1 12344	. . . . . . . . . . . . . 14 |- ( j e. ( ( L + 1 ) ... M ) -> ( L + 1 ) <_ j )
104	103	adantl 482	. . . . . . . . . . . . 13 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> ( L + 1 ) <_ j )
105	95, 98, 100, 102, 104	letrd 10194	. . . . . . . . . . . 12 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> L <_ j )
106		elfzle2 12345	. . . . . . . . . . . . 13 |- ( j e. ( ( L + 1 ) ... M ) -> j <_ M )
107	106	adantl 482	. . . . . . . . . . . 12 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> j <_ M )
108	105, 107	jca 554	. . . . . . . . . . 11 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> ( L <_ j /\ j <_ M ) )
109		elfz2 12333	. . . . . . . . . . 11 |- ( j e. ( L ... M ) <-> ( ( L e. ZZ /\ M e. ZZ /\ j e. ZZ ) /\ ( L <_ j /\ j <_ M ) ) )
110	94, 108, 109	sylanbrc 698	. . . . . . . . . 10 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> j e. ( L ... M ) )
111	110, 69	syldan 487	. . . . . . . . 9 |- ( ( ph /\ j e. ( ( L + 1 ) ... M ) ) -> ( B ` j ) e. RR )
112	111	adantlr 751	. . . . . . . 8 |- ( ( ( ph /\ L < M ) /\ j e. ( ( L + 1 ) ... M ) ) -> ( B ` j ) e. RR )
113	51, 112, 30	seqcl 12821	. . . . . . 7 |- ( ( ph /\ L < M ) -> ( seq ( L + 1 ) ( x. , B ) ` M ) e. RR )
114	89, 113	remulcld 10070	. . . . . 6 |- ( ( ph /\ L < M ) -> ( ( seq L ( x. , B ) ` L ) x. ( seq ( L + 1 ) ( x. , B ) ` M ) ) e. RR )
115		fmul01lt1lem1.9	. . . . . . . 8 |- ( ph -> E e. RR+ )
116	115	rpred 11872	. . . . . . 7 |- ( ph -> E e. RR )
117	116	adantr 481	. . . . . 6 |- ( ( ph /\ L < M ) -> E e. RR )
118		1red 10055	. . . . . . . 8 |- ( ( ph /\ L < M ) -> 1 e. RR )
119		nfcv 2764	. . . . . . . . . . . . . 14 |- F/_ i 0
120		nfcv 2764	. . . . . . . . . . . . . 14 |- F/_ i <_
121	119, 120, 78	nfbr 4699	. . . . . . . . . . . . 13 |- F/ i 0 <_ ( B ` L )
122	76, 121	nfim 1825	. . . . . . . . . . . 12 |- F/ i ( ( ph /\ L e. ( L ... M ) ) -> 0 <_ ( B ` L ) )
123	83	breq2d 4665	. . . . . . . . . . . . 13 |- ( i = L -> ( 0 <_ ( B ` i ) <-> 0 <_ ( B ` L ) ) )
124	82, 123	imbi12d 334	. . . . . . . . . . . 12 |- ( i = L -> ( ( ( ph /\ i e. ( L ... M ) ) -> 0 <_ ( B ` i ) ) <-> ( ( ph /\ L e. ( L ... M ) ) -> 0 <_ ( B ` L ) ) ) )
125		fmul01lt1lem1.7	. . . . . . . . . . . 12 |- ( ( ph /\ i e. ( L ... M ) ) -> 0 <_ ( B ` i ) )
126	122, 124, 125	vtoclg1f 3265	. . . . . . . . . . 11 |- ( L e. ( L ... M ) -> ( ( ph /\ L e. ( L ... M ) ) -> 0 <_ ( B ` L ) ) )
127	73, 74, 126	sylc 65	. . . . . . . . . 10 |- ( ph -> 0 <_ ( B ` L ) )
128	127, 8	breqtrrd 4681	. . . . . . . . 9 |- ( ph -> 0 <_ ( seq L ( x. , B ) ` L ) )
129	128	adantr 481	. . . . . . . 8 |- ( ( ph /\ L < M ) -> 0 <_ ( seq L ( x. , B ) ` L ) )
130		nfv 1843	. . . . . . . . . . 11 |- F/ i L < M
131	55, 130	nfan 1828	. . . . . . . . . 10 |- F/ i ( ph /\ L < M )
132		eqid 2622	. . . . . . . . . 10 |- seq ( L + 1 ) ( x. , B ) = seq ( L + 1 ) ( x. , B )
133	6	peano2zd 11485	. . . . . . . . . . 11 |- ( ph -> ( L + 1 ) e. ZZ )
134	133	adantr 481	. . . . . . . . . 10 |- ( ( ph /\ L < M ) -> ( L + 1 ) e. ZZ )
135	16	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ L < M ) -> L e. RR )
136	135, 39	gtned 10172	. . . . . . . . . . . 12 |- ( ( ph /\ L < M ) -> M =/= L )
137	136	neneqd 2799	. . . . . . . . . . 11 |- ( ( ph /\ L < M ) -> -. M = L )
138	17	adantr 481	. . . . . . . . . . . 12 |- ( ( ph /\ L < M ) -> M e. ( ZZ>= ` L ) )
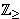139	138, 47	syl 17	. . . . . . . . . . 11 |- ( ( ph /\ L < M ) -> ( M = L \/ M e. ( ZZ>= ` ( L + 1 ) ) ) )
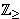140		orel1 397	. . . . . . . . . . 11 |- ( -. M = L -> ( ( M = L \/ M e. ( ZZ>= ` ( L + 1 ) ) ) -> M e. ( ZZ>= ` ( L + 1 ) ) ) )
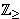
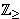141	137, 139, 140	sylc 65	. . . . . . . . . 10 |- ( ( ph /\ L < M ) -> M e. ( ZZ>= ` ( L + 1 ) ) )
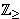142	19	adantr 481	. . . . . . . . . . . 12 |- ( ( ph /\ L < M ) -> M e. ZZ )
143	134, 142, 142	3jca 1242	. . . . . . . . . . 11 |- ( ( ph /\ L < M ) -> ( ( L + 1 ) e. ZZ /\ M e. ZZ /\ M e. ZZ ) )
144		zltp1le 11427	. . . . . . . . . . . . . 14 |- ( ( L e. ZZ /\ M e. ZZ ) -> ( L < M <-> ( L + 1 ) <_ M ) )
145	52, 142, 144	syl2anc 693	. . . . . . . . . . . . 13 |- ( ( ph /\ L < M ) -> ( L < M <-> ( L + 1 ) <_ M ) )
146	39, 145	mpbid 222	. . . . . . . . . . . 12 |- ( ( ph /\ L < M ) -> ( L + 1 ) <_ M )
147	20	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ L < M ) -> M e. RR )
148	147	leidd 10594	. . . . . . . . . . . 12 |- ( ( ph /\ L < M ) -> M <_ M )
149	146, 148	jca 554	. . . . . . . . . . 11 |- ( ( ph /\ L < M ) -> ( ( L + 1 ) <_ M /\ M <_ M ) )
150		elfz2 12333	. . . . . . . . . . 11 |- ( M e. ( ( L + 1 ) ... M ) <-> ( ( ( L + 1 ) e. ZZ /\ M e. ZZ /\ M e. ZZ ) /\ ( ( L + 1 ) <_ M /\ M <_ M ) ) )
151	143, 149, 150	sylanbrc 698	. . . . . . . . . 10 |- ( ( ph /\ L < M ) -> M e. ( ( L + 1 ) ... M ) )
152	6	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> L e. ZZ )
153	19	adantr 481	. . . . . . . . . . . . . 14 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> M e. ZZ )
154		elfzelz 12342	. . . . . . . . . . . . . . 15 |- ( i e. ( ( L + 1 ) ... M ) -> i e. ZZ )
155	154	adantl 482	. . . . . . . . . . . . . 14 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> i e. ZZ )
156	152, 153, 155	3jca 1242	. . . . . . . . . . . . 13 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> ( L e. ZZ /\ M e. ZZ /\ i e. ZZ ) )
157	16	adantr 481	. . . . . . . . . . . . . . 15 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> L e. RR )
158	157, 96	syl 17	. . . . . . . . . . . . . . 15 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> ( L + 1 ) e. RR )
159	154	zred 11482	. . . . . . . . . . . . . . . 16 |- ( i e. ( ( L + 1 ) ... M ) -> i e. RR )
160	159	adantl 482	. . . . . . . . . . . . . . 15 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> i e. RR )
161	101	adantr 481	. . . . . . . . . . . . . . 15 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> L <_ ( L + 1 ) )
162		elfzle1 12344	. . . . . . . . . . . . . . . 16 |- ( i e. ( ( L + 1 ) ... M ) -> ( L + 1 ) <_ i )
163	162	adantl 482	. . . . . . . . . . . . . . 15 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> ( L + 1 ) <_ i )
164	157, 158, 160, 161, 163	letrd 10194	. . . . . . . . . . . . . 14 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> L <_ i )
165		elfzle2 12345	. . . . . . . . . . . . . . 15 |- ( i e. ( ( L + 1 ) ... M ) -> i <_ M )
166	165	adantl 482	. . . . . . . . . . . . . 14 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> i <_ M )
167	164, 166	jca 554	. . . . . . . . . . . . 13 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> ( L <_ i /\ i <_ M ) )
168		elfz2 12333	. . . . . . . . . . . . 13 |- ( i e. ( L ... M ) <-> ( ( L e. ZZ /\ M e. ZZ /\ i e. ZZ ) /\ ( L <_ i /\ i <_ M ) ) )
169	156, 167, 168	sylanbrc 698	. . . . . . . . . . . 12 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> i e. ( L ... M ) )
170	169, 68	syldan 487	. . . . . . . . . . 11 |- ( ( ph /\ i e. ( ( L + 1 ) ... M ) ) -> ( B ` i ) e. RR )
171	170	adantlr 751	. . . . . . . . . 10 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> ( B ` i ) e. RR )
172		simpll 790	. . . . . . . . . . 11 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> ph )
173	6	ad2antrr 762	. . . . . . . . . . . . 13 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> L e. ZZ )
174	19	ad2antrr 762	. . . . . . . . . . . . 13 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> M e. ZZ )
175	154	adantl 482	. . . . . . . . . . . . 13 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> i e. ZZ )
176	173, 174, 175	3jca 1242	. . . . . . . . . . . 12 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> ( L e. ZZ /\ M e. ZZ /\ i e. ZZ ) )
177	16	ad2antrr 762	. . . . . . . . . . . . . 14 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> L e. RR )
178	97	ad2antrr 762	. . . . . . . . . . . . . 14 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> ( L + 1 ) e. RR )
179	159	adantl 482	. . . . . . . . . . . . . 14 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> i e. RR )
180	101	ad2antrr 762	. . . . . . . . . . . . . 14 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> L <_ ( L + 1 ) )
181	162	adantl 482	. . . . . . . . . . . . . 14 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> ( L + 1 ) <_ i )
182	177, 178, 179, 180, 181	letrd 10194	. . . . . . . . . . . . 13 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> L <_ i )
183	165	adantl 482	. . . . . . . . . . . . 13 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> i <_ M )
184	182, 183	jca 554	. . . . . . . . . . . 12 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> ( L <_ i /\ i <_ M ) )
185	176, 184, 168	sylanbrc 698	. . . . . . . . . . 11 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> i e. ( L ... M ) )
186	172, 185, 125	syl2anc 693	. . . . . . . . . 10 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> 0 <_ ( B ` i ) )
187		fmul01lt1lem1.8	. . . . . . . . . . 11 |- ( ( ph /\ i e. ( L ... M ) ) -> ( B ` i ) <_ 1 )
188	172, 185, 187	syl2anc 693	. . . . . . . . . 10 |- ( ( ( ph /\ L < M ) /\ i e. ( ( L + 1 ) ... M ) ) -> ( B ` i ) <_ 1 )
189	58, 131, 132, 134, 141, 151, 171, 186, 188	fmul01 39812	. . . . . . . . 9 |- ( ( ph /\ L < M ) -> ( 0 <_ ( seq ( L + 1 ) ( x. , B ) ` M ) /\ ( seq ( L + 1 ) ( x. , B ) ` M ) <_ 1 ) )
190	189	simprd 479	. . . . . . . 8 |- ( ( ph /\ L < M ) -> ( seq ( L + 1 ) ( x. , B ) ` M ) <_ 1 )
191	113, 118, 89, 129, 190	lemul2ad 10964	. . . . . . 7 |- ( ( ph /\ L < M ) -> ( ( seq L ( x. , B ) ` L ) x. ( seq ( L + 1 ) ( x. , B ) ` M ) ) <_ ( ( seq L ( x. , B ) ` L ) x. 1 ) )
192	88	recnd 10068	. . . . . . . . 9 |- ( ph -> ( seq L ( x. , B ) ` L ) e. CC )
193	192	mulid1d 10057	. . . . . . . 8 |- ( ph -> ( ( seq L ( x. , B ) ` L ) x. 1 ) = ( seq L ( x. , B ) ` L ) )
194	193	adantr 481	. . . . . . 7 |- ( ( ph /\ L < M ) -> ( ( seq L ( x. , B ) ` L ) x. 1 ) = ( seq L ( x. , B ) ` L ) )
195	191, 194	breqtrd 4679	. . . . . 6 |- ( ( ph /\ L < M ) -> ( ( seq L ( x. , B ) ` L ) x. ( seq ( L + 1 ) ( x. , B ) ` M ) ) <_ ( seq L ( x. , B ) ` L ) )
196	8, 11	eqbrtrd 4675	. . . . . . 7 |- ( ph -> ( seq L ( x. , B ) ` L ) < E )
197	196	adantr 481	. . . . . 6 |- ( ( ph /\ L < M ) -> ( seq L ( x. , B ) ` L ) < E )
198	114, 89, 117, 195, 197	lelttrd 10195	. . . . 5 |- ( ( ph /\ L < M ) -> ( ( seq L ( x. , B ) ` L ) x. ( seq ( L + 1 ) ( x. , B ) ` M ) ) < E )
199	71, 198	eqbrtrd 4675	. . . 4 |- ( ( ph /\ L < M ) -> ( seq L ( x. , B ) ` M ) < E )
200	28, 199	syl5eqbr 4688	. . 3 |- ( ( ph /\ L < M ) -> ( A ` M ) < E )
201	27, 200	syldan 487	. 2 |- ( ( ph /\ -. M = L ) -> ( A ` M ) < E )
202	13, 201	pm2.61dan 832	1 |- ( ph -> ( A ` M ) < E )

Syntax hints:   -. wn 3   -> wi 4   <-> wb 196   \/ wo 383   /\ wa 384   /\ w3a 1037   = wceq 1483   F/wnf 1708   e. wcel 1990   F/_wnfc 2751   =/= wne 2794   class class class wbr 4653   ` cfv 5888  (class class class)co 6650   CCcc 9934   RRcr 9935   0cc0 9936   1c1 9937   + caddc 9939   x. cmul 9941   < clt 10074   <_ cle 10075   ZZcz 11377   ZZ>=cuz 11687   RR+crp 11832   ...cfz 12326   seqcseq 12801

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949  ax-cnex 9992  ax-resscn 9993  ax-1cn 9994  ax-icn 9995  ax-addcl 9996  ax-addrcl 9997  ax-mulcl 9998  ax-mulrcl 9999  ax-mulcom 10000  ax-addass 10001  ax-mulass 10002  ax-distr 10003  ax-i2m1 10004  ax-1ne0 10005  ax-1rid 10006  ax-rnegex 10007  ax-rrecex 10008  ax-cnre 10009  ax-pre-lttri 10010  ax-pre-lttrn 10011  ax-pre-ltadd 10012  ax-pre-mulgt0 10013

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3or 1038  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-nel 2898  df-ral 2917  df-rex 2918  df-reu 2919  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-pss 3590  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-tp 4182  df-op 4184  df-uni 4437  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-tr 4753  df-id 5024  df-eprel 5029  df-po 5035  df-so 5036  df-fr 5073  df-we 5075  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-pred 5680  df-ord 5726  df-on 5727  df-lim 5728  df-suc 5729  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-riota 6611  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-om 7066  df-1st 7168  df-2nd 7169  df-wrecs 7407  df-recs 7468  df-rdg 7506  df-er 7742  df-en 7956  df-dom 7957  df-sdom 7958  df-pnf 10076  df-mnf 10077  df-xr 10078  df-ltxr 10079  df-le 10080  df-sub 10268  df-neg 10269  df-nn 11021  df-n0 11293  df-z 11378  df-uz 11688  df-rp 11833  df-fz 12327  df-fzo 12466  df-seq 12802

This theorem is referenced by:  fmul01lt1lem2  39817