| Step | Hyp | Ref
| Expression |
|---|
| 1 | | elfvex 6221 |
. . . 4
     mzPoly mzPoly   
    |
| 2 | 1 | adantl 482 |
. . 3
    
   mzPoly mzPoly         |
| 3 | | mzpval 37295 |
. . . . . . 7
       mzPoly mzPoly      mzPolyCld mzPolyCld    |
| 4 | 3 | adantl 482 |
. . . . . 6
    
   
  mzPoly mzPoly      mzPolyCld mzPolyCld    |
| 5 | | ssrab2 3687 |
. . . . . . . . . 10
                  
       |
| 6 | 5 | a1i 11 |
. . . . . . . . 9
    
   
                  
        |
| 7 | | ovex 6678 |
. . . . . . . . . . . . . . 15
        |
| 8 | | zex 11386 |
. . . . . . . . . . . . . . 15
    |
| 9 | 7, 8 | constmap 37276 |
. . . . . . . . . . . . . 14
                            |
| 10 | 9 | adantl 482 |
. . . . . . . . . . . . 13
    
   
                       |
| 11 | | mzpindd.co |
. . . . . . . . . . . . 13
    
   
   |
| 12 | | mzpindd.1 |
. . . . . . . . . . . . . 14
                  
   |
| 13 | 12 | elrab 3363 |
. . . . . . . . . . . . 13
                             
                          |
| 14 | 10, 11, 13 | sylanbrc 698 |
. . . . . . . . . . . 12
    
   
                             |
| 15 | 14 | ralrimiva 2966 |
. . . . . . . . . . 11
                                    |
| 16 | 15 | adantr 481 |
. . . . . . . . . 10
    
   
                                 |
| 17 | 8 | a1i 11 |
. . . . . . . . . . . . . . . 16
        
   
   
   
    
    |
| 18 | | simpllr 799 |
. . . . . . . . . . . . . . . 16
        
   
   
   
    
    |
| 19 | | simpr 477 |
. . . . . . . . . . . . . . . 16
        
   
   
   
    
   
    |
| 20 | | elmapg 7870 |
. . . . . . . . . . . . . . . . 17
        
                   |
| 21 | 20 | biimpa 501 |
. . . . . . . . . . . . . . . 16
             
              |
| 22 | 17, 18, 19, 21 | syl21anc 1325 |
. . . . . . . . . . . . . . 15
        
   
   
   
    
      |
| 23 | | simplr 792 |
. . . . . . . . . . . . . . 15
        
   
   
   
    
    |
| 24 | 22, 23 | ffvelrnd 6360 |
. . . . . . . . . . . . . 14
        
   
   
   
    
        |
| 25 | | eqid 2622 |
. . . . . . . . . . . . . 14
                                |
| 26 | 24, 25 | fmptd 6385 |
. . . . . . . . . . . . 13
                                        |
| 27 | 8, 7 | elmap 7886 |
. . . . . . . . . . . . 13
                           
                        |
| 28 | 26, 27 | sylibr 224 |
. . . . . . . . . . . 12
                                          |
| 29 | | mzpindd.pr |
. . . . . . . . . . . . 13
    
       |
| 30 | 29 | adantlr 751 |
. . . . . . . . . . . 12
                  |
| 31 | | mzpindd.2 |
. . . . . . . . . . . . 13
                      
   |
| 32 | 31 | elrab 3363 |
. . . . . . . . . . . 12
                                 
                              |
| 33 | 28, 30, 32 | sylanbrc 698 |
. . . . . . . . . . 11
                                                |
| 34 | 33 | ralrimiva 2966 |
. . . . . . . . . 10
    
   
    
                                |
| 35 | 16, 34 | jca 554 |
. . . . . . . . 9
    
   
    
                               
                                  |
| 36 | | zaddcl 11417 |
. . . . . . . . . . . . . . . . . . . 20
        
           |
| 37 | 36 | adantl 482 |
. . . . . . . . . . . . . . . . . . 19
                                   
        |
| 38 | | simpl 473 |
. . . . . . . . . . . . . . . . . . 19
                                  |
| 39 | | simpr 477 |
. . . . . . . . . . . . . . . . . . 19
                                  |
| 40 | 7 | a1i 11 |
. . . . . . . . . . . . . . . . . . 19
                                |
| 41 | | inidm 3822 |
. . . . . . . . . . . . . . . . . . 19
                    |
| 42 | 37, 38, 39, 40, 40, 41 | off 6912 |
. . . . . . . . . . . . . . . . . 18
                                        |
| 43 | 42 | ad2ant2r 783 |
. . . . . . . . . . . . . . . . 17
              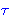              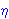   
   
            |
| 44 | 43 | adantl 482 |
. . . . . . . . . . . . . . . 16
    
            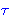              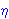    
   
            |
| 45 | | mzpindd.ad |
. . . . . . . . . . . . . . . . 17
    
           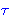 
            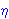    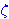  |
| 46 | 45 | 3expb 1266 |
. . . . . . . . . . . . . . . 16
    
            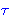              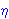    
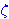  |
| 47 | 44, 46 | jca 554 |
. . . . . . . . . . . . . . 15
    
            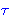              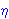    
                 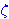   |
| 48 | | zmulcl 11426 |
. . . . . . . . . . . . . . . . . . 19
        
           |
| 49 | 48 | adantl 482 |
. . . . . . . . . . . . . . . . . 18
                                   
        |
| 50 | 49, 38, 39, 40, 40, 41 | off 6912 |
. . . . . . . . . . . . . . . . 17
                                        |
| 51 | 50 | ad2ant2r 783 |
. . . . . . . . . . . . . . . 16
              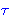              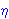   
   
            |
| 52 | 51 | adantl 482 |
. . . . . . . . . . . . . . 15
    
            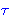              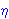    
   
            |
| 53 | | mzpindd.mu |
. . . . . . . . . . . . . . . 16
    
           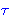 
            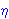    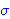  |
| 54 | 53 | 3expb 1266 |
. . . . . . . . . . . . . . 15
    
            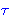              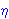    
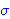  |
| 55 | 47, 52, 54 | jca32 558 |
. . . . . . . . . . . . . 14
    
            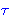              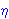    
                  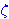                    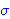    |
| 56 | 55 | ex 450 |
. . . . . . . . . . . . 13
                 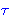 
            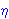                      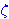                    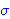     |
| 57 | 8, 7 | elmap 7886 |
. . . . . . . . . . . . . . 15
        
               |
| 58 | 57 | anbi1i 731 |
. . . . . . . . . . . . . 14
               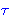 
            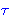   |
| 59 | 8, 7 | elmap 7886 |
. . . . . . . . . . . . . . 15
        
               |
| 60 | 59 | anbi1i 731 |
. . . . . . . . . . . . . 14
               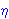 
            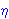   |
| 61 | 58, 60 | anbi12i 733 |
. . . . . . . . . . . . 13
                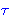                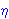                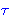              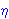    |
| 62 | 8, 7 | elmap 7886 |
. . . . . . . . . . . . . . 15
                                    |
| 63 | 62 | anbi1i 731 |
. . . . . . . . . . . . . 14
                     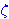  
                 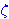   |
| 64 | 8, 7 | elmap 7886 |
. . . . . . . . . . . . . . 15
                                    |
| 65 | 64 | anbi1i 731 |
. . . . . . . . . . . . . 14
                     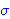  
                 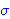   |
| 66 | 63, 65 | anbi12i 733 |
. . . . . . . . . . . . 13
                
     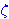                
     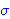                      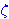                    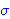    |
| 67 | 56, 61, 66 | 3imtr4g 285 |
. . . . . . . . . . . 12
             
     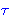                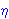                        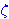                
     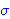     |
| 68 | | mzpindd.3 |
. . . . . . . . . . . . . 14
         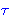   |
| 69 | 68 | elrab 3363 |
. . . . . . . . . . . . 13
                   
             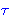   |
| 70 | | mzpindd.4 |
. . . . . . . . . . . . . 14
         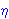   |
| 71 | 70 | elrab 3363 |
. . . . . . . . . . . . 13
                   
             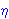   |
| 72 | 69, 71 | anbi12i 733 |
. . . . . . . . . . . 12
                      
                               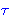                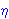    |
| 73 | | mzpindd.5 |
. . . . . . . . . . . . . 14
              
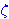   |
| 74 | 73 | elrab 3363 |
. . . . . . . . . . . . 13
                         
                   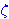   |
| 75 | | mzpindd.6 |
. . . . . . . . . . . . . 14
              
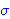   |
| 76 | 75 | elrab 3363 |
. . . . . . . . . . . . 13
                         
                   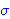   |
| 77 | 74, 76 | anbi12i 733 |
. . . . . . . . . . . 12
                                                                        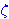 
                    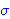    |
| 78 | 67, 72, 77 | 3imtr4g 285 |
. . . . . . . . . . 11
                         
                                                                    |
| 79 | 78 | ralrimivv 2970 |
. . . . . . . . . 10
                                                                                          |
| 80 | 79 | adantr 481 |
. . . . . . . . 9
    
   
                     
                                                                 |
| 81 | 6, 35, 80 | jca32 558 |
. . . . . . . 8
    
   
                                                                                                                       
                                                                   |
| 82 | | elmzpcl 37289 |
. . . . . . . . 9
        
               mzPolyCld mzPolyCld   
                                                                                                                      
                                                                    |
| 83 | 82 | adantl 482 |
. . . . . . . 8
    
   
                   mzPolyCld mzPolyCld   
                                                                                                                      
                                                                    |
| 84 | 81, 83 | mpbird 247 |
. . . . . . 7
    
   
                  mzPolyCld mzPolyCld    |
| 85 | | intss1 4492 |
. . . . . . 7
                   mzPolyCld mzPolyCld      mzPolyCld mzPolyCld                    |
| 86 | 84, 85 | syl 17 |
. . . . . 6
    
   
   mzPolyCld mzPolyCld                    |
| 87 | 4, 86 | eqsstrd 3639 |
. . . . 5
    
   
  mzPoly mzPoly                    |
| 88 | 87 | sselda 3603 |
. . . 4
              mzPoly mzPoly    
                  |
| 89 | 88 | an32s 846 |
. . 3
         mzPoly mzPoly    
   
                   |
| 90 | 2, 89 | mpdan 702 |
. 2
    
   mzPoly mzPoly                       |
| 91 | | mzpindd.7 |
. . . 4
         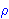   |
| 92 | 91 | elrab 3363 |
. . 3
                   
             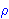   |
| 93 | 92 | simprbi 480 |
. 2
                    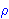  |
| 94 | 90, 93 | syl 17 |
1
    
   mzPoly mzPoly     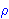  |