| Step | Hyp | Ref
| Expression |
|---|
| 1 | | catcxpccl.o |
. . . . 5
      c c   |
| 2 | | eqid 2622 |
. . . . 5
            |
| 3 | | eqid 2622 |
. . . . 5
            |
| 4 | | eqid 2622 |
. . . . 5
            |
| 5 | | eqid 2622 |
. . . . 5
            |
| 6 | | eqid 2622 |
. . . . 5
  comp comp     comp comp   |
| 7 | | eqid 2622 |
. . . . 5
  comp comp     comp comp   |
| 8 | | catcxpccl.x |
. . . . 5
        |
| 9 | | catcxpccl.y |
. . . . 5
        |
| 10 | | eqidd 2623 |
. . . . 5
                                |
| 11 | 1, 2, 3 | xpcbas 16818 |
. . . . . . 7
                    |
| 12 | | eqid 2622 |
. . . . . . 7
            |
| 13 | 1, 11, 4, 5, 12 | xpchomfval 16819 |
. . . . . 6
                                                                              |
| 14 | 13 | a1i 11 |
. . . . 5
                                                                                  |
| 15 | | eqidd 2623 |
. . . . 5
                                                                                                                comp comp                                             comp comp                                                                                                                               comp comp                                             comp comp                   |
| 16 | 1, 2, 3, 4, 5, 6, 7, 8, 9, 10,
14, 15 | xpcval 16817 |
. . . 4
           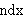                      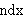  
         comp comp 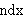                                                                                                               comp comp                                             comp comp                     |
| 17 | | catcxpccl.u |
. . . . 5
      WUni WUni |
| 18 | | df-base 15863 |
. . . . . . 7
   Slot Slot  |
| 19 | | catcxpccl.1 |
. . . . . . . 8
        |
| 20 | 17, 19 | wunndx 15878 |
. . . . . . 7
    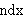    |
| 21 | 18, 17, 20 | wunstr 15881 |
. . . . . 6
       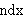  
  |
| 22 | | inss1 3833 |
. . . . . . . . 9
        |
| 23 | | catcxpccl.c |
. . . . . . . . . . 11
    CatCat CatCat   |
| 24 | | catcxpccl.b |
. . . . . . . . . . 11
        |
| 25 | 23, 24, 17 | catcbas 16747 |
. . . . . . . . . 10
            |
| 26 | 8, 25 | eleqtrd 2703 |
. . . . . . . . 9
            |
| 27 | 22, 26 | sseldi 3601 |
. . . . . . . 8
        |
| 28 | 18, 17, 27 | wunstr 15881 |
. . . . . . 7
            |
| 29 | 9, 25 | eleqtrd 2703 |
. . . . . . . . 9
            |
| 30 | 22, 29 | sseldi 3601 |
. . . . . . . 8
        |
| 31 | 18, 17, 30 | wunstr 15881 |
. . . . . . 7
            |
| 32 | 17, 28, 31 | wunxp 9546 |
. . . . . 6
                    |
| 33 | 17, 21, 32 | wunop 9544 |
. . . . 5
        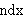  
                 |
| 34 | | df-hom 15966 |
. . . . . . 7
   Slot ; Slot ; 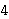 |
| 35 | 34, 17, 20 | wunstr 15881 |
. . . . . 6
       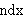  
  |
| 36 | 17, 32, 32 | wunxp 9546 |
. . . . . . . 8
                                    |
| 37 | 34, 17, 27 | wunstr 15881 |
. . . . . . . . . . . 12
            |
| 38 | 17, 37 | wunrn 9551 |
. . . . . . . . . . 11
             |
| 39 | 17, 38 | wununi 9528 |
. . . . . . . . . 10
              |
| 40 | 34, 17, 30 | wunstr 15881 |
. . . . . . . . . . . 12
            |
| 41 | 17, 40 | wunrn 9551 |
. . . . . . . . . . 11
             |
| 42 | 17, 41 | wununi 9528 |
. . . . . . . . . 10
              |
| 43 | 17, 39, 42 | wunxp 9546 |
. . . . . . . . 9
              
         |
| 44 | 17, 43 | wunpw 9529 |
. . . . . . . 8
                         |
| 45 | | ovssunirn 6681 |
. . . . . . . . . . . . 13
                          |
| 46 | | ovssunirn 6681 |
. . . . . . . . . . . . 13
                          |
| 47 | | xpss12 5225 |
. . . . . . . . . . . . 13
                                                       
                                                        |
| 48 | 45, 46, 47 | mp2an 708 |
. . . . . . . . . . . 12
                                                        |
| 49 | | ovex 6678 |
. . . . . . . . . . . . . 14
                    |
| 50 | | ovex 6678 |
. . . . . . . . . . . . . 14
                    |
| 51 | 49, 50 | xpex 6962 |
. . . . . . . . . . . . 13
                                        |
| 52 | 51 | elpw 4164 |
. . . . . . . . . . . 12
                                                          
                                                        |
| 53 | 48, 52 | mpbir 221 |
. . . . . . . . . . 11
                                                         |
| 54 | 53 | rgen2w 2925 |
. . . . . . . . . 10
                   
                                                                     |
| 55 | | eqid 2622 |
. . . . . . . . . . 11
                                                                                                                                                |
| 56 | 55 | fmpt2 7237 |
. . . . . . . . . 10
    
                                                                                     
                                                                                                                 
       |
| 57 | 54, 56 | mpbi 220 |
. . . . . . . . 9
                                                                                                                  
      |
| 58 | 57 | a1i 11 |
. . . . . . . 8
                                                                                                                     
       |
| 59 | 17, 36, 44, 58 | wunf 9549 |
. . . . . . 7
                                                                              |
| 60 | 13, 59 | syl5eqel 2705 |
. . . . . 6
            |
| 61 | 17, 35, 60 | wunop 9544 |
. . . . 5
        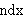  
         |
| 62 | | df-cco 15967 |
. . . . . . 7
 comp comp  Slot ; Slot ; 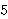 |
| 63 | 62, 17, 20 | wunstr 15881 |
. . . . . 6
     comp comp 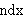     |
| 64 | 17, 36, 32 | wunxp 9546 |
. . . . . . 7
                                                    |
| 65 | 62, 17, 27 | wunstr 15881 |
. . . . . . . . . . . . . 14
     comp comp      |
| 66 | 17, 65 | wunrn 9551 |
. . . . . . . . . . . . 13
      comp comp      |
| 67 | 17, 66 | wununi 9528 |
. . . . . . . . . . . 12
       comp comp      |
| 68 | 17, 67 | wunrn 9551 |
. . . . . . . . . . 11
        comp comp      |
| 69 | 17, 68 | wununi 9528 |
. . . . . . . . . 10
         comp comp      |
| 70 | 17, 69 | wunpw 9529 |
. . . . . . . . 9
          comp comp      |
| 71 | 62, 17, 30 | wunstr 15881 |
. . . . . . . . . . . . . 14
     comp comp      |
| 72 | 17, 71 | wunrn 9551 |
. . . . . . . . . . . . 13
      comp comp      |
| 73 | 17, 72 | wununi 9528 |
. . . . . . . . . . . 12
       comp comp      |
| 74 | 17, 73 | wunrn 9551 |
. . . . . . . . . . 11
        comp comp      |
| 75 | 17, 74 | wununi 9528 |
. . . . . . . . . 10
         comp comp      |
| 76 | 17, 75 | wunpw 9529 |
. . . . . . . . 9
          comp comp      |
| 77 | 17, 70, 76 | wunxp 9546 |
. . . . . . . 8
           comp comp          comp comp       |
| 78 | 17, 60 | wunrn 9551 |
. . . . . . . . . 10
             |
| 79 | 17, 78 | wununi 9528 |
. . . . . . . . 9
              |
| 80 | 17, 79, 79 | wunxp 9546 |
. . . . . . . 8
              
         |
| 81 | 17, 77, 80 | wunpm 9547 |
. . . . . . 7
            comp comp          comp comp    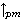                      |
| 82 | | fvex 6201 |
. . . . . . . . . . . . . . . . 17
  comp comp     |
| 83 | 82 | rnex 7100 |
. . . . . . . . . . . . . . . 16
   comp comp     |
| 84 | 83 | uniex 6953 |
. . . . . . . . . . . . . . 15
    comp comp     |
| 85 | 84 | rnex 7100 |
. . . . . . . . . . . . . 14
     comp comp     |
| 86 | 85 | uniex 6953 |
. . . . . . . . . . . . 13
      comp comp     |
| 87 | 86 | pwex 4848 |
. . . . . . . . . . . 12
       comp comp     |
| 88 | | fvex 6201 |
. . . . . . . . . . . . . . . . 17
  comp comp     |
| 89 | 88 | rnex 7100 |
. . . . . . . . . . . . . . . 16
   comp comp     |
| 90 | 89 | uniex 6953 |
. . . . . . . . . . . . . . 15
    comp comp     |
| 91 | 90 | rnex 7100 |
. . . . . . . . . . . . . 14
     comp comp     |
| 92 | 91 | uniex 6953 |
. . . . . . . . . . . . 13
      comp comp     |
| 93 | 92 | pwex 4848 |
. . . . . . . . . . . 12
       comp comp     |
| 94 | 87, 93 | xpex 6962 |
. . . . . . . . . . 11
    
   comp comp          comp comp      |
| 95 | | fvex 6201 |
. . . . . . . . . . . . . 14
        |
| 96 | 95 | rnex 7100 |
. . . . . . . . . . . . 13
         |
| 97 | 96 | uniex 6953 |
. . . . . . . . . . . 12
          |
| 98 | 97, 97 | xpex 6962 |
. . . . . . . . . . 11
                    |
| 99 | | ovssunirn 6681 |
. . . . . . . . . . . . . . . 16
                              comp comp                                         comp comp         |
| 100 | | ovssunirn 6681 |
. . . . . . . . . . . . . . . . 17
                        comp comp             comp comp   |
| 101 | | rnss 5354 |
. . . . . . . . . . . . . . . . 17
                         comp comp             comp comp                            comp comp              comp comp    |
| 102 | | uniss 4458 |
. . . . . . . . . . . . . . . . 17
                          comp comp              comp comp                             comp comp               comp comp    |
| 103 | 100, 101,
102 | mp2b 10 |
. . . . . . . . . . . . . . . 16
                          comp comp               comp comp   |
| 104 | 99, 103 | sstri 3612 |
. . . . . . . . . . . . . . 15
                              comp comp                     comp comp   |
| 105 | | ovex 6678 |
. . . . . . . . . . . . . . . 16
                              comp comp                 |
| 106 | 105 | elpw 4164 |
. . . . . . . . . . . . . . 15
                               comp comp                      comp comp   
                             comp comp                     comp comp    |
| 107 | 104, 106 | mpbir 221 |
. . . . . . . . . . . . . 14
                              comp comp                      comp comp   |
| 108 | | ovssunirn 6681 |
. . . . . . . . . . . . . . . 16
                              comp comp                                         comp comp         |
| 109 | | ovssunirn 6681 |
. . . . . . . . . . . . . . . . 17
                        comp comp             comp comp   |
| 110 | | rnss 5354 |
. . . . . . . . . . . . . . . . 17
                         comp comp             comp comp                            comp comp              comp comp    |
| 111 | | uniss 4458 |
. . . . . . . . . . . . . . . . 17
                          comp comp              comp comp                             comp comp               comp comp    |
| 112 | 109, 110,
111 | mp2b 10 |
. . . . . . . . . . . . . . . 16
                          comp comp               comp comp   |
| 113 | 108, 112 | sstri 3612 |
. . . . . . . . . . . . . . 15
                              comp comp                     comp comp   |
| 114 | | ovex 6678 |
. . . . . . . . . . . . . . . 16
                              comp comp                 |
| 115 | 114 | elpw 4164 |
. . . . . . . . . . . . . . 15
                               comp comp                      comp comp   
                             comp comp                     comp comp    |
| 116 | 113, 115 | mpbir 221 |
. . . . . . . . . . . . . 14
                              comp comp                      comp comp   |
| 117 | | opelxpi 5148 |
. . . . . . . . . . . . . 14
                                comp comp                      comp comp                                 comp comp                      comp comp                                   comp comp                                             comp comp                        comp comp          comp comp     |
| 118 | 107, 116,
117 | mp2an 708 |
. . . . . . . . . . . . 13
                               comp comp                                             comp comp                        comp comp          comp comp    |
| 119 | 118 | rgen2w 2925 |
. . . . . . . . . . . 12
                   
                                       comp comp                                             comp comp                        comp comp          comp comp    |
| 120 | | eqid 2622 |
. . . . . . . . . . . . 13
                                                            comp comp                                             comp comp                                                                             comp comp                                             comp comp                 |
| 121 | 120 | fmpt2 7237 |
. . . . . . . . . . . 12
    
                                                       comp comp                                             comp comp                        comp comp          comp comp                                                                comp comp                                             comp comp                                                   comp comp          comp comp     |
| 122 | 119, 121 | mpbi 220 |
. . . . . . . . . . 11
                                                            comp comp                                             comp comp                                                   comp comp          comp comp    |
| 123 | | ovssunirn 6681 |
. . . . . . . . . . . 12
                      |
| 124 | | fvssunirn 6217 |
. . . . . . . . . . . 12
                  |
| 125 | | xpss12 5225 |
. . . . . . . . . . . 12
                        
                  
                         
                  |
| 126 | 123, 124,
125 | mp2an 708 |
. . . . . . . . . . 11
                          
                 |
| 127 | | elpm2r 7875 |
. . . . . . . . . . 11
           comp comp          comp comp      
                                                                                 comp comp                                             comp comp                                                   comp comp          comp comp                                                                                                              comp comp                                             comp comp                          comp comp          comp comp    
                   |
| 128 | 94, 98, 122, 126, 127 | mp4an 709 |
. . . . . . . . . 10
                                                            comp comp                                             comp comp                          comp comp          comp comp    
                  |
| 129 | 128 | rgen2w 2925 |
. . . . . . . . 9
                                                                                                            comp comp                                             comp comp                          comp comp          comp comp    
                  |
| 130 | | eqid 2622 |
. . . . . . . . . 10
                                                                                                             comp comp                                             comp comp                                                                                                                               comp comp                                             comp comp                  |
| 131 | 130 | fmpt2 7237 |
. . . . . . . . 9
    
                               
                                                                        comp comp                                             comp comp                          comp comp          comp comp    
                  
                                                                                                            comp comp                                             comp comp                                                                         comp comp          comp comp    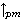
                   |
| 132 | 129, 131 | mpbi 220 |
. . . . . . . 8
                                                                                                             comp comp                                             comp comp                                                                         comp comp          comp comp    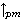
                  |
| 133 | 132 | a1i 11 |
. . . . . . 7
                                                                                                                comp comp                                             comp comp                                                                         comp comp          comp comp    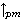
                   |
| 134 | 17, 64, 81, 133 | wunf 9549 |
. . . . . 6
                                                                                                                comp comp                                             comp comp                     |
| 135 | 17, 63, 134 | wunop 9544 |
. . . . 5
      comp comp 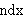                                                                                                               comp comp                                             comp comp                      |
| 136 | 17, 33, 61, 135 | wuntp 9533 |
. . . 4
         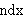  
                   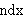            comp comp 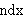                                                                                                               comp comp                                             comp comp                       |
| 137 | 16, 136 | eqeltrd 2701 |
. . 3
        |
| 138 | | inss2 3834 |
. . . . 5
        |
| 139 | 138, 26 | sseldi 3601 |
. . . 4
        |
| 140 | 138, 29 | sseldi 3601 |
. . . 4
        |
| 141 | 1, 139, 140 | xpccat 16830 |
. . 3
        |
| 142 | 137, 141 | elind 3798 |
. 2
            |
| 143 | 142, 25 | eleqtrrd 2704 |
1
        |