| Step | Hyp | Ref
| Expression |
|---|
| 1 | | prdsdsf.b |
. . . 4
        |
| 2 | | fvex 6201 |
. . . 4
        |
| 3 | 1, 2 | eqeltri 2697 |
. . 3
    |
| 4 | 3 | a1i 11 |
. 2
        |
| 5 | | prdsdsf.y |
. . . 4
     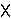 s s        |
| 6 | | prdsdsf.v |
. . . 4
        |
| 7 | | prdsdsf.e |
. . . 4
                |
| 8 | | prdsdsf.d |
. . . 4
        |
| 9 | | prdsdsf.s |
. . . 4
        |
| 10 | | prdsdsf.i |
. . . 4
        |
| 11 | | prdsdsf.r |
. . . 4
    
         |
| 12 | | prdsdsf.m |
. . . 4
    
              |
| 13 | 5, 1, 6, 7, 8, 9, 10, 11, 12 | prdsdsf 22172 |
. . 3
              ![[,] [,]](_icc.gif) 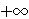   |
| 14 | | iccssxr 12256 |
. . 3
   ![[,] [,]](_icc.gif) 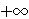    |
| 15 | | fss 6056 |
. . 3
             ![[,] [,]](_icc.gif) 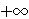     ![[,] [,]](_icc.gif) 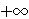                |
| 16 | 13, 14, 15 | sylancl 694 |
. 2
              |
| 17 | 13 | fovrnda 6805 |
. . 3
    
   
               ![[,] [,]](_icc.gif) 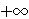   |
| 18 | | elxrge0 12281 |
. . . 4
          ![[,] [,]](_icc.gif) 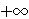 
         
         |
| 19 | 18 | simprbi 480 |
. . 3
          ![[,] [,]](_icc.gif) 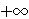 
         |
| 20 | 17, 19 | syl 17 |
. 2
    
   
        
      |
| 21 | 9 | adantr 481 |
. . . . 5
    
   
        
  |
| 22 | 10 | adantr 481 |
. . . . 5
    
   
        
  |
| 23 | 11 | ralrimiva 2966 |
. . . . . 6
         
  |
| 24 | 23 | adantr 481 |
. . . . 5
    
   
        
   
  |
| 25 | | simprl 794 |
. . . . 5
    
   
           |
| 26 | | simprr 796 |
. . . . 5
    
   
           |
| 27 | 5, 1, 21, 22, 24, 25, 26, 6, 7, 8 | prdsdsval3 16145 |
. . . 4
    
   
             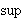   
   
                          |
| 28 | 27 | breq1d 4663 |
. . 3
    
   
                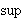                                     |
| 29 | 12 | adantlr 751 |
. . . . . . . 8
            
   
              |
| 30 | 5, 1, 21, 22, 24, 6, 25 | prdsbascl 16143 |
. . . . . . . . 9
    
   
        
          |
| 31 | 30 | r19.21bi 2932 |
. . . . . . . 8
            
   
             |
| 32 | 5, 1, 21, 22, 24, 6, 26 | prdsbascl 16143 |
. . . . . . . . 9
    
   
        
          |
| 33 | 32 | r19.21bi 2932 |
. . . . . . . 8
            
   
             |
| 34 | | xmetcl 22136 |
. . . . . . . 8
                                             |
| 35 | 29, 31, 33, 34 | syl3anc 1326 |
. . . . . . 7
            
   
                     |
| 36 | | eqid 2622 |
. . . . . . 7
                                        |
| 37 | 35, 36 | fmptd 6385 |
. . . . . 6
    
   
                               |
| 38 | | frn 6053 |
. . . . . 6
                                                 |
| 39 | 37, 38 | syl 17 |
. . . . 5
    
   
                              |
| 40 | | 0xr 10086 |
. . . . . . 7
    |
| 41 | 40 | a1i 11 |
. . . . . 6
    
   
           |
| 42 | 41 | snssd 4340 |
. . . . 5
    
   
             |
| 43 | 39, 42 | unssd 3789 |
. . . 4
    
   
        
   
                    
  |
| 44 | | supxrleub 12156 |
. . . 4
                             
        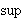                                                                      |
| 45 | 43, 40, 44 | sylancl 694 |
. . 3
    
   
        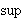                                                                  
   |
| 46 | | 0le0 11110 |
. . . . . . 7
    |
| 47 | | c0ex 10034 |
. . . . . . . 8
    |
| 48 | | breq1 4656 |
. . . . . . . 8
        
 
     |
| 49 | 47, 48 | ralsn 4222 |
. . . . . . 7
    
           |
| 50 | 46, 49 | mpbir 221 |
. . . . . 6
          |
| 51 | | ralunb 3794 |
. . . . . 6
    
   
                         
   
                     
             |
| 52 | 50, 51 | mpbiran2 954 |
. . . . 5
    
   
                         
                           |
| 53 | | ovex 6678 |
. . . . . . 7
                |
| 54 | 53 | rgenw 2924 |
. . . . . 6
    
               |
| 55 | | breq1 4656 |
. . . . . . 7
                    
 
                 |
| 56 | 36, 55 | ralrnmpt 6368 |
. . . . . 6
    
                   
   
                                      
   |
| 57 | 54, 56 | ax-mp 5 |
. . . . 5
    
                     
 
   
                |
| 58 | 52, 57 | bitri 264 |
. . . 4
    
   
                         
   
                |
| 59 | | xmetge0 22149 |
. . . . . . . . 9
                                             |
| 60 | 29, 31, 33, 59 | syl3anc 1326 |
. . . . . . . 8
            
   
                     |
| 61 | 60 | biantrud 528 |
. . . . . . 7
            
   
                   
 
                                   |
| 62 | | xrletri3 11985 |
. . . . . . . 8
                                                       
                    |
| 63 | 35, 40, 62 | sylancl 694 |
. . . . . . 7
            
   
                                    
                    |
| 64 | | xmeteq0 22143 |
. . . . . . . 8
                                                           |
| 65 | 29, 31, 33, 64 | syl3anc 1326 |
. . . . . . 7
            
   
                                   |
| 66 | 61, 63, 65 | 3bitr2d 296 |
. . . . . 6
            
   
                   
 
             |
| 67 | 66 | ralbidva 2985 |
. . . . 5
    
   
                                             |
| 68 | | eqid 2622 |
. . . . . . . . . 10
                |
| 69 | 68 | fnmpt 6020 |
. . . . . . . . 9
    
   
           |
| 70 | 23, 69 | syl 17 |
. . . . . . . 8
              |
| 71 | 70 | adantr 481 |
. . . . . . 7
    
   
                 |
| 72 | 5, 1, 21, 22, 71, 25 | prdsbasfn 16131 |
. . . . . 6
    
   
           |
| 73 | 5, 1, 21, 22, 71, 26 | prdsbasfn 16131 |
. . . . . 6
    
   
           |
| 74 | | eqfnfv 6311 |
. . . . . 6
        
         
               |
| 75 | 72, 73, 74 | syl2anc 693 |
. . . . 5
    
   
             
               |
| 76 | 67, 75 | bitr4d 271 |
. . . 4
    
   
                                 |
| 77 | 58, 76 | syl5bb 272 |
. . 3
    
   
                                              |
| 78 | 28, 45, 77 | 3bitrd 294 |
. 2
    
   
                     |
| 79 | 27 | 3adantr3 1222 |
. . . 4
    
   
   
             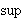   
   
                          |
| 80 | 79 | 3adant3 1081 |
. . 3
    
   
   
                        
      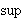                              
   |
| 81 | 12 | 3ad2antl1 1223 |
. . . . . . . . . 10
            
   
                                    |
| 82 | 30 | 3adantr3 1222 |
. . . . . . . . . . . 12
    
   
   
        
          |
| 83 | 82 | 3adant3 1081 |
. . . . . . . . . . 11
    
   
   
                        
   
        |
| 84 | 83 | r19.21bi 2932 |
. . . . . . . . . 10
            
   
                                   |
| 85 | 32 | 3adantr3 1222 |
. . . . . . . . . . . 12
    
   
   
        
          |
| 86 | 85 | 3adant3 1081 |
. . . . . . . . . . 11
    
   
   
                        
   
        |
| 87 | 86 | r19.21bi 2932 |
. . . . . . . . . 10
            
   
                                   |
| 88 | 81, 84, 87, 34 | syl3anc 1326 |
. . . . . . . . 9
            
   
                                           |
| 89 | 9 | 3ad2ant1 1082 |
. . . . . . . . . . . . . 14
    
   
   
                        
    |
| 90 | 10 | 3ad2ant1 1082 |
. . . . . . . . . . . . . 14
    
   
   
                        
    |
| 91 | 23 | 3ad2ant1 1082 |
. . . . . . . . . . . . . 14
    
   
   
                        
   
    |
| 92 | | simp23 1096 |
. . . . . . . . . . . . . 14
    
   
   
                        
    |
| 93 | 5, 1, 89, 90, 91, 6, 92 | prdsbascl 16143 |
. . . . . . . . . . . . 13
    
   
   
                        
   
        |
| 94 | 93 | r19.21bi 2932 |
. . . . . . . . . . . 12
            
   
                                   |
| 95 | | xmetcl 22136 |
. . . . . . . . . . . 12
                                             |
| 96 | 81, 94, 84, 95 | syl3anc 1326 |
. . . . . . . . . . 11
            
   
                                           |
| 97 | | simp3l 1089 |
. . . . . . . . . . . 12
    
   
   
                        
        |
| 98 | 97 | adantr 481 |
. . . . . . . . . . 11
            
   
                                   |
| 99 | | xmetge0 22149 |
. . . . . . . . . . . 12
                                             |
| 100 | 81, 94, 84, 99 | syl3anc 1326 |
. . . . . . . . . . 11
            
   
                                           |
| 101 | | eqid 2622 |
. . . . . . . . . . . . . . . . 17
                                        |
| 102 | 96, 101 | fmptd 6385 |
. . . . . . . . . . . . . . . 16
    
   
   
                        
   
                    |
| 103 | | frn 6053 |
. . . . . . . . . . . . . . . 16
                                                 |
| 104 | 102, 103 | syl 17 |
. . . . . . . . . . . . . . 15
    
   
   
                        
                       |
| 105 | 40 | a1i 11 |
. . . . . . . . . . . . . . . 16
    
   
   
                        
    |
| 106 | 105 | snssd 4340 |
. . . . . . . . . . . . . . 15
    
   
   
                        
      |
| 107 | 104, 106 | unssd 3789 |
. . . . . . . . . . . . . 14
    
   
   
                        
   
                         |
| 108 | 107 | adantr 481 |
. . . . . . . . . . . . 13
            
   
                                                        |
| 109 | | ssun1 3776 |
. . . . . . . . . . . . . 14
                     
   
                      |
| 110 | | ovex 6678 |
. . . . . . . . . . . . . . . . 17
                |
| 111 | 110 | elabrex 6501 |
. . . . . . . . . . . . . . . 16
                                            |
| 112 | 111 | adantl 482 |
. . . . . . . . . . . . . . 15
            
   
                                                                 |
| 113 | 101 | rnmpt 5371 |
. . . . . . . . . . . . . . 15
                                             |
| 114 | 112, 113 | syl6eleqr 2712 |
. . . . . . . . . . . . . 14
            
   
                                                              |
| 115 | 109, 114 | sseldi 3601 |
. . . . . . . . . . . . 13
            
   
                                                                    |
| 116 | | supxrub 12154 |
. . . . . . . . . . . . 13
                             
                                                         
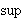   
   
                          |
| 117 | 108, 115,
116 | syl2anc 693 |
. . . . . . . . . . . 12
            
   
                                         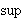                              
   |
| 118 | | simp21 1094 |
. . . . . . . . . . . . . 14
    
   
   
                        
    |
| 119 | 5, 1, 89, 90, 91, 92, 118, 6, 7, 8 | prdsdsval3 16145 |
. . . . . . . . . . . . 13
    
   
   
                        
      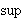                              
   |
| 120 | 119 | adantr 481 |
. . . . . . . . . . . 12
            
   
                                 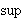                                  |
| 121 | 117, 120 | breqtrrd 4681 |
. . . . . . . . . . 11
            
   
                                               |
| 122 | | xrrege0 12005 |
. . . . . . . . . . 11
                                                                                    |
| 123 | 96, 98, 100, 121, 122 | syl22anc 1327 |
. . . . . . . . . 10
            
   
                                           |
| 124 | | xmetcl 22136 |
. . . . . . . . . . . 12
                                             |
| 125 | 81, 94, 87, 124 | syl3anc 1326 |
. . . . . . . . . . 11
            
   
                                           |
| 126 | | simp3r 1090 |
. . . . . . . . . . . 12
    
   
   
                        
        |
| 127 | 126 | adantr 481 |
. . . . . . . . . . 11
            
   
                                   |
| 128 | | xmetge0 22149 |
. . . . . . . . . . . 12
                                             |
| 129 | 81, 94, 87, 128 | syl3anc 1326 |
. . . . . . . . . . 11
            
   
                                           |
| 130 | | eqid 2622 |
. . . . . . . . . . . . . . . . 17
                                        |
| 131 | 125, 130 | fmptd 6385 |
. . . . . . . . . . . . . . . 16
    
   
   
                        
   
                    |
| 132 | | frn 6053 |
. . . . . . . . . . . . . . . 16
                                                 |
| 133 | 131, 132 | syl 17 |
. . . . . . . . . . . . . . 15
    
   
   
                        
                       |
| 134 | 133, 106 | unssd 3789 |
. . . . . . . . . . . . . 14
    
   
   
                        
   
                         |
| 135 | 134 | adantr 481 |
. . . . . . . . . . . . 13
            
   
                                                        |
| 136 | | ssun1 3776 |
. . . . . . . . . . . . . 14
                     
   
                      |
| 137 | | ovex 6678 |
. . . . . . . . . . . . . . . . 17
                |
| 138 | 137 | elabrex 6501 |
. . . . . . . . . . . . . . . 16
                                            |
| 139 | 138 | adantl 482 |
. . . . . . . . . . . . . . 15
            
   
                                                                 |
| 140 | 130 | rnmpt 5371 |
. . . . . . . . . . . . . . 15
                                             |
| 141 | 139, 140 | syl6eleqr 2712 |
. . . . . . . . . . . . . 14
            
   
                                                              |
| 142 | 136, 141 | sseldi 3601 |
. . . . . . . . . . . . 13
            
   
                                                                    |
| 143 | | supxrub 12154 |
. . . . . . . . . . . . 13
                             
                                                         
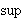   
   
                          |
| 144 | 135, 142,
143 | syl2anc 693 |
. . . . . . . . . . . 12
            
   
                                         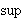                              
   |
| 145 | | simp22 1095 |
. . . . . . . . . . . . . 14
    
   
   
                        
    |
| 146 | 5, 1, 89, 90, 91, 92, 145, 6, 7, 8 | prdsdsval3 16145 |
. . . . . . . . . . . . 13
    
   
   
                        
      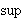                              
   |
| 147 | 146 | adantr 481 |
. . . . . . . . . . . 12
            
   
                                 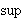                                  |
| 148 | 144, 147 | breqtrrd 4681 |
. . . . . . . . . . 11
            
   
                                               |
| 149 | | xrrege0 12005 |
. . . . . . . . . . 11
                                                                                    |
| 150 | 125, 127,
129, 148, 149 | syl22anc 1327 |
. . . . . . . . . 10
            
   
                                           |
| 151 | 123, 150 | readdcld 10069 |
. . . . . . . . 9
            
   
                                                           |
| 152 | 81, 84, 87, 59 | syl3anc 1326 |
. . . . . . . . 9
            
   
                                           |
| 153 | | xmettri2 22145 |
. . . . . . . . . . 11
                                                    
                               |
| 154 | 81, 94, 84, 87, 153 | syl13anc 1328 |
. . . . . . . . . 10
            
   
                                                                        |
| 155 | | rexadd 12063 |
. . . . . . . . . . 11
                                                                                                 |
| 156 | 123, 150,
155 | syl2anc 693 |
. . . . . . . . . 10
            
   
                                                                                        |
| 157 | 154, 156 | breqtrd 4679 |
. . . . . . . . 9
            
   
                                                                       |
| 158 | | xrrege0 12005 |
. . . . . . . . 9
                                                                                                                                    |
| 159 | 88, 151, 152, 157, 158 | syl22anc 1327 |
. . . . . . . 8
            
   
                                           |
| 160 | | readdcl 10019 |
. . . . . . . . . 10
                                    |
| 161 | 160 | 3ad2ant3 1084 |
. . . . . . . . 9
    
   
   
                        
                |
| 162 | 161 | adantr 481 |
. . . . . . . 8
            
   
                                           |
| 163 | 123, 150,
98, 127, 121, 148 | le2addd 10646 |
. . . . . . . 8
            
   
                                                                       |
| 164 | 159, 151,
162, 157, 163 | letrd 10194 |
. . . . . . 7
            
   
                                                       |
| 165 | 164 | ralrimiva 2966 |
. . . . . 6
    
   
   
                        
   
                            |
| 166 | 88 | ralrimiva 2966 |
. . . . . . 7
    
   
   
                        
   
                |
| 167 | | breq1 4656 |
. . . . . . . 8
                    
             
                             |
| 168 | 36, 167 | ralrnmpt 6368 |
. . . . . . 7
    
               
    
                     
             
   
                             |
| 169 | 166, 168 | syl 17 |
. . . . . 6
    
   
   
                        
   
                     
             
   
                             |
| 170 | 165, 169 | mpbird 247 |
. . . . 5
    
   
   
                        
                                       |
| 171 | 13 | 3ad2ant1 1082 |
. . . . . . . . 9
    
   
   
                        
          ![[,] [,]](_icc.gif) 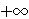   |
| 172 | 171, 92, 118 | fovrnd 6806 |
. . . . . . . 8
    
   
   
                        
        ![[,] [,]](_icc.gif) 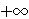   |
| 173 | | elxrge0 12281 |
. . . . . . . . 9
          ![[,] [,]](_icc.gif) 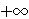 
         
         |
| 174 | 173 | simprbi 480 |
. . . . . . . 8
          ![[,] [,]](_icc.gif) 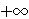 
         |
| 175 | 172, 174 | syl 17 |
. . . . . . 7
    
   
   
                        
        |
| 176 | 171, 92, 145 | fovrnd 6806 |
. . . . . . . 8
    
   
   
                        
        ![[,] [,]](_icc.gif) 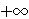   |
| 177 | | elxrge0 12281 |
. . . . . . . . 9
          ![[,] [,]](_icc.gif) 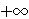 
         
         |
| 178 | 177 | simprbi 480 |
. . . . . . . 8
          ![[,] [,]](_icc.gif)  
         |
| 179 | 176, 178 | syl 17 |
. . . . . . 7
    
   
   
                        
        |
| 180 | 97, 126, 175, 179 | addge0d 10603 |
. . . . . 6
    
   
   
                        
                |
| 181 | | breq1 4656 |
. . . . . . 7
        
             
                 |
| 182 | 47, 181 | ralsn 4222 |
. . . . . 6
    
                    
              |
| 183 | 180, 182 | sylibr 224 |
. . . . 5
    
   
   
                        
                      |
| 184 | | ralunb 3794 |
. . . . 5
    
   
                                     
   
                     
                                     |
| 185 | 170, 183,
184 | sylanbrc 698 |
. . . 4
    
   
   
                        
                                             |
| 186 | 43 | 3adantr3 1222 |
. . . . . 6
    
   
   
        
   
                    
  |
| 187 | 186 | 3adant3 1081 |
. . . . 5
    
   
   
                        
   
                         |
| 188 | 161 | rexrd 10089 |
. . . . 5
    
   
   
                        
                |
| 189 | | supxrleub 12156 |
. . . . 5
                             
                                            |
| 190 | 187, 188,
189 | syl2anc 693 |
. . . 4
    
   
   
                        
                                            |
| 191 | 185, 190 | mpbird 247 |
. . 3
    
   
   
                        
   
                                        |
| 192 | 80, 191 | eqbrtrd 4675 |
. 2
    
   
   
                        
                    |
| 193 | 4, 16, 20, 78, 192 | isxmet2d 22132 |
1
             |