| Step | Hyp | Ref
| Expression |
|---|
| 1 | | rexnal 2995 |
. . . . 5
              
    
        |
| 2 | | df-ne 2795 |
. . . . . . 7
         
         |
| 3 | | oveq2 6658 |
. . . . . . . . . . . 12
       ℂfld ℂfld  g g     ℂfld ℂfld  g g    |
| 4 | | tdeglem.h |
. . . . . . . . . . . 12
         ℂfld ℂfld  g g    |
| 5 | | ovex 6678 |
. . . . . . . . . . . 12
  ℂfld ℂfld  g g     |
| 6 | 3, 4, 5 | fvmpt 6282 |
. . . . . . . . . . 11
             ℂfld ℂfld  g g    |
| 7 | 6 | ad2antlr 763 |
. . . . . . . . . 10
        
   
   
                  ℂfld ℂfld  g g    |
| 8 | | tdeglem.a |
. . . . . . . . . . . . . 14
                     |
| 9 | 8 | psrbagf 19365 |
. . . . . . . . . . . . 13
        
         |
| 10 | 9 | feqmptd 6249 |
. . . . . . . . . . . 12
        
                 |
| 11 | 10 | adantr 481 |
. . . . . . . . . . 11
        
   
   
            
   
        |
| 12 | 11 | oveq2d 6666 |
. . . . . . . . . 10
        
   
   
            ℂfld ℂfld  g g     ℂfld ℂfld  g g              |
| 13 | | cnfldbas 19750 |
. . . . . . . . . . 11
      ℂfld ℂfld |
| 14 | | cnfld0 19770 |
. . . . . . . . . . 11
      ℂfld ℂfld |
| 15 | | cnfldadd 19751 |
. . . . . . . . . . 11
      ℂfld ℂfld |
| 16 | | cnring 19768 |
. . . . . . . . . . . 12
 ℂfld
ℂfld   |
| 17 | | ringcmn 18581 |
. . . . . . . . . . . 12
  ℂfld ℂfld    ℂfld ℂfld  CMnd CMnd |
| 18 | 16, 17 | mp1i 13 |
. . . . . . . . . . 11
        
   
   
           ℂfld ℂfld  CMnd CMnd |
| 19 | | simpll 790 |
. . . . . . . . . . 11
        
   
   
            
  |
| 20 | 9 | adantr 481 |
. . . . . . . . . . . . 13
        
   
   
                 |
| 21 | 20 | ffvelrnda 6359 |
. . . . . . . . . . . 12
                                 
        |
| 22 | 21 | nn0cnd 11353 |
. . . . . . . . . . 11
                                 
        |
| 23 | 8 | psrbagfsupp 19509 |
. . . . . . . . . . . . . 14
        
    finSupp finSupp   |
| 24 | 23 | ancoms 469 |
. . . . . . . . . . . . 13
        
    finSupp finSupp   |
| 25 | 24 | adantr 481 |
. . . . . . . . . . . 12
        
   
   
            finSupp finSupp   |
| 26 | 11, 25 | eqbrtrrd 4677 |
. . . . . . . . . . 11
        
   
   
                      finSupp finSupp
  |
| 27 | | incom 3805 |
. . . . . . . . . . . . 13
                            |
| 28 | | disjdif 4040 |
. . . . . . . . . . . . 13
        
       |
| 29 | 27, 28 | eqtri 2644 |
. . . . . . . . . . . 12
                |
| 30 | 29 | a1i 11 |
. . . . . . . . . . 11
        
   
   
                           |
| 31 | | difsnid 4341 |
. . . . . . . . . . . . 13
                      |
| 32 | 31 | eqcomd 2628 |
. . . . . . . . . . . 12
                      |
| 33 | 32 | ad2antrl 764 |
. . . . . . . . . . 11
        
   
   
            
   
          |
| 34 | 13, 14, 15, 18, 19, 22, 26, 30, 33 | gsumsplit2 18329 |
. . . . . . . . . 10
        
   
   
            ℂfld ℂfld  g g                ℂfld ℂfld  g g                     ℂfld ℂfld  g g                 |
| 35 | 7, 12, 34 | 3eqtrd 2660 |
. . . . . . . . 9
        
   
   
                   ℂfld ℂfld  g g                     ℂfld ℂfld  g g                 |
| 36 | | difexg 4808 |
. . . . . . . . . . . . 13
        
       |
| 37 | 36 | ad2antrr 762 |
. . . . . . . . . . . 12
        
   
   
                     |
| 38 | | nn0subm 19801 |
. . . . . . . . . . . . 13
    SubMnd SubMnd ℂfld ℂfld |
| 39 | 38 | a1i 11 |
. . . . . . . . . . . 12
        
   
   
              SubMnd SubMnd ℂfld ℂfld  |
| 40 | | eldifi 3732 |
. . . . . . . . . . . . . 14
                |
| 41 | | ffvelrn 6357 |
. . . . . . . . . . . . . 14
          
           |
| 42 | 20, 40, 41 | syl2an 494 |
. . . . . . . . . . . . 13
                                                |
| 43 | | eqid 2622 |
. . . . . . . . . . . . 13
                                    |
| 44 | 42, 43 | fmptd 6385 |
. . . . . . . . . . . 12
        
   
   
                                       |
| 45 | | mptexg 6484 |
. . . . . . . . . . . . . . 15
                                |
| 46 | 36, 45 | syl 17 |
. . . . . . . . . . . . . 14
                          |
| 47 | 46 | ad2antrr 762 |
. . . . . . . . . . . . 13
        
   
   
                               |
| 48 | | funmpt 5926 |
. . . . . . . . . . . . . 14
                   |
| 49 | 48 | a1i 11 |
. . . . . . . . . . . . 13
        
   
   
                              |
| 50 | | funmpt 5926 |
. . . . . . . . . . . . . . 15
             |
| 51 | 50 | a1i 11 |
. . . . . . . . . . . . . 14
        
   
   
                        |
| 52 | | difss 3737 |
. . . . . . . . . . . . . . . . 17
        
 |
| 53 | | resmpt 5449 |
. . . . . . . . . . . . . . . . 17
                          
                         |
| 54 | 52, 53 | ax-mp 5 |
. . . . . . . . . . . . . . . 16
             
                          |
| 55 | | resss 5422 |
. . . . . . . . . . . . . . . 16
             
        
   
       |
| 56 | 54, 55 | eqsstr3i 3636 |
. . . . . . . . . . . . . . 15
                              |
| 57 | 56 | a1i 11 |
. . . . . . . . . . . . . 14
        
   
   
                                         |
| 58 | | mptexg 6484 |
. . . . . . . . . . . . . . 15
                    |
| 59 | 58 | ad2antrr 762 |
. . . . . . . . . . . . . 14
        
   
   
                         |
| 60 | | funsssuppss 7321 |
. . . . . . . . . . . . . 14
                                                                               supp supp                supp supp    |
| 61 | 51, 57, 59, 60 | syl3anc 1326 |
. . . . . . . . . . . . 13
        
   
   
                             supp supp                supp supp    |
| 62 | | fsuppsssupp 8291 |
. . . . . . . . . . . . 13
                                                        finSupp finSupp                     supp supp                supp supp                       finSupp finSupp   |
| 63 | 47, 49, 26, 61, 62 | syl22anc 1327 |
. . . . . . . . . . . 12
        
   
   
                            finSupp finSupp   |
| 64 | 14, 18, 37, 39, 44, 63 | gsumsubmcl 18319 |
. . . . . . . . . . 11
        
   
   
            ℂfld ℂfld  g g                      |
| 65 | | ringmnd 18556 |
. . . . . . . . . . . . . 14
  ℂfld ℂfld    ℂfld ℂfld  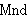  |
| 66 | 16, 65 | mp1i 13 |
. . . . . . . . . . . . 13
        
   
   
           ℂfld ℂfld  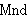  |
| 67 | | simprl 794 |
. . . . . . . . . . . . 13
        
   
   
            
  |
| 68 | 20, 67 | ffvelrnd 6360 |
. . . . . . . . . . . . . 14
        
   
   
                   |
| 69 | 68 | nn0cnd 11353 |
. . . . . . . . . . . . 13
        
   
   
                   |
| 70 | | fveq2 6191 |
. . . . . . . . . . . . . 14
                  |
| 71 | 13, 70 | gsumsn 18354 |
. . . . . . . . . . . . 13
   ℂfld ℂfld  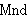 
              ℂfld ℂfld  g g                      |
| 72 | 66, 67, 69, 71 | syl3anc 1326 |
. . . . . . . . . . . 12
        
   
   
            ℂfld ℂfld  g g                      |
| 73 | | simprr 796 |
. . . . . . . . . . . . . 14
        
   
   
                   |
| 74 | 73, 2 | sylib 208 |
. . . . . . . . . . . . 13
        
   
   
                    |
| 75 | | elnn0 11294 |
. . . . . . . . . . . . . 14
        
                   |
| 76 | 68, 75 | sylib 208 |
. . . . . . . . . . . . 13
        
   
   
                             |
| 77 | | orel2 398 |
. . . . . . . . . . . . 13
                                       |
| 78 | 74, 76, 77 | sylc 65 |
. . . . . . . . . . . 12
        
   
   
                   |
| 79 | 72, 78 | eqeltrd 2701 |
. . . . . . . . . . 11
        
   
   
            ℂfld ℂfld  g g                  |
| 80 | | nn0nnaddcl 11324 |
. . . . . . . . . . 11
    ℂfld ℂfld  g g                       ℂfld ℂfld  g g                     ℂfld ℂfld  g g                     ℂfld ℂfld  g g                   |
| 81 | 64, 79, 80 | syl2anc 693 |
. . . . . . . . . 10
        
   
   
             ℂfld ℂfld  g g                     ℂfld ℂfld  g g                   |
| 82 | 81 | nnne0d 11065 |
. . . . . . . . 9
        
   
   
             ℂfld ℂfld  g g                     ℂfld ℂfld  g g                   |
| 83 | 35, 82 | eqnetrd 2861 |
. . . . . . . 8
        
   
   
                   |
| 84 | 83 | expr 643 |
. . . . . . 7
        
   
                       |
| 85 | 2, 84 | syl5bir 233 |
. . . . . 6
        
   
                        |
| 86 | 85 | rexlimdva 3031 |
. . . . 5
        
                
         |
| 87 | 1, 86 | syl5bir 233 |
. . . 4
        
        
                 |
| 88 | 87 | necon4bd 2814 |
. . 3
        
                         |
| 89 | | ffn 6045 |
. . . . . 6
            |
| 90 | 9, 89 | syl 17 |
. . . . 5
        
       |
| 91 | | 0nn0 11307 |
. . . . . 6
    |
| 92 | | fnconstg 6093 |
. . . . . 6
    
           |
| 93 | 91, 92 | mp1i 13 |
. . . . 5
        
             |
| 94 | | eqfnfv 6311 |
. . . . 5
        
        
            
                     |
| 95 | 90, 93, 94 | syl2anc 693 |
. . . 4
        
             
   
                   |
| 96 | | c0ex 10034 |
. . . . . . 7
    |
| 97 | 96 | fvconst2 6469 |
. . . . . 6
                    |
| 98 | 97 | eqeq2d 2632 |
. . . . 5
                                  |
| 99 | 98 | ralbiia 2979 |
. . . 4
    
                    
          |
| 100 | 95, 99 | syl6bb 276 |
. . 3
        
             
   
         |
| 101 | 88, 100 | sylibrd 249 |
. 2
        
                       |
| 102 | 8 | psrbag0 19494 |
. . . . . 6
                |
| 103 | 102 | adantr 481 |
. . . . 5
        
             |
| 104 | | oveq2 6658 |
. . . . . 6
             ℂfld ℂfld  g g     ℂfld ℂfld  g g          |
| 105 | | ovex 6678 |
. . . . . 6
  ℂfld ℂfld  g g           |
| 106 | 104, 4, 105 | fvmpt 6282 |
. . . . 5
                         ℂfld ℂfld  g g  
       |
| 107 | 103, 106 | syl 17 |
. . . 4
        
                ℂfld ℂfld  g g  
       |
| 108 | | fconstmpt 5163 |
. . . . . 6
                |
| 109 | 108 | oveq2i 6661 |
. . . . 5
  ℂfld ℂfld  g g           ℂfld ℂfld  g g  
      |
| 110 | 16, 65 | ax-mp 5 |
. . . . . . 7
 ℂfld
ℂfld  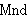 |
| 111 | 14 | gsumz 17374 |
. . . . . . 7
   ℂfld ℂfld  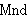 
      ℂfld ℂfld  g g            |
| 112 | 110, 111 | mpan 706 |
. . . . . 6
       ℂfld ℂfld  g g            |
| 113 | 112 | adantr 481 |
. . . . 5
        
    ℂfld ℂfld  g g  
         |
| 114 | 109, 113 | syl5eq 2668 |
. . . 4
        
    ℂfld ℂfld  g g  
         |
| 115 | 107, 114 | eqtrd 2656 |
. . 3
        
                 |
| 116 | | fveq2 6191 |
. . . 4
                              |
| 117 | 116 | eqeq1d 2624 |
. . 3
                         
          |
| 118 | 115, 117 | syl5ibrcom 237 |
. 2
        
                       |
| 119 | 101, 118 | impbid 202 |
1
        
                       |