| Step | Hyp | Ref
| Expression |
|---|
| 1 | | elssuni 4467 |
. . . 4
     ordTop ordTop        ordTop ordTop    |
| 2 | | letopuni 21011 |
. . . 4
     ordTop ordTop
  |
| 3 | 1, 2 | syl6sseqr 3652 |
. . 3
     ordTop ordTop        |
| 4 | | eqid 2622 |
. . . . . . . . 9
   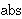              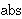           |
| 5 | 4 | rexmet 22594 |
. . . . . . . 8
   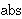                  |
| 6 | 5 | a1i 11 |
. . . . . . 7
       ordTop ordTop                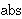
                  |
| 7 | | letop 21010 |
. . . . . . . . 9
  ordTop ordTop     |
| 8 | | reex 10027 |
. . . . . . . . 9
    |
| 9 | | elrestr 16089 |
. . . . . . . . 9
    ordTop ordTop
       ordTop ordTop          
  ordTop ordTop   ↾t
↾t    |
| 10 | 7, 8, 9 | mp3an12 1414 |
. . . . . . . 8
     ordTop ordTop            ordTop ordTop
  ↾t ↾t    |
| 11 | 10 | ad2antrr 762 |
. . . . . . 7
       ordTop ordTop                
     ordTop ordTop   ↾t ↾t    |
| 12 | | elin 3796 |
. . . . . . . . 9
         
   
      |
| 13 | 12 | biimpri 218 |
. . . . . . . 8
        
           |
| 14 | 13 | adantll 750 |
. . . . . . 7
       ordTop ordTop                      |
| 15 | | eqid 2622 |
. . . . . . . . . 10
   ordTop ordTop   ↾t
↾t      ordTop ordTop
  ↾t ↾t   |
| 16 | 15 | xrtgioo 22609 |
. . . . . . . . 9
          ordTop ordTop
  ↾t ↾t   |
| 17 | | eqid 2622 |
. . . . . . . . . 10
      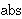   
              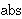   
        |
| 18 | 4, 17 | tgioo 22599 |
. . . . . . . . 9
     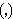    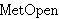    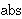  
         |
| 19 | 16, 18 | eqtr3i 2646 |
. . . . . . . 8
   ordTop ordTop   ↾t
↾t     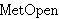    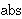  
         |
| 20 | 19 | mopni2 22298 |
. . . . . . 7
     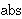   
                 
     ordTop ordTop   ↾t ↾t                        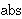      
       
      |
| 21 | 6, 11, 14, 20 | syl3anc 1326 |
. . . . . 6
       ordTop ordTop                         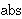      
       
      |
| 22 | | xrsxmet.1 |
. . . . . . . . . . . . 13
         |
| 23 | 22 | xrsxmet 22612 |
. . . . . . . . . . . 12
         |
| 24 | 23 | a1i 11 |
. . . . . . . . . . 11
        ordTop ordTop
    
   
                  |
| 25 | | simplr 792 |
. . . . . . . . . . . 12
        ordTop ordTop
    
   
             |
| 26 | | ressxr 10083 |
. . . . . . . . . . . . 13
    |
| 27 | | sseqin2 3817 |
. . . . . . . . . . . . 13
              |
| 28 | 26, 27 | mpbi 220 |
. . . . . . . . . . . 12
        |
| 29 | 25, 28 | syl6eleqr 2712 |
. . . . . . . . . . 11
        ordTop ordTop
    
   
             
   |
| 30 | | rpxr 11840 |
. . . . . . . . . . . 12
    
     |
| 31 | 30 | adantl 482 |
. . . . . . . . . . 11
        ordTop ordTop
    
   
             |
| 32 | 22 | xrsdsre 22613 |
. . . . . . . . . . . . 13
             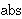           |
| 33 | 32 | eqcomi 2631 |
. . . . . . . . . . . 12
   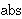              
      |
| 34 | 33 | blres 22236 |
. . . . . . . . . . 11
            
        
          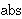   
                         |
| 35 | 24, 29, 31, 34 | syl3anc 1326 |
. . . . . . . . . 10
        ordTop ordTop
    
   
                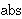      
                      |
| 36 | 22 | xrsblre 22614 |
. . . . . . . . . . . . 13
        
               |
| 37 | 30, 36 | sylan2 491 |
. . . . . . . . . . . 12
        
               |
| 38 | 37 | adantll 750 |
. . . . . . . . . . 11
        ordTop ordTop
    
   
                     |
| 39 | | df-ss 3588 |
. . . . . . . . . . 11
                           
          |
| 40 | 38, 39 | sylib 208 |
. . . . . . . . . 10
        ordTop ordTop
    
   
                                 |
| 41 | 35, 40 | eqtrd 2656 |
. . . . . . . . 9
        ordTop ordTop
    
   
                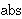      
                  |
| 42 | 41 | sseq1d 3632 |
. . . . . . . 8
        ordTop ordTop
    
   
                 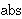
             
     
            
    |
| 43 | | inss1 3833 |
. . . . . . . . 9
        |
| 44 | | sstr 3611 |
. . . . . . . . 9
                     
                  |
| 45 | 43, 44 | mpan2 707 |
. . . . . . . 8
             
                |
| 46 | 42, 45 | syl6bi 243 |
. . . . . . 7
        ordTop ordTop
    
   
                 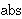
             
                   |
| 47 | 46 | reximdva 3017 |
. . . . . 6
       ordTop ordTop                
         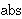
             
                       |
| 48 | 21, 47 | mpd 15 |
. . . . 5
       ordTop ordTop                              |
| 49 | | 1rp 11836 |
. . . . . 6
    |
| 50 | 23 | a1i 11 |
. . . . . . . . 9
       ordTop ordTop           
            |
| 51 | 3 | sselda 3603 |
. . . . . . . . . 10
      ordTop ordTop             |
| 52 | 51 | adantr 481 |
. . . . . . . . 9
       ordTop ordTop           
       |
| 53 | | rpxr 11840 |
. . . . . . . . . 10
    
     |
| 54 | 49, 53 | mp1i 13 |
. . . . . . . . 9
       ordTop ordTop           
       |
| 55 | | elbl 22193 |
. . . . . . . . 9
            
                    
               |
| 56 | 50, 52, 54, 55 | syl3anc 1326 |
. . . . . . . 8
       ordTop ordTop           
               
               |
| 57 | | simp2 1062 |
. . . . . . . . . 10
       ordTop ordTop           
                 
    |
| 58 | 23 | a1i 11 |
. . . . . . . . . . . . . . . . 17
        ordTop ordTop
    
                                     |
| 59 | 51 | 3ad2ant1 1082 |
. . . . . . . . . . . . . . . . . 18
       ordTop ordTop           
                     |
| 60 | 59 | adantr 481 |
. . . . . . . . . . . . . . . . 17
        ordTop ordTop
    
                                |
| 61 | | simpl3l 1116 |
. . . . . . . . . . . . . . . . 17
        ordTop ordTop
    
                                |
| 62 | | xmetcl 22136 |
. . . . . . . . . . . . . . . . 17
            
                |
| 63 | 58, 60, 61, 62 | syl3anc 1326 |
. . . . . . . . . . . . . . . 16
        ordTop ordTop
    
                                    |
| 64 | | 1red 10055 |
. . . . . . . . . . . . . . . 16
        ordTop ordTop
    
                                |
| 65 | | xmetge0 22149 |
. . . . . . . . . . . . . . . . 17
            
                |
| 66 | 58, 60, 61, 65 | syl3anc 1326 |
. . . . . . . . . . . . . . . 16
        ordTop ordTop
    
                                    |
| 67 | | simpl3r 1117 |
. . . . . . . . . . . . . . . . 17
        ordTop ordTop
    
                                    |
| 68 | 49, 53 | ax-mp 5 |
. . . . . . . . . . . . . . . . . 18
    |
| 69 | | xrltle 11982 |
. . . . . . . . . . . . . . . . . 18
                              
   |
| 70 | 63, 68, 69 | sylancl 694 |
. . . . . . . . . . . . . . . . 17
        ordTop ordTop
    
                                          
   |
| 71 | 67, 70 | mpd 15 |
. . . . . . . . . . . . . . . 16
        ordTop ordTop
    
                                    |
| 72 | | xrrege0 12005 |
. . . . . . . . . . . . . . . 16
                                            |
| 73 | 63, 64, 66, 71, 72 | syl22anc 1327 |
. . . . . . . . . . . . . . 15
        ordTop ordTop
    
                                    |
| 74 | | simpr 477 |
. . . . . . . . . . . . . . . 16
        ordTop ordTop
    
                                |
| 75 | 22 | xrsdsreclb 19793 |
. . . . . . . . . . . . . . . 16
                        
           |
| 76 | 60, 61, 74, 75 | syl3anc 1326 |
. . . . . . . . . . . . . . 15
        ordTop ordTop
    
                                    
           |
| 77 | 73, 76 | mpbid 222 |
. . . . . . . . . . . . . 14
        ordTop ordTop
    
                                  
   |
| 78 | 77 | simpld 475 |
. . . . . . . . . . . . 13
        ordTop ordTop
    
                                |
| 79 | 78 | ex 450 |
. . . . . . . . . . . 12
       ordTop ordTop           
                     
     |
| 80 | 79 | necon1bd 2812 |
. . . . . . . . . . 11
       ordTop ordTop           
                      
     |
| 81 | | simp1r 1086 |
. . . . . . . . . . . 12
       ordTop ordTop           
                     |
| 82 | | elequ1 1997 |
. . . . . . . . . . . 12
          
     |
| 83 | 81, 82 | syl5ibcom 235 |
. . . . . . . . . . 11
       ordTop ordTop           
                     
     |
| 84 | 80, 83 | syld 47 |
. . . . . . . . . 10
       ordTop ordTop           
                      
     |
| 85 | 57, 84 | mpd 15 |
. . . . . . . . 9
       ordTop ordTop           
                     |
| 86 | 85 | 3expia 1267 |
. . . . . . . 8
       ordTop ordTop           
        
              |
| 87 | 56, 86 | sylbid 230 |
. . . . . . 7
       ordTop ordTop           
                     |
| 88 | 87 | ssrdv 3609 |
. . . . . 6
       ordTop ordTop           
               |
| 89 | | oveq2 6658 |
. . . . . . . 8
                          |
| 90 | 89 | sseq1d 3632 |
. . . . . . 7
                  
             |
| 91 | 90 | rspcev 3309 |
. . . . . 6
                                    |
| 92 | 49, 88, 91 | sylancr 695 |
. . . . 5
       ordTop ordTop           
                   |
| 93 | 48, 92 | pm2.61dan 832 |
. . . 4
      ordTop ordTop                         |
| 94 | 93 | ralrimiva 2966 |
. . 3
     ordTop ordTop         
              |
| 95 | | xrsmopn.1 |
. . . . 5
    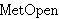    |
| 96 | 95 | elmopn2 22250 |
. . . 4
               
    
   
                  |
| 97 | 23, 96 | ax-mp 5 |
. . 3
     
    
   
                 |
| 98 | 3, 94, 97 | sylanbrc 698 |
. 2
     ordTop ordTop        |
| 99 | 98 | ssriv 3607 |
1
  ordTop ordTop   
 |