| Step | Hyp | Ref
| Expression |
|---|
| 1 | | isogrp 29702 |
. . . . 5
    oGrp oGrp         oMnd oMnd  |
| 2 | 1 | simprbi 480 |
. . . 4
    oGrp oGrp    oMnd oMnd |
| 3 | | omndtos 29705 |
. . . 4
    oMnd oMnd    Toset Toset |
| 4 | 2, 3 | syl 17 |
. . 3
    oGrp oGrp    Toset Toset |
| 5 | | grpmnd 17429 |
. . . . 5
        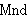  |
| 6 | 5 | adantr 481 |
. . . 4
         oMnd
oMnd    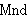  |
| 7 | 1, 6 | sylbi 207 |
. . 3
    oGrp oGrp    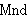  |
| 8 | | isarchi3.b |
. . . 4
        |
| 9 | | isarchi3.0 |
. . . 4
        |
| 10 | | isarchi3.x |
. . . 4
    .g .g   |
| 11 | | eqid 2622 |
. . . 4
            |
| 12 | | isarchi3.i |
. . . 4
        |
| 13 | 8, 9, 10, 11, 12 | isarchi2 29739 |
. . 3
     Toset Toset
   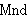      Archi
Archi       
    
                    |
| 14 | 4, 7, 13 | syl2anc 693 |
. 2
    oGrp oGrp     Archi Archi        
                        |
| 15 | | simpr 477 |
. . . . . . . . . . 11
        oGrp oGrp 
                        |
| 16 | 15 | adantr 481 |
. . . . . . . . . 10
         oGrp oGrp                                    
  |
| 17 | 16 | peano2nnd 11037 |
. . . . . . . . 9
         oGrp oGrp                                           |
| 18 | | simp-4l 806 |
. . . . . . . . . . . . 13
        oGrp oGrp 
                      oGrp oGrp |
| 19 | 18 | adantr 481 |
. . . . . . . . . . . 12
         oGrp oGrp                                     oGrp
oGrp |
| 20 | | ogrpgrp 29703 |
. . . . . . . . . . . . 13
    oGrp oGrp      |
| 21 | 8, 9 | grpidcl 17450 |
. . . . . . . . . . . . 13
          |
| 22 | 19, 20, 21 | 3syl 18 |
. . . . . . . . . . . 12
         oGrp oGrp                                       |
| 23 | | simp-4r 807 |
. . . . . . . . . . . . 13
        oGrp oGrp 
                        |
| 24 | 23 | adantr 481 |
. . . . . . . . . . . 12
         oGrp oGrp                                    
  |
| 25 | 20 | ad4antr 768 |
. . . . . . . . . . . . . 14
        oGrp oGrp 
                        |
| 26 | 15 | nnzd 11481 |
. . . . . . . . . . . . . 14
        oGrp oGrp 
                        |
| 27 | 8, 10 | mulgcl 17559 |
. . . . . . . . . . . . . 14
        
         
     |
| 28 | 25, 26, 23, 27 | syl3anc 1326 |
. . . . . . . . . . . . 13
        oGrp oGrp 
                      
     |
| 29 | 28 | adantr 481 |
. . . . . . . . . . . 12
         oGrp oGrp                                           |
| 30 | | simpllr 799 |
. . . . . . . . . . . 12
         oGrp oGrp                                       |
| 31 | | eqid 2622 |
. . . . . . . . . . . . 13
            |
| 32 | 8, 12, 31 | ogrpaddlt 29718 |
. . . . . . . . . . . 12
     oGrp oGrp         
   
                                       |
| 33 | 19, 22, 24, 29, 30, 32 | syl131anc 1339 |
. . . . . . . . . . 11
         oGrp oGrp                                                               |
| 34 | 19, 20 | syl 17 |
. . . . . . . . . . . 12
         oGrp oGrp                                    
  |
| 35 | 8, 31, 9 | grplid 17452 |
. . . . . . . . . . . 12
        
                           |
| 36 | 34, 29, 35 | syl2anc 693 |
. . . . . . . . . . 11
         oGrp oGrp                                                       |
| 37 | | nncn 11028 |
. . . . . . . . . . . . . . 15
          |
| 38 | | ax-1cn 9994 |
. . . . . . . . . . . . . . 15
    |
| 39 | | addcom 10222 |
. . . . . . . . . . . . . . 15
        
               |
| 40 | 37, 38, 39 | sylancl 694 |
. . . . . . . . . . . . . 14
                  |
| 41 | 40 | oveq1d 6665 |
. . . . . . . . . . . . 13
                          |
| 42 | 16, 41 | syl 17 |
. . . . . . . . . . . 12
         oGrp oGrp                                                       |
| 43 | | grpsgrp 17446 |
. . . . . . . . . . . . . 14
        SGrp SGrp |
| 44 | 19, 20, 43 | 3syl 18 |
. . . . . . . . . . . . 13
         oGrp oGrp                                     SGrp
SGrp |
| 45 | | 1nn 11031 |
. . . . . . . . . . . . . 14
    |
| 46 | 45 | a1i 11 |
. . . . . . . . . . . . 13
         oGrp oGrp                                       |
| 47 | 8, 10, 31 | mulgnndir 17569 |
. . . . . . . . . . . . 13
     SGrp SGrp        
                                    |
| 48 | 44, 46, 16, 24, 47 | syl13anc 1328 |
. . . . . . . . . . . 12
         oGrp oGrp                                                               |
| 49 | 8, 10 | mulg1 17548 |
. . . . . . . . . . . . . 14
        
     |
| 50 | 24, 49 | syl 17 |
. . . . . . . . . . . . 13
         oGrp oGrp                                           |
| 51 | 50 | oveq1d 6665 |
. . . . . . . . . . . 12
         oGrp oGrp                                                                   |
| 52 | 42, 48, 51 | 3eqtrrd 2661 |
. . . . . . . . . . 11
         oGrp oGrp                                                           |
| 53 | 33, 36, 52 | 3brtr3d 4684 |
. . . . . . . . . 10
         oGrp oGrp                                                   |
| 54 | | tospos 29658 |
. . . . . . . . . . . . 13
    Toset Toset      |
| 55 | 18, 4, 54 | 3syl 18 |
. . . . . . . . . . . 12
        oGrp oGrp 
                        |
| 56 | | simpllr 799 |
. . . . . . . . . . . 12
        oGrp oGrp 
                        |
| 57 | 26 | peano2zd 11485 |
. . . . . . . . . . . . 13
        oGrp oGrp 
                            |
| 58 | 8, 10 | mulgcl 17559 |
. . . . . . . . . . . . 13
        
       
               |
| 59 | 25, 57, 23, 58 | syl3anc 1326 |
. . . . . . . . . . . 12
        oGrp oGrp 
                                |
| 60 | 8, 11, 12 | plelttr 16972 |
. . . . . . . . . . . 12
                                    
                           |
| 61 | 55, 56, 28, 59, 60 | syl13anc 1328 |
. . . . . . . . . . 11
        oGrp oGrp 
                                                  
             |
| 62 | 61 | impl 650 |
. . . . . . . . . 10
          oGrp oGrp   
   
   
    
   
               
                           |
| 63 | 53, 62 | mpdan 702 |
. . . . . . . . 9
         oGrp oGrp                                               |
| 64 | | oveq1 6657 |
. . . . . . . . . . 11
            
             |
| 65 | 64 | breq2d 4665 |
. . . . . . . . . 10
                  
             |
| 66 | 65 | rspcev 3309 |
. . . . . . . . 9
                         
          |
| 67 | 17, 63, 66 | syl2anc 693 |
. . . . . . . 8
         oGrp oGrp                                    
          |
| 68 | 67 | r19.29an 3077 |
. . . . . . 7
        oGrp oGrp 
                 
               
          |
| 69 | | oveq1 6657 |
. . . . . . . . 9
        
         |
| 70 | 69 | breq2d 4665 |
. . . . . . . 8
              
         |
| 71 | 70 | cbvrexv 3172 |
. . . . . . 7
                          |
| 72 | 68, 71 | sylib 208 |
. . . . . 6
        oGrp oGrp 
                 
               
          |
| 73 | 11, 12 | pltle 16961 |
. . . . . . . . 9
     oGrp oGrp    
   
                            |
| 74 | 18, 56, 28, 73 | syl3anc 1326 |
. . . . . . . 8
        oGrp oGrp 
                                          |
| 75 | 74 | reximdva 3017 |
. . . . . . 7
       oGrp oGrp  
   
            
                           |
| 76 | 75 | imp 445 |
. . . . . 6
        oGrp oGrp 
                 
                          |
| 77 | 72, 76 | impbida 877 |
. . . . 5
       oGrp oGrp  
   
            
             
        
    |
| 78 | 77 | pm5.74da 723 |
. . . 4
      oGrp oGrp   
   
   
                                          |
| 79 | 78 | ralbidva 2985 |
. . 3
     oGrp oGrp         
    
                  
   
                   |
| 80 | 79 | ralbidva 2985 |
. 2
    oGrp oGrp      
   
                       
   
    
                |
| 81 | 14, 80 | bitrd 268 |
1
    oGrp oGrp     Archi Archi        
              
     |