Theorem znf1o 19900

Description: The function F enumerates all equivalence classes in ℤ/nℤ for each n. When n = 0, ZZ / 0 ZZ = ZZ / { 0 } ~~ ZZ so we let W = ZZ; otherwise W = { 0 , ... , n - 1 } enumerates all the equivalence classes. (Contributed by Mario Carneiro, 15-Jun-2015.) (Revised by Mario Carneiro, 2-May-2016.) (Revised by AV, 13-Jun-2019.)

Hypotheses

Ref	Expression
znf1o.y	|- Y = (ℤ/nℤ ` N )
znf1o.b	|- B = ( Base ` Y )
znf1o.f	|- F = ( ( ZRHom ` Y ) |` W )
znf1o.w	|- W = if ( N = 0 , ZZ , ( 0..^ N ) )

Assertion

Ref	Expression
znf1o	|- ( N e. NN0 -> F : W -1-1-onto-> B )

Proof of Theorem znf1o

Dummy variables x y z are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		znf1o.y	. . . . . . 7 |- Y = (ℤ/nℤ ` N )
2	1	zncrng 19893	. . . . . 6 |- ( N e. NN0 -> Y e. CRing )
3		crngring 18558	. . . . . 6 |- ( Y e. CRing -> Y e. Ring )
4		eqid 2622	. . . . . . 7 |- ( ZRHom ` Y ) = ( ZRHom ` Y )
5	4	zrhrhm 19860	. . . . . 6 |- ( Y e. Ring -> ( ZRHom ` Y ) e. (ℤring RingHom Y ) )
6		zringbas 19824	. . . . . . 7 |- ZZ = ( Base ` ℤring )
7		znf1o.b	. . . . . . 7 |- B = ( Base ` Y )
8	6, 7	rhmf 18726	. . . . . 6 |- ( ( ZRHom ` Y ) e. (ℤring RingHom Y ) -> ( ZRHom ` Y ) : ZZ --> B )
9	2, 3, 5, 8	4syl 19	. . . . 5 |- ( N e. NN0 -> ( ZRHom ` Y ) : ZZ --> B )
10		znf1o.w	. . . . . 6 |- W = if ( N = 0 , ZZ , ( 0..^ N ) )
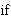11		sseq1 3626	. . . . . . 7 |- ( ZZ = if ( N = 0 , ZZ , ( 0..^ N ) ) -> ( ZZ C_ ZZ <-> if ( N = 0 , ZZ , ( 0..^ N ) ) C_ ZZ ) )
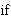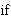
12		sseq1 3626	. . . . . . 7 |- ( ( 0..^ N ) = if ( N = 0 , ZZ , ( 0..^ N ) ) -> ( ( 0..^ N ) C_ ZZ <-> if ( N = 0 , ZZ , ( 0..^ N ) ) C_ ZZ ) )
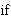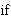13		ssid 3624	. . . . . . 7 |- ZZ C_ ZZ
14		elfzoelz 12470	. . . . . . . 8 |- ( x e. ( 0..^ N ) -> x e. ZZ )
15	14	ssriv 3607	. . . . . . 7 |- ( 0..^ N ) C_ ZZ
16	11, 12, 13, 15	keephyp 4152	. . . . . 6 |- if ( N = 0 , ZZ , ( 0..^ N ) ) C_ ZZ
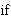17	10, 16	eqsstri 3635	. . . . 5 |- W C_ ZZ
18		fssres 6070	. . . . 5 |- ( ( ( ZRHom ` Y ) : ZZ --> B /\ W C_ ZZ ) -> ( ( ZRHom ` Y ) |` W ) : W --> B )
19	9, 17, 18	sylancl 694	. . . 4 |- ( N e. NN0 -> ( ( ZRHom ` Y ) |` W ) : W --> B )
20		znf1o.f	. . . . 5 |- F = ( ( ZRHom ` Y ) |` W )
21	20	feq1i 6036	. . . 4 |- ( F : W --> B <-> ( ( ZRHom ` Y ) |` W ) : W --> B )
22	19, 21	sylibr 224	. . 3 |- ( N e. NN0 -> F : W --> B )
23	20	fveq1i 6192	. . . . . . . 8 |- ( F ` x ) = ( ( ( ZRHom ` Y ) |` W ) ` x )
24		fvres 6207	. . . . . . . . 9 |- ( x e. W -> ( ( ( ZRHom ` Y ) |` W ) ` x ) = ( ( ZRHom ` Y ) ` x ) )
25	24	ad2antrl 764	. . . . . . . 8 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( ( ( ZRHom ` Y ) |` W ) ` x ) = ( ( ZRHom ` Y ) ` x ) )
26	23, 25	syl5eq 2668	. . . . . . 7 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( F ` x ) = ( ( ZRHom ` Y ) ` x ) )
27	20	fveq1i 6192	. . . . . . . 8 |- ( F ` y ) = ( ( ( ZRHom ` Y ) |` W ) ` y )
28		fvres 6207	. . . . . . . . 9 |- ( y e. W -> ( ( ( ZRHom ` Y ) |` W ) ` y ) = ( ( ZRHom ` Y ) ` y ) )
29	28	ad2antll 765	. . . . . . . 8 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( ( ( ZRHom ` Y ) |` W ) ` y ) = ( ( ZRHom ` Y ) ` y ) )
30	27, 29	syl5eq 2668	. . . . . . 7 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( F ` y ) = ( ( ZRHom ` Y ) ` y ) )
31	26, 30	eqeq12d 2637	. . . . . 6 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( ( F ` x ) = ( F ` y ) <-> ( ( ZRHom ` Y ) ` x ) = ( ( ZRHom ` Y ) ` y ) ) )
32		simpl 473	. . . . . . 7 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> N e. NN0 )
33		simprl 794	. . . . . . . 8 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> x e. W )
34	17, 33	sseldi 3601	. . . . . . 7 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> x e. ZZ )
35		simprr 796	. . . . . . . 8 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> y e. W )
36	17, 35	sseldi 3601	. . . . . . 7 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> y e. ZZ )
37	1, 4	zndvds 19898	. . . . . . 7 |- ( ( N e. NN0 /\ x e. ZZ /\ y e. ZZ ) -> ( ( ( ZRHom ` Y ) ` x ) = ( ( ZRHom ` Y ) ` y ) <-> N || ( x - y ) ) )
38	32, 34, 36, 37	syl3anc 1326	. . . . . 6 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( ( ( ZRHom ` Y ) ` x ) = ( ( ZRHom ` Y ) ` y ) <-> N || ( x - y ) ) )
39		elnn0 11294	. . . . . . 7 |- ( N e. NN0 <-> ( N e. NN \/ N = 0 ) )
40		simpl 473	. . . . . . . . . 10 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> N e. NN )
41		simprl 794	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> x e. W )
42	17, 41	sseldi 3601	. . . . . . . . . 10 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> x e. ZZ )
43		simprr 796	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> y e. W )
44	17, 43	sseldi 3601	. . . . . . . . . 10 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> y e. ZZ )
45		moddvds 14991	. . . . . . . . . 10 |- ( ( N e. NN /\ x e. ZZ /\ y e. ZZ ) -> ( ( x mod N ) = ( y mod N ) <-> N || ( x - y ) ) )
46	40, 42, 44, 45	syl3anc 1326	. . . . . . . . 9 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> ( ( x mod N ) = ( y mod N ) <-> N || ( x - y ) ) )
47	42	zred 11482	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> x e. RR )
48		nnrp 11842	. . . . . . . . . . . 12 |- ( N e. NN -> N e. RR+ )
49	48	adantr 481	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> N e. RR+ )
50		nnne0 11053	. . . . . . . . . . . . . . . 16 |- ( N e. NN -> N =/= 0 )
51		ifnefalse 4098	. . . . . . . . . . . . . . . 16 |- ( N =/= 0 -> if ( N = 0 , ZZ , ( 0..^ N ) ) = ( 0..^ N ) )
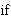
52	50, 51	syl 17	. . . . . . . . . . . . . . 15 |- ( N e. NN -> if ( N = 0 , ZZ , ( 0..^ N ) ) = ( 0..^ N ) )
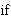
53	10, 52	syl5eq 2668	. . . . . . . . . . . . . 14 |- ( N e. NN -> W = ( 0..^ N ) )
54	53	adantr 481	. . . . . . . . . . . . 13 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> W = ( 0..^ N ) )
55	41, 54	eleqtrd 2703	. . . . . . . . . . . 12 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> x e. ( 0..^ N ) )
56		elfzole1 12478	. . . . . . . . . . . 12 |- ( x e. ( 0..^ N ) -> 0 <_ x )
57	55, 56	syl 17	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> 0 <_ x )
58		elfzolt2 12479	. . . . . . . . . . . 12 |- ( x e. ( 0..^ N ) -> x < N )
59	55, 58	syl 17	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> x < N )
60		modid 12695	. . . . . . . . . . 11 |- ( ( ( x e. RR /\ N e. RR+ ) /\ ( 0 <_ x /\ x < N ) ) -> ( x mod N ) = x )
61	47, 49, 57, 59, 60	syl22anc 1327	. . . . . . . . . 10 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> ( x mod N ) = x )
62	44	zred 11482	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> y e. RR )
63	43, 54	eleqtrd 2703	. . . . . . . . . . . 12 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> y e. ( 0..^ N ) )
64		elfzole1 12478	. . . . . . . . . . . 12 |- ( y e. ( 0..^ N ) -> 0 <_ y )
65	63, 64	syl 17	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> 0 <_ y )
66		elfzolt2 12479	. . . . . . . . . . . 12 |- ( y e. ( 0..^ N ) -> y < N )
67	63, 66	syl 17	. . . . . . . . . . 11 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> y < N )
68		modid 12695	. . . . . . . . . . 11 |- ( ( ( y e. RR /\ N e. RR+ ) /\ ( 0 <_ y /\ y < N ) ) -> ( y mod N ) = y )
69	62, 49, 65, 67, 68	syl22anc 1327	. . . . . . . . . 10 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> ( y mod N ) = y )
70	61, 69	eqeq12d 2637	. . . . . . . . 9 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> ( ( x mod N ) = ( y mod N ) <-> x = y ) )
71	46, 70	bitr3d 270	. . . . . . . 8 |- ( ( N e. NN /\ ( x e. W /\ y e. W ) ) -> ( N || ( x - y ) <-> x = y ) )
72		simpl 473	. . . . . . . . . 10 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> N = 0 )
73	72	breq1d 4663	. . . . . . . . 9 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> ( N || ( x - y ) <-> 0 || ( x - y ) ) )
74		id 22	. . . . . . . . . . . . 13 |- ( N = 0 -> N = 0 )
75		0nn0 11307	. . . . . . . . . . . . 13 |- 0 e. NN0
76	74, 75	syl6eqel 2709	. . . . . . . . . . . 12 |- ( N = 0 -> N e. NN0 )
77	76, 34	sylan 488	. . . . . . . . . . 11 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> x e. ZZ )
78	76, 36	sylan 488	. . . . . . . . . . 11 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> y e. ZZ )
79	77, 78	zsubcld 11487	. . . . . . . . . 10 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> ( x - y ) e. ZZ )
80		0dvds 15002	. . . . . . . . . 10 |- ( ( x - y ) e. ZZ -> ( 0 || ( x - y ) <-> ( x - y ) = 0 ) )
81	79, 80	syl 17	. . . . . . . . 9 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> ( 0 || ( x - y ) <-> ( x - y ) = 0 ) )
82	77	zcnd 11483	. . . . . . . . . 10 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> x e. CC )
83	78	zcnd 11483	. . . . . . . . . 10 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> y e. CC )
84	82, 83	subeq0ad 10402	. . . . . . . . 9 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> ( ( x - y ) = 0 <-> x = y ) )
85	73, 81, 84	3bitrd 294	. . . . . . . 8 |- ( ( N = 0 /\ ( x e. W /\ y e. W ) ) -> ( N || ( x - y ) <-> x = y ) )
86	71, 85	jaoian 824	. . . . . . 7 |- ( ( ( N e. NN \/ N = 0 ) /\ ( x e. W /\ y e. W ) ) -> ( N || ( x - y ) <-> x = y ) )
87	39, 86	sylanb 489	. . . . . 6 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( N || ( x - y ) <-> x = y ) )
88	31, 38, 87	3bitrd 294	. . . . 5 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( ( F ` x ) = ( F ` y ) <-> x = y ) )
89	88	biimpd 219	. . . 4 |- ( ( N e. NN0 /\ ( x e. W /\ y e. W ) ) -> ( ( F ` x ) = ( F ` y ) -> x = y ) )
90	89	ralrimivva 2971	. . 3 |- ( N e. NN0 -> A. x e. W A. y e. W ( ( F ` x ) = ( F ` y ) -> x = y ) )
91		dff13 6512	. . 3 |- ( F : W -1-1-> B <-> ( F : W --> B /\ A. x e. W A. y e. W ( ( F ` x ) = ( F ` y ) -> x = y ) ) )
92	22, 90, 91	sylanbrc 698	. 2 |- ( N e. NN0 -> F : W -1-1-> B )
93		zmodfzo 12693	. . . . . . . . . . . 12 |- ( ( z e. ZZ /\ N e. NN ) -> ( z mod N ) e. ( 0..^ N ) )
94	93	ancoms 469	. . . . . . . . . . 11 |- ( ( N e. NN /\ z e. ZZ ) -> ( z mod N ) e. ( 0..^ N ) )
95	53	adantr 481	. . . . . . . . . . 11 |- ( ( N e. NN /\ z e. ZZ ) -> W = ( 0..^ N ) )
96	94, 95	eleqtrrd 2704	. . . . . . . . . 10 |- ( ( N e. NN /\ z e. ZZ ) -> ( z mod N ) e. W )
97		zre 11381	. . . . . . . . . . . . . 14 |- ( z e. ZZ -> z e. RR )
98		modabs2 12704	. . . . . . . . . . . . . 14 |- ( ( z e. RR /\ N e. RR+ ) -> ( ( z mod N ) mod N ) = ( z mod N ) )
99	97, 48, 98	syl2anr 495	. . . . . . . . . . . . 13 |- ( ( N e. NN /\ z e. ZZ ) -> ( ( z mod N ) mod N ) = ( z mod N ) )
100		simpl 473	. . . . . . . . . . . . . 14 |- ( ( N e. NN /\ z e. ZZ ) -> N e. NN )
101	15, 94	sseldi 3601	. . . . . . . . . . . . . 14 |- ( ( N e. NN /\ z e. ZZ ) -> ( z mod N ) e. ZZ )
102		simpr 477	. . . . . . . . . . . . . 14 |- ( ( N e. NN /\ z e. ZZ ) -> z e. ZZ )
103		moddvds 14991	. . . . . . . . . . . . . 14 |- ( ( N e. NN /\ ( z mod N ) e. ZZ /\ z e. ZZ ) -> ( ( ( z mod N ) mod N ) = ( z mod N ) <-> N || ( ( z mod N ) - z ) ) )
104	100, 101, 102, 103	syl3anc 1326	. . . . . . . . . . . . 13 |- ( ( N e. NN /\ z e. ZZ ) -> ( ( ( z mod N ) mod N ) = ( z mod N ) <-> N || ( ( z mod N ) - z ) ) )
105	99, 104	mpbid 222	. . . . . . . . . . . 12 |- ( ( N e. NN /\ z e. ZZ ) -> N || ( ( z mod N ) - z ) )
106		nnnn0 11299	. . . . . . . . . . . . . 14 |- ( N e. NN -> N e. NN0 )
107	106	adantr 481	. . . . . . . . . . . . 13 |- ( ( N e. NN /\ z e. ZZ ) -> N e. NN0 )
108	1, 4	zndvds 19898	. . . . . . . . . . . . 13 |- ( ( N e. NN0 /\ ( z mod N ) e. ZZ /\ z e. ZZ ) -> ( ( ( ZRHom ` Y ) ` ( z mod N ) ) = ( ( ZRHom ` Y ) ` z ) <-> N || ( ( z mod N ) - z ) ) )
109	107, 101, 102, 108	syl3anc 1326	. . . . . . . . . . . 12 |- ( ( N e. NN /\ z e. ZZ ) -> ( ( ( ZRHom ` Y ) ` ( z mod N ) ) = ( ( ZRHom ` Y ) ` z ) <-> N || ( ( z mod N ) - z ) ) )
110	105, 109	mpbird 247	. . . . . . . . . . 11 |- ( ( N e. NN /\ z e. ZZ ) -> ( ( ZRHom ` Y ) ` ( z mod N ) ) = ( ( ZRHom ` Y ) ` z ) )
111	110	eqcomd 2628	. . . . . . . . . 10 |- ( ( N e. NN /\ z e. ZZ ) -> ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` ( z mod N ) ) )
112		fveq2 6191	. . . . . . . . . . . 12 |- ( y = ( z mod N ) -> ( ( ZRHom ` Y ) ` y ) = ( ( ZRHom ` Y ) ` ( z mod N ) ) )
113	112	eqeq2d 2632	. . . . . . . . . . 11 |- ( y = ( z mod N ) -> ( ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) <-> ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` ( z mod N ) ) ) )
114	113	rspcev 3309	. . . . . . . . . 10 |- ( ( ( z mod N ) e. W /\ ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` ( z mod N ) ) ) -> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) )
115	96, 111, 114	syl2anc 693	. . . . . . . . 9 |- ( ( N e. NN /\ z e. ZZ ) -> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) )
116		iftrue 4092	. . . . . . . . . . . . 13 |- ( N = 0 -> if ( N = 0 , ZZ , ( 0..^ N ) ) = ZZ )
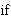
117	116	eleq2d 2687	. . . . . . . . . . . 12 |- ( N = 0 -> ( z e. if ( N = 0 , ZZ , ( 0..^ N ) ) <-> z e. ZZ ) )
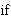118	117	biimpar 502	. . . . . . . . . . 11 |- ( ( N = 0 /\ z e. ZZ ) -> z e. if ( N = 0 , ZZ , ( 0..^ N ) ) )
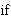
119	118, 10	syl6eleqr 2712	. . . . . . . . . 10 |- ( ( N = 0 /\ z e. ZZ ) -> z e. W )
120		eqidd 2623	. . . . . . . . . 10 |- ( ( N = 0 /\ z e. ZZ ) -> ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` z ) )
121		fveq2 6191	. . . . . . . . . . . 12 |- ( y = z -> ( ( ZRHom ` Y ) ` y ) = ( ( ZRHom ` Y ) ` z ) )
122	121	eqeq2d 2632	. . . . . . . . . . 11 |- ( y = z -> ( ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) <-> ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` z ) ) )
123	122	rspcev 3309	. . . . . . . . . 10 |- ( ( z e. W /\ ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` z ) ) -> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) )
124	119, 120, 123	syl2anc 693	. . . . . . . . 9 |- ( ( N = 0 /\ z e. ZZ ) -> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) )
125	115, 124	jaoian 824	. . . . . . . 8 |- ( ( ( N e. NN \/ N = 0 ) /\ z e. ZZ ) -> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) )
126	39, 125	sylanb 489	. . . . . . 7 |- ( ( N e. NN0 /\ z e. ZZ ) -> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) )
127	27, 28	syl5eq 2668	. . . . . . . . 9 |- ( y e. W -> ( F ` y ) = ( ( ZRHom ` Y ) ` y ) )
128	127	eqeq2d 2632	. . . . . . . 8 |- ( y e. W -> ( ( ( ZRHom ` Y ) ` z ) = ( F ` y ) <-> ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) ) )
129	128	rexbiia 3040	. . . . . . 7 |- ( E. y e. W ( ( ZRHom ` Y ) ` z ) = ( F ` y ) <-> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( ( ZRHom ` Y ) ` y ) )
130	126, 129	sylibr 224	. . . . . 6 |- ( ( N e. NN0 /\ z e. ZZ ) -> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( F ` y ) )
131	130	ralrimiva 2966	. . . . 5 |- ( N e. NN0 -> A. z e. ZZ E. y e. W ( ( ZRHom ` Y ) ` z ) = ( F ` y ) )
132	1, 7, 4	znzrhfo 19896	. . . . . 6 |- ( N e. NN0 -> ( ZRHom ` Y ) : ZZ -onto-> B )
133		fofn 6117	. . . . . 6 |- ( ( ZRHom ` Y ) : ZZ -onto-> B -> ( ZRHom ` Y ) Fn ZZ )
134		eqeq1 2626	. . . . . . . 8 |- ( x = ( ( ZRHom ` Y ) ` z ) -> ( x = ( F ` y ) <-> ( ( ZRHom ` Y ) ` z ) = ( F ` y ) ) )
135	134	rexbidv 3052	. . . . . . 7 |- ( x = ( ( ZRHom ` Y ) ` z ) -> ( E. y e. W x = ( F ` y ) <-> E. y e. W ( ( ZRHom ` Y ) ` z ) = ( F ` y ) ) )
136	135	ralrn 6362	. . . . . 6 |- ( ( ZRHom ` Y ) Fn ZZ -> ( A. x e. ran ( ZRHom ` Y ) E. y e. W x = ( F ` y ) <-> A. z e. ZZ E. y e. W ( ( ZRHom ` Y ) ` z ) = ( F ` y ) ) )
137	132, 133, 136	3syl 18	. . . . 5 |- ( N e. NN0 -> ( A. x e. ran ( ZRHom ` Y ) E. y e. W x = ( F ` y ) <-> A. z e. ZZ E. y e. W ( ( ZRHom ` Y ) ` z ) = ( F ` y ) ) )
138	131, 137	mpbird 247	. . . 4 |- ( N e. NN0 -> A. x e. ran ( ZRHom ` Y ) E. y e. W x = ( F ` y ) )
139		forn 6118	. . . . . 6 |- ( ( ZRHom ` Y ) : ZZ -onto-> B -> ran ( ZRHom ` Y ) = B )
140	132, 139	syl 17	. . . . 5 |- ( N e. NN0 -> ran ( ZRHom ` Y ) = B )
141	140	raleqdv 3144	. . . 4 |- ( N e. NN0 -> ( A. x e. ran ( ZRHom ` Y ) E. y e. W x = ( F ` y ) <-> A. x e. B E. y e. W x = ( F ` y ) ) )
142	138, 141	mpbid 222	. . 3 |- ( N e. NN0 -> A. x e. B E. y e. W x = ( F ` y ) )
143		dffo3 6374	. . 3 |- ( F : W -onto-> B <-> ( F : W --> B /\ A. x e. B E. y e. W x = ( F ` y ) ) )
144	22, 142, 143	sylanbrc 698	. 2 |- ( N e. NN0 -> F : W -onto-> B )
145		df-f1o 5895	. 2 |- ( F : W -1-1-onto-> B <-> ( F : W -1-1-> B /\ F : W -onto-> B ) )
146	92, 144, 145	sylanbrc 698	1 |- ( N e. NN0 -> F : W -1-1-onto-> B )

Syntax hints:   -> wi 4   <-> wb 196   \/ wo 383   /\ wa 384   = wceq 1483   e. wcel 1990   =/= wne 2794   A.wral 2912   E.wrex 2913   C_ wss 3574   ifcif 4086   class class class wbr 4653   ran crn 5115   |` cres 5116   Fn wfn 5883   -->wf 5884   -1-1->wf1 5885   -onto->wfo 5886   -1-1-onto->wf1o 5887   ` cfv 5888  (class class class)co 6650   RRcr 9935   0cc0 9936   < clt 10074   <_ cle 10075   - cmin 10266   NNcn 11020   NN0cn0 11292   ZZcz 11377   RR+crp 11832  ..^cfzo 12465   mod cmo 12668   || cdvds 14983   Basecbs 15857   Ringcrg 18547   CRingccrg 18548   RingHom crh 18712  ℤ_ringzring 19818   ZRHomczrh 19848  ℤ/nℤczn 19851

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-rep 4771  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949  ax-inf2 8538  ax-cnex 9992  ax-resscn 9993  ax-1cn 9994  ax-icn 9995  ax-addcl 9996  ax-addrcl 9997  ax-mulcl 9998  ax-mulrcl 9999  ax-mulcom 10000  ax-addass 10001  ax-mulass 10002  ax-distr 10003  ax-i2m1 10004  ax-1ne0 10005  ax-1rid 10006  ax-rnegex 10007  ax-rrecex 10008  ax-cnre 10009  ax-pre-lttri 10010  ax-pre-lttrn 10011  ax-pre-ltadd 10012  ax-pre-mulgt0 10013  ax-pre-sup 10014  ax-addf 10015  ax-mulf 10016

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3or 1038  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-nel 2898  df-ral 2917  df-rex 2918  df-reu 2919  df-rmo 2920  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-pss 3590  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-tp 4182  df-op 4184  df-uni 4437  df-int 4476  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-tr 4753  df-id 5024  df-eprel 5029  df-po 5035  df-so 5036  df-fr 5073  df-we 5075  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-pred 5680  df-ord 5726  df-on 5727  df-lim 5728  df-suc 5729  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-riota 6611  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-om 7066  df-1st 7168  df-2nd 7169  df-tpos 7352  df-wrecs 7407  df-recs 7468  df-rdg 7506  df-1o 7560  df-oadd 7564  df-er 7742  df-ec 7744  df-qs 7748  df-map 7859  df-en 7956  df-dom 7957  df-sdom 7958  df-fin 7959  df-sup 8348  df-inf 8349  df-pnf 10076  df-mnf 10077  df-xr 10078  df-ltxr 10079  df-le 10080  df-sub 10268  df-neg 10269  df-div 10685  df-nn 11021  df-2 11079  df-3 11080  df-4 11081  df-5 11082  df-6 11083  df-7 11084  df-8 11085  df-9 11086  df-n0 11293  df-z 11378  df-dec 11494  df-uz 11688  df-rp 11833  df-fz 12327  df-fzo 12466  df-fl 12593  df-mod 12669  df-seq 12802  df-dvds 14984  df-struct 15859  df-ndx 15860  df-slot 15861  df-base 15863  df-sets 15864  df-ress 15865  df-plusg 15954  df-mulr 15955  df-starv 15956  df-sca 15957  df-vsca 15958  df-ip 15959  df-tset 15960  df-ple 15961  df-ds 15964  df-unif 15965  df-0g 16102  df-imas 16168  df-qus 16169  df-mgm 17242  df-sgrp 17284  df-mnd 17295  df-mhm 17335  df-grp 17425  df-minusg 17426  df-sbg 17427  df-mulg 17541  df-subg 17591  df-nsg 17592  df-eqg 17593  df-ghm 17658  df-cmn 18195  df-abl 18196  df-mgp 18490  df-ur 18502  df-ring 18549  df-cring 18550  df-oppr 18623  df-dvdsr 18641  df-rnghom 18715  df-subrg 18778  df-lmod 18865  df-lss 18933  df-lsp 18972  df-sra 19172  df-rgmod 19173  df-lidl 19174  df-rsp 19175  df-2idl 19232  df-cnfld 19747  df-zring 19819  df-zrh 19852  df-zn 19855

This theorem is referenced by:  zzngim  19901  znleval  19903  zntoslem  19905  znhash  19907  znunithash  19913  dchrisumlem1  25178