Theorem frlmphl 20120

Description: Conditions for a free module to be a pre-Hilbert space. (Contributed by Thierry Arnoux, 21-Jun-2019.) (Proof shortened by AV, 21-Jul-2019.)

Hypotheses

Ref	Expression
frlmphl.y	|- Y = ( R freeLMod I )
frlmphl.b	|- B = ( Base ` R )
frlmphl.t	|- .x. = ( .r ` R )
frlmphl.v	|- V = ( Base ` Y )
frlmphl.j	|- ., = ( .i ` Y )
frlmphl.o	|- O = ( 0g ` Y )
frlmphl.0	|- .0. = ( 0g ` R )
frlmphl.s	|- .* = ( *r ` R )
frlmphl.f	|- ( ph -> R e. Field )
frlmphl.m	|- ( ( ph /\ g e. V /\ ( g ., g ) = .0. ) -> g = O )
frlmphl.u	|- ( ( ph /\ x e. B ) -> ( .* ` x ) = x )
frlmphl.i	|- ( ph -> I e. W )

Assertion

Ref	Expression
frlmphl	|- ( ph -> Y e. PreHil )

Distinct variable groups:   B, g, x   g, I, x   R, g, x   g, V, x   g, W, x   .x. , g, x   g, Y, x   .0. , g, x   ph, g, x   ., , g, x   g, O   x, .*

Allowed substitution hints:   .* ( g)   O( x)

Proof of Theorem frlmphl

Dummy variables f e h i y z k are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		frlmphl.v	. . 3 |- V = ( Base ` Y )
2	1	a1i 11	. 2 |- ( ph -> V = ( Base ` Y ) )
3		eqidd 2623	. 2 |- ( ph -> ( +g ` Y ) = ( +g ` Y ) )
4		eqidd 2623	. 2 |- ( ph -> ( .s ` Y ) = ( .s ` Y ) )
5		frlmphl.j	. . 3 |- ., = ( .i ` Y )
6	5	a1i 11	. 2 |- ( ph -> ., = ( .i ` Y ) )
7		frlmphl.o	. . 3 |- O = ( 0g ` Y )
8	7	a1i 11	. 2 |- ( ph -> O = ( 0g ` Y ) )
9		frlmphl.f	. . . . 5 |- ( ph -> R e. Field )
10		isfld 18756	. . . . 5 |- ( R e. Field <-> ( R e. DivRing /\ R e. CRing ) )
11	9, 10	sylib 208	. . . 4 |- ( ph -> ( R e. DivRing /\ R e. CRing ) )
12	11	simpld 475	. . 3 |- ( ph -> R e. DivRing )
13		frlmphl.i	. . 3 |- ( ph -> I e. W )
14		frlmphl.y	. . . 4 |- Y = ( R freeLMod I )
15	14	frlmsca 20097	. . 3 |- ( ( R e. DivRing /\ I e. W ) -> R = (Scalar ` Y ) )
16	12, 13, 15	syl2anc 693	. 2 |- ( ph -> R = (Scalar ` Y ) )
17		frlmphl.b	. . 3 |- B = ( Base ` R )
18	17	a1i 11	. 2 |- ( ph -> B = ( Base ` R ) )
19		eqidd 2623	. 2 |- ( ph -> ( +g ` R ) = ( +g ` R ) )
20		frlmphl.t	. . 3 |- .x. = ( .r ` R )
21	20	a1i 11	. 2 |- ( ph -> .x. = ( .r ` R ) )
22		frlmphl.s	. . 3 |- .* = ( *r ` R )
23	22	a1i 11	. 2 |- ( ph -> .* = ( *r ` R ) )
24		frlmphl.0	. . 3 |- .0. = ( 0g ` R )
25	24	a1i 11	. 2 |- ( ph -> .0. = ( 0g ` R ) )
26		drngring 18754	. . . . 5 |- ( R e. DivRing -> R e. Ring )
27	12, 26	syl 17	. . . 4 |- ( ph -> R e. Ring )
28	14	frlmlmod 20093	. . . 4 |- ( ( R e. Ring /\ I e. W ) -> Y e. LMod )
29	27, 13, 28	syl2anc 693	. . 3 |- ( ph -> Y e. LMod )
30	16, 12	eqeltrrd 2702	. . 3 |- ( ph -> (Scalar ` Y ) e. DivRing )
31		eqid 2622	. . . 4 |- (Scalar ` Y ) = (Scalar ` Y )
32	31	islvec 19104	. . 3 |- ( Y e. LVec <-> ( Y e. LMod /\ (Scalar ` Y ) e. DivRing ) )
33	29, 30, 32	sylanbrc 698	. 2 |- ( ph -> Y e. LVec )
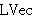34	11	simprd 479	. . 3 |- ( ph -> R e. CRing )
35		frlmphl.u	. . 3 |- ( ( ph /\ x e. B ) -> ( .* ` x ) = x )
36	17, 22, 34, 35	idsrngd 18862	. 2 |- ( ph -> R e. *Ring )
37	13	3ad2ant1 1082	. . . . 5 |- ( ( ph /\ g e. V /\ h e. V ) -> I e. W )
38	27	3ad2ant1 1082	. . . . 5 |- ( ( ph /\ g e. V /\ h e. V ) -> R e. Ring )
39		simp2 1062	. . . . 5 |- ( ( ph /\ g e. V /\ h e. V ) -> g e. V )
40		simp3 1063	. . . . 5 |- ( ( ph /\ g e. V /\ h e. V ) -> h e. V )
41	14, 17, 20, 1, 5	frlmipval 20118	. . . . 5 |- ( ( ( I e. W /\ R e. Ring ) /\ ( g e. V /\ h e. V ) ) -> ( g ., h ) = ( R gsumg ( g oF .x. h ) ) )
42	37, 38, 39, 40, 41	syl22anc 1327	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ( g ., h ) = ( R gsumg ( g oF .x. h ) ) )
43	14, 17, 1	frlmbasmap 20103	. . . . . . . . 9 |- ( ( I e. W /\ g e. V ) -> g e. ( B ^m I ) )
44	37, 39, 43	syl2anc 693	. . . . . . . 8 |- ( ( ph /\ g e. V /\ h e. V ) -> g e. ( B ^m I ) )
45		elmapi 7879	. . . . . . . 8 |- ( g e. ( B ^m I ) -> g : I --> B )
46	44, 45	syl 17	. . . . . . 7 |- ( ( ph /\ g e. V /\ h e. V ) -> g : I --> B )
47		ffn 6045	. . . . . . 7 |- ( g : I --> B -> g Fn I )
48	46, 47	syl 17	. . . . . 6 |- ( ( ph /\ g e. V /\ h e. V ) -> g Fn I )
49	14, 17, 1	frlmbasmap 20103	. . . . . . . . 9 |- ( ( I e. W /\ h e. V ) -> h e. ( B ^m I ) )
50	37, 40, 49	syl2anc 693	. . . . . . . 8 |- ( ( ph /\ g e. V /\ h e. V ) -> h e. ( B ^m I ) )
51		elmapi 7879	. . . . . . . 8 |- ( h e. ( B ^m I ) -> h : I --> B )
52	50, 51	syl 17	. . . . . . 7 |- ( ( ph /\ g e. V /\ h e. V ) -> h : I --> B )
53		ffn 6045	. . . . . . 7 |- ( h : I --> B -> h Fn I )
54	52, 53	syl 17	. . . . . 6 |- ( ( ph /\ g e. V /\ h e. V ) -> h Fn I )
55		inidm 3822	. . . . . 6 |- ( I i^i I ) = I
56		eqidd 2623	. . . . . 6 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> ( g ` x ) = ( g ` x ) )
57		eqidd 2623	. . . . . 6 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> ( h ` x ) = ( h ` x ) )
58	48, 54, 37, 37, 55, 56, 57	offval 6904	. . . . 5 |- ( ( ph /\ g e. V /\ h e. V ) -> ( g oF .x. h ) = ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) )
59	58	oveq2d 6666	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ( R gsumg ( g oF .x. h ) ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) )
60	42, 59	eqtrd 2656	. . 3 |- ( ( ph /\ g e. V /\ h e. V ) -> ( g ., h ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) )
61		ringcmn 18581	. . . . . 6 |- ( R e. Ring -> R e. CMnd )
62	27, 61	syl 17	. . . . 5 |- ( ph -> R e. CMnd )
63	62	3ad2ant1 1082	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> R e. CMnd )
64	38	adantr 481	. . . . . 6 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> R e. Ring )
65	46	ffvelrnda 6359	. . . . . 6 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> ( g ` x ) e. B )
66	52	ffvelrnda 6359	. . . . . 6 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> ( h ` x ) e. B )
67	17, 20	ringcl 18561	. . . . . 6 |- ( ( R e. Ring /\ ( g ` x ) e. B /\ ( h ` x ) e. B ) -> ( ( g ` x ) .x. ( h ` x ) ) e. B )
68	64, 65, 66, 67	syl3anc 1326	. . . . 5 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> ( ( g ` x ) .x. ( h ` x ) ) e. B )
69		eqid 2622	. . . . 5 |- ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) = ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) )
70	68, 69	fmptd 6385	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) : I --> B )
71		frlmphl.m	. . . . 5 |- ( ( ph /\ g e. V /\ ( g ., g ) = .0. ) -> g = O )
72	14, 17, 20, 1, 5, 7, 24, 22, 9, 71, 35, 13	frlmphllem 20119	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) finSupp .0. )
73	17, 24, 63, 37, 70, 72	gsumcl 18316	. . 3 |- ( ( ph /\ g e. V /\ h e. V ) -> ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) e. B )
74	60, 73	eqeltrd 2701	. 2 |- ( ( ph /\ g e. V /\ h e. V ) -> ( g ., h ) e. B )
75		eqid 2622	. . . 4 |- ( +g ` R ) = ( +g ` R )
76	62	3ad2ant1 1082	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> R e. CMnd )
77	13	3ad2ant1 1082	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> I e. W )
78	27	3ad2ant1 1082	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> R e. Ring )
79	78	adantr 481	. . . . 5 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> R e. Ring )
80		simp2 1062	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> k e. B )
81	80	adantr 481	. . . . 5 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> k e. B )
82		simp31 1097	. . . . . . . . 9 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> g e. V )
83	77, 82, 43	syl2anc 693	. . . . . . . 8 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> g e. ( B ^m I ) )
84	83, 45	syl 17	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> g : I --> B )
85	84	ffvelrnda 6359	. . . . . 6 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( g ` x ) e. B )
86		simp33 1099	. . . . . . . . 9 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> i e. V )
87	14, 17, 1	frlmbasmap 20103	. . . . . . . . 9 |- ( ( I e. W /\ i e. V ) -> i e. ( B ^m I ) )
88	77, 86, 87	syl2anc 693	. . . . . . . 8 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> i e. ( B ^m I ) )
89		elmapi 7879	. . . . . . . 8 |- ( i e. ( B ^m I ) -> i : I --> B )
90	88, 89	syl 17	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> i : I --> B )
91	90	ffvelrnda 6359	. . . . . 6 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( i ` x ) e. B )
92	17, 20	ringcl 18561	. . . . . 6 |- ( ( R e. Ring /\ ( g ` x ) e. B /\ ( i ` x ) e. B ) -> ( ( g ` x ) .x. ( i ` x ) ) e. B )
93	79, 85, 91, 92	syl3anc 1326	. . . . 5 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( g ` x ) .x. ( i ` x ) ) e. B )
94	17, 20	ringcl 18561	. . . . 5 |- ( ( R e. Ring /\ k e. B /\ ( ( g ` x ) .x. ( i ` x ) ) e. B ) -> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) e. B )
95	79, 81, 93, 94	syl3anc 1326	. . . 4 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) e. B )
96		simp32 1098	. . . . . . . 8 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> h e. V )
97	77, 96, 49	syl2anc 693	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> h e. ( B ^m I ) )
98	97, 51	syl 17	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> h : I --> B )
99	98	ffvelrnda 6359	. . . . 5 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( h ` x ) e. B )
100	17, 20	ringcl 18561	. . . . 5 |- ( ( R e. Ring /\ ( h ` x ) e. B /\ ( i ` x ) e. B ) -> ( ( h ` x ) .x. ( i ` x ) ) e. B )
101	79, 99, 91, 100	syl3anc 1326	. . . 4 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( h ` x ) .x. ( i ` x ) ) e. B )
102		eqidd 2623	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) = ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) )
103		eqidd 2623	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) = ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) )
104		fveq2 6191	. . . . . . . . 9 |- ( x = y -> ( g ` x ) = ( g ` y ) )
105	104	oveq2d 6666	. . . . . . . 8 |- ( x = y -> ( k .x. ( g ` x ) ) = ( k .x. ( g ` y ) ) )
106	105	cbvmptv 4750	. . . . . . 7 |- ( x e. I |-> ( k .x. ( g ` x ) ) ) = ( y e. I |-> ( k .x. ( g ` y ) ) )
107	106	oveq1i 6660	. . . . . 6 |- ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) = ( ( y e. I |-> ( k .x. ( g ` y ) ) ) oF .x. i )
108	17, 20	ringcl 18561	. . . . . . . . . . . 12 |- ( ( R e. Ring /\ k e. B /\ ( g ` x ) e. B ) -> ( k .x. ( g ` x ) ) e. B )
109	79, 81, 85, 108	syl3anc 1326	. . . . . . . . . . 11 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( k .x. ( g ` x ) ) e. B )
110		eqid 2622	. . . . . . . . . . 11 |- ( x e. I |-> ( k .x. ( g ` x ) ) ) = ( x e. I |-> ( k .x. ( g ` x ) ) )
111	109, 110	fmptd 6385	. . . . . . . . . 10 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( k .x. ( g ` x ) ) ) : I --> B )
112		ffn 6045	. . . . . . . . . 10 |- ( ( x e. I |-> ( k .x. ( g ` x ) ) ) : I --> B -> ( x e. I |-> ( k .x. ( g ` x ) ) ) Fn I )
113	111, 112	syl 17	. . . . . . . . 9 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( k .x. ( g ` x ) ) ) Fn I )
114	106	fneq1i 5985	. . . . . . . . 9 |- ( ( x e. I |-> ( k .x. ( g ` x ) ) ) Fn I <-> ( y e. I |-> ( k .x. ( g ` y ) ) ) Fn I )
115	113, 114	sylib 208	. . . . . . . 8 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( y e. I |-> ( k .x. ( g ` y ) ) ) Fn I )
116		ffn 6045	. . . . . . . . 9 |- ( i : I --> B -> i Fn I )
117	90, 116	syl 17	. . . . . . . 8 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> i Fn I )
118		eqidd 2623	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( y e. I |-> ( k .x. ( g ` y ) ) ) = ( y e. I |-> ( k .x. ( g ` y ) ) ) )
119		simpr 477	. . . . . . . . . . 11 |- ( ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) /\ y = x ) -> y = x )
120	119	fveq2d 6195	. . . . . . . . . 10 |- ( ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) /\ y = x ) -> ( g ` y ) = ( g ` x ) )
121	120	oveq2d 6666	. . . . . . . . 9 |- ( ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) /\ y = x ) -> ( k .x. ( g ` y ) ) = ( k .x. ( g ` x ) ) )
122		simpr 477	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> x e. I )
123		ovexd 6680	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( k .x. ( g ` x ) ) e. _V )
124	118, 121, 122, 123	fvmptd 6288	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( y e. I |-> ( k .x. ( g ` y ) ) ) ` x ) = ( k .x. ( g ` x ) ) )
125		eqidd 2623	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( i ` x ) = ( i ` x ) )
126	115, 117, 77, 77, 55, 124, 125	offval 6904	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( y e. I |-> ( k .x. ( g ` y ) ) ) oF .x. i ) = ( x e. I |-> ( ( k .x. ( g ` x ) ) .x. ( i ` x ) ) ) )
127	17, 20	ringass 18564	. . . . . . . . 9 |- ( ( R e. Ring /\ ( k e. B /\ ( g ` x ) e. B /\ ( i ` x ) e. B ) ) -> ( ( k .x. ( g ` x ) ) .x. ( i ` x ) ) = ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) )
128	79, 81, 85, 91, 127	syl13anc 1328	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( k .x. ( g ` x ) ) .x. ( i ` x ) ) = ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) )
129	128	mpteq2dva 4744	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( ( k .x. ( g ` x ) ) .x. ( i ` x ) ) ) = ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) )
130	126, 129	eqtrd 2656	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( y e. I |-> ( k .x. ( g ` y ) ) ) oF .x. i ) = ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) )
131	107, 130	syl5eq 2668	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) = ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) )
132		ovexd 6680	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) e. _V )
133		funmpt 5926	. . . . . . 7 |- Fun ( z e. I |-> ( ( ( x e. I |-> ( k .x. ( g ` x ) ) ) ` z ) .x. ( i ` z ) ) )
134		eqidd 2623	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ z e. I ) -> ( ( x e. I |-> ( k .x. ( g ` x ) ) ) ` z ) = ( ( x e. I |-> ( k .x. ( g ` x ) ) ) ` z ) )
135		eqidd 2623	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ z e. I ) -> ( i ` z ) = ( i ` z ) )
136	113, 117, 77, 77, 55, 134, 135	offval 6904	. . . . . . . 8 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) = ( z e. I |-> ( ( ( x e. I |-> ( k .x. ( g ` x ) ) ) ` z ) .x. ( i ` z ) ) ) )
137	136	funeqd 5910	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( Fun ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) <-> Fun ( z e. I |-> ( ( ( x e. I |-> ( k .x. ( g ` x ) ) ) ` z ) .x. ( i ` z ) ) ) ) )
138	133, 137	mpbiri 248	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> Fun ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) )
139		simp3 1063	. . . . . . . . 9 |- ( ( g e. V /\ h e. V /\ i e. V ) -> i e. V )
140	13, 139	anim12i 590	. . . . . . . 8 |- ( ( ph /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( I e. W /\ i e. V ) )
141	140	3adant2 1080	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( I e. W /\ i e. V ) )
142	14, 24, 1	frlmbasfsupp 20102	. . . . . . 7 |- ( ( I e. W /\ i e. V ) -> i finSupp .0. )
143	141, 142	syl 17	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> i finSupp .0. )
144	17, 24	ring0cl 18569	. . . . . . . 8 |- ( R e. Ring -> .0. e. B )
145	78, 144	syl 17	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> .0. e. B )
146	17, 20, 24	ringrz 18588	. . . . . . . 8 |- ( ( R e. Ring /\ y e. B ) -> ( y .x. .0. ) = .0. )
147	78, 146	sylan 488	. . . . . . 7 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ y e. B ) -> ( y .x. .0. ) = .0. )
148	77, 145, 111, 90, 147	suppofss2d 7333	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) supp .0. ) C_ ( i supp .0. ) )
149		fsuppsssupp 8291	. . . . . 6 |- ( ( ( ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) e. _V /\ Fun ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) ) /\ ( i finSupp .0. /\ ( ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) supp .0. ) C_ ( i supp .0. ) ) ) -> ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) finSupp .0. )
150	132, 138, 143, 148, 149	syl22anc 1327	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( x e. I |-> ( k .x. ( g ` x ) ) ) oF .x. i ) finSupp .0. )
151	131, 150	eqbrtrrd 4677	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) finSupp .0. )
152		simp1 1061	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ph )
153		eleq1 2689	. . . . . . . . 9 |- ( g = h -> ( g e. V <-> h e. V ) )
154		id 22	. . . . . . . . . . 11 |- ( g = h -> g = h )
155	154, 154	oveq12d 6668	. . . . . . . . . 10 |- ( g = h -> ( g ., g ) = ( h ., h ) )
156	155	eqeq1d 2624	. . . . . . . . 9 |- ( g = h -> ( ( g ., g ) = .0. <-> ( h ., h ) = .0. ) )
157	153, 156	3anbi23d 1402	. . . . . . . 8 |- ( g = h -> ( ( ph /\ g e. V /\ ( g ., g ) = .0. ) <-> ( ph /\ h e. V /\ ( h ., h ) = .0. ) ) )
158		eqeq1 2626	. . . . . . . 8 |- ( g = h -> ( g = O <-> h = O ) )
159	157, 158	imbi12d 334	. . . . . . 7 |- ( g = h -> ( ( ( ph /\ g e. V /\ ( g ., g ) = .0. ) -> g = O ) <-> ( ( ph /\ h e. V /\ ( h ., h ) = .0. ) -> h = O ) ) )
160	159, 71	chvarv 2263	. . . . . 6 |- ( ( ph /\ h e. V /\ ( h ., h ) = .0. ) -> h = O )
161	14, 17, 20, 1, 5, 7, 24, 22, 9, 160, 35, 13	frlmphllem 20119	. . . . 5 |- ( ( ph /\ h e. V /\ i e. V ) -> ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) finSupp .0. )
162	152, 96, 86, 161	syl3anc 1326	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) finSupp .0. )
163	17, 24, 75, 76, 77, 95, 101, 102, 103, 151, 162	gsummptfsadd 18324	. . 3 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( R gsumg ( x e. I |-> ( ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) ) ) = ( ( R gsumg ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) ) ( +g ` R ) ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) ) ) )
164	14, 17, 20	frlmip 20117	. . . . . . . . 9 |- ( ( I e. W /\ R e. DivRing ) -> ( g e. ( B ^m I ) , h e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) ) = ( .i ` Y ) )
165	13, 12, 164	syl2anc 693	. . . . . . . 8 |- ( ph -> ( g e. ( B ^m I ) , h e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) ) = ( .i ` Y ) )
166	165, 5	syl6reqr 2675	. . . . . . 7 |- ( ph -> ., = ( g e. ( B ^m I ) , h e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) ) )
167		fveq1 6190	. . . . . . . . . . 11 |- ( e = g -> ( e ` x ) = ( g ` x ) )
168	167	oveq1d 6665	. . . . . . . . . 10 |- ( e = g -> ( ( e ` x ) .x. ( f ` x ) ) = ( ( g ` x ) .x. ( f ` x ) ) )
169	168	mpteq2dv 4745	. . . . . . . . 9 |- ( e = g -> ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) = ( x e. I |-> ( ( g ` x ) .x. ( f ` x ) ) ) )
170	169	oveq2d 6666	. . . . . . . 8 |- ( e = g -> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( f ` x ) ) ) ) )
171		fveq1 6190	. . . . . . . . . . 11 |- ( f = h -> ( f ` x ) = ( h ` x ) )
172	171	oveq2d 6666	. . . . . . . . . 10 |- ( f = h -> ( ( g ` x ) .x. ( f ` x ) ) = ( ( g ` x ) .x. ( h ` x ) ) )
173	172	mpteq2dv 4745	. . . . . . . . 9 |- ( f = h -> ( x e. I |-> ( ( g ` x ) .x. ( f ` x ) ) ) = ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) )
174	173	oveq2d 6666	. . . . . . . 8 |- ( f = h -> ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( f ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) )
175	170, 174	cbvmpt2v 6735	. . . . . . 7 |- ( e e. ( B ^m I ) , f e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) ) = ( g e. ( B ^m I ) , h e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) )
176	166, 175	syl6eqr 2674	. . . . . 6 |- ( ph -> ., = ( e e. ( B ^m I ) , f e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) ) )
177	176	3ad2ant1 1082	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ., = ( e e. ( B ^m I ) , f e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) ) )
178		simprl 794	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) /\ f = i ) ) -> e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) )
179	178	fveq1d 6193	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) /\ f = i ) ) -> ( e ` x ) = ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) )
180		simprr 796	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) /\ f = i ) ) -> f = i )
181	180	fveq1d 6193	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) /\ f = i ) ) -> ( f ` x ) = ( i ` x ) )
182	179, 181	oveq12d 6668	. . . . . . 7 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) /\ f = i ) ) -> ( ( e ` x ) .x. ( f ` x ) ) = ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) )
183	182	mpteq2dv 4745	. . . . . 6 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) /\ f = i ) ) -> ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) = ( x e. I |-> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) ) )
184	183	oveq2d 6666	. . . . 5 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) /\ f = i ) ) -> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) ) ) )
185	29	3ad2ant1 1082	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> Y e. LMod )
186	16	3ad2ant1 1082	. . . . . . . . . . 11 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> R = (Scalar ` Y ) )
187	186	fveq2d 6195	. . . . . . . . . 10 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( Base ` R ) = ( Base ` (Scalar ` Y ) ) )
188	17, 187	syl5eq 2668	. . . . . . . . 9 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> B = ( Base ` (Scalar ` Y ) ) )
189	80, 188	eleqtrd 2703	. . . . . . . 8 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> k e. ( Base ` (Scalar ` Y ) ) )
190		eqid 2622	. . . . . . . . 9 |- ( .s ` Y ) = ( .s ` Y )
191		eqid 2622	. . . . . . . . 9 |- ( Base ` (Scalar ` Y ) ) = ( Base ` (Scalar ` Y ) )
192	1, 31, 190, 191	lmodvscl 18880	. . . . . . . 8 |- ( ( Y e. LMod /\ k e. ( Base ` (Scalar ` Y ) ) /\ g e. V ) -> ( k ( .s ` Y ) g ) e. V )
193	185, 189, 82, 192	syl3anc 1326	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( k ( .s ` Y ) g ) e. V )
194		eqid 2622	. . . . . . . 8 |- ( +g ` Y ) = ( +g ` Y )
195	1, 194	lmodvacl 18877	. . . . . . 7 |- ( ( Y e. LMod /\ ( k ( .s ` Y ) g ) e. V /\ h e. V ) -> ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) e. V )
196	185, 193, 96, 195	syl3anc 1326	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) e. V )
197	14, 17, 1	frlmbasmap 20103	. . . . . 6 |- ( ( I e. W /\ ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) e. V ) -> ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) e. ( B ^m I ) )
198	77, 196, 197	syl2anc 693	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) e. ( B ^m I ) )
199		ovexd 6680	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( R gsumg ( x e. I |-> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) ) ) e. _V )
200	177, 184, 198, 88, 199	ovmpt2d 6788	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ., i ) = ( R gsumg ( x e. I |-> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) ) ) )
201	14, 1, 78, 77, 193, 96, 75, 194	frlmplusgval 20107	. . . . . . . . . 10 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) = ( ( k ( .s ` Y ) g ) oF ( +g ` R ) h ) )
202	14, 17, 1	frlmbasmap 20103	. . . . . . . . . . . . 13 |- ( ( I e. W /\ ( k ( .s ` Y ) g ) e. V ) -> ( k ( .s ` Y ) g ) e. ( B ^m I ) )
203	77, 193, 202	syl2anc 693	. . . . . . . . . . . 12 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( k ( .s ` Y ) g ) e. ( B ^m I ) )
204		elmapi 7879	. . . . . . . . . . . 12 |- ( ( k ( .s ` Y ) g ) e. ( B ^m I ) -> ( k ( .s ` Y ) g ) : I --> B )
205		ffn 6045	. . . . . . . . . . . 12 |- ( ( k ( .s ` Y ) g ) : I --> B -> ( k ( .s ` Y ) g ) Fn I )
206	203, 204, 205	3syl 18	. . . . . . . . . . 11 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( k ( .s ` Y ) g ) Fn I )
207	98, 53	syl 17	. . . . . . . . . . 11 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> h Fn I )
208	77	adantr 481	. . . . . . . . . . . 12 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> I e. W )
209	82	adantr 481	. . . . . . . . . . . 12 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> g e. V )
210	14, 1, 17, 208, 81, 209, 122, 190, 20	frlmvscaval 20110	. . . . . . . . . . 11 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( k ( .s ` Y ) g ) ` x ) = ( k .x. ( g ` x ) ) )
211		eqidd 2623	. . . . . . . . . . 11 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( h ` x ) = ( h ` x ) )
212	206, 207, 77, 77, 55, 210, 211	offval 6904	. . . . . . . . . 10 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( k ( .s ` Y ) g ) oF ( +g ` R ) h ) = ( x e. I |-> ( ( k .x. ( g ` x ) ) ( +g ` R ) ( h ` x ) ) ) )
213	201, 212	eqtrd 2656	. . . . . . . . 9 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) = ( x e. I |-> ( ( k .x. ( g ` x ) ) ( +g ` R ) ( h ` x ) ) ) )
214		ovexd 6680	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( k .x. ( g ` x ) ) ( +g ` R ) ( h ` x ) ) e. _V )
215	213, 214	fvmpt2d 6293	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) = ( ( k .x. ( g ` x ) ) ( +g ` R ) ( h ` x ) ) )
216	215	oveq1d 6665	. . . . . . 7 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) = ( ( ( k .x. ( g ` x ) ) ( +g ` R ) ( h ` x ) ) .x. ( i ` x ) ) )
217	17, 75, 20	ringdir 18567	. . . . . . . 8 |- ( ( R e. Ring /\ ( ( k .x. ( g ` x ) ) e. B /\ ( h ` x ) e. B /\ ( i ` x ) e. B ) ) -> ( ( ( k .x. ( g ` x ) ) ( +g ` R ) ( h ` x ) ) .x. ( i ` x ) ) = ( ( ( k .x. ( g ` x ) ) .x. ( i ` x ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) )
218	79, 109, 99, 91, 217	syl13anc 1328	. . . . . . 7 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( ( k .x. ( g ` x ) ) ( +g ` R ) ( h ` x ) ) .x. ( i ` x ) ) = ( ( ( k .x. ( g ` x ) ) .x. ( i ` x ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) )
219	128	oveq1d 6665	. . . . . . 7 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( ( k .x. ( g ` x ) ) .x. ( i ` x ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) = ( ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) )
220	216, 218, 219	3eqtrd 2660	. . . . . 6 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ x e. I ) -> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) = ( ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) )
221	220	mpteq2dva 4744	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) ) = ( x e. I |-> ( ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) ) )
222	221	oveq2d 6666	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( R gsumg ( x e. I |-> ( ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ` x ) .x. ( i ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) ) ) )
223	200, 222	eqtrd 2656	. . 3 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ., i ) = ( R gsumg ( x e. I |-> ( ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ( +g ` R ) ( ( h ` x ) .x. ( i ` x ) ) ) ) ) )
224		simprl 794	. . . . . . . . . . 11 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = g /\ f = i ) ) -> e = g )
225	224	fveq1d 6193	. . . . . . . . . 10 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = g /\ f = i ) ) -> ( e ` x ) = ( g ` x ) )
226		simprr 796	. . . . . . . . . . 11 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = g /\ f = i ) ) -> f = i )
227	226	fveq1d 6193	. . . . . . . . . 10 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = g /\ f = i ) ) -> ( f ` x ) = ( i ` x ) )
228	225, 227	oveq12d 6668	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = g /\ f = i ) ) -> ( ( e ` x ) .x. ( f ` x ) ) = ( ( g ` x ) .x. ( i ` x ) ) )
229	228	mpteq2dv 4745	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = g /\ f = i ) ) -> ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) = ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) )
230	229	oveq2d 6666	. . . . . . 7 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = g /\ f = i ) ) -> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) ) )
231		ovexd 6680	. . . . . . 7 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) ) e. _V )
232	177, 230, 83, 88, 231	ovmpt2d 6788	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( g ., i ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) ) )
233	232	oveq2d 6666	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( k .x. ( g ., i ) ) = ( k .x. ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) ) ) )
234	14, 17, 20, 1, 5, 7, 24, 22, 9, 71, 35, 13	frlmphllem 20119	. . . . . . 7 |- ( ( ph /\ g e. V /\ i e. V ) -> ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) finSupp .0. )
235	152, 82, 86, 234	syl3anc 1326	. . . . . 6 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) finSupp .0. )
236	17, 24, 75, 20, 78, 77, 80, 93, 235	gsummulc2 18607	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( R gsumg ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) ) = ( k .x. ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( i ` x ) ) ) ) ) )
237	233, 236	eqtr4d 2659	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( k .x. ( g ., i ) ) = ( R gsumg ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) ) )
238		simprl 794	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = h /\ f = i ) ) -> e = h )
239	238	fveq1d 6193	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = h /\ f = i ) ) -> ( e ` x ) = ( h ` x ) )
240		simprr 796	. . . . . . . . 9 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = h /\ f = i ) ) -> f = i )
241	240	fveq1d 6193	. . . . . . . 8 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = h /\ f = i ) ) -> ( f ` x ) = ( i ` x ) )
242	239, 241	oveq12d 6668	. . . . . . 7 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = h /\ f = i ) ) -> ( ( e ` x ) .x. ( f ` x ) ) = ( ( h ` x ) .x. ( i ` x ) ) )
243	242	mpteq2dv 4745	. . . . . 6 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = h /\ f = i ) ) -> ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) = ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) )
244	243	oveq2d 6666	. . . . 5 |- ( ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) /\ ( e = h /\ f = i ) ) -> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) ) )
245		ovexd 6680	. . . . 5 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) ) e. _V )
246	177, 244, 97, 88, 245	ovmpt2d 6788	. . . 4 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( h ., i ) = ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) ) )
247	237, 246	oveq12d 6668	. . 3 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( k .x. ( g ., i ) ) ( +g ` R ) ( h ., i ) ) = ( ( R gsumg ( x e. I |-> ( k .x. ( ( g ` x ) .x. ( i ` x ) ) ) ) ) ( +g ` R ) ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( i ` x ) ) ) ) ) )
248	163, 223, 247	3eqtr4d 2666	. 2 |- ( ( ph /\ k e. B /\ ( g e. V /\ h e. V /\ i e. V ) ) -> ( ( ( k ( .s ` Y ) g ) ( +g ` Y ) h ) ., i ) = ( ( k .x. ( g ., i ) ) ( +g ` R ) ( h ., i ) ) )
249	34	3ad2ant1 1082	. . . . . . 7 |- ( ( ph /\ g e. V /\ h e. V ) -> R e. CRing )
250	249	adantr 481	. . . . . 6 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> R e. CRing )
251	17, 20	crngcom 18562	. . . . . 6 |- ( ( R e. CRing /\ ( h ` x ) e. B /\ ( g ` x ) e. B ) -> ( ( h ` x ) .x. ( g ` x ) ) = ( ( g ` x ) .x. ( h ` x ) ) )
252	250, 66, 65, 251	syl3anc 1326	. . . . 5 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ x e. I ) -> ( ( h ` x ) .x. ( g ` x ) ) = ( ( g ` x ) .x. ( h ` x ) ) )
253	252	mpteq2dva 4744	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ( x e. I |-> ( ( h ` x ) .x. ( g ` x ) ) ) = ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) )
254	253	oveq2d 6666	. . 3 |- ( ( ph /\ g e. V /\ h e. V ) -> ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( g ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) )
255	176	3ad2ant1 1082	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ., = ( e e. ( B ^m I ) , f e. ( B ^m I ) |-> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) ) )
256		simprl 794	. . . . . . . 8 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ ( e = h /\ f = g ) ) -> e = h )
257	256	fveq1d 6193	. . . . . . 7 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ ( e = h /\ f = g ) ) -> ( e ` x ) = ( h ` x ) )
258		simprr 796	. . . . . . . 8 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ ( e = h /\ f = g ) ) -> f = g )
259	258	fveq1d 6193	. . . . . . 7 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ ( e = h /\ f = g ) ) -> ( f ` x ) = ( g ` x ) )
260	257, 259	oveq12d 6668	. . . . . 6 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ ( e = h /\ f = g ) ) -> ( ( e ` x ) .x. ( f ` x ) ) = ( ( h ` x ) .x. ( g ` x ) ) )
261	260	mpteq2dv 4745	. . . . 5 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ ( e = h /\ f = g ) ) -> ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) = ( x e. I |-> ( ( h ` x ) .x. ( g ` x ) ) ) )
262	261	oveq2d 6666	. . . 4 |- ( ( ( ph /\ g e. V /\ h e. V ) /\ ( e = h /\ f = g ) ) -> ( R gsumg ( x e. I |-> ( ( e ` x ) .x. ( f ` x ) ) ) ) = ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( g ` x ) ) ) ) )
263		ovexd 6680	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( g ` x ) ) ) ) e. _V )
264	255, 262, 50, 44, 263	ovmpt2d 6788	. . 3 |- ( ( ph /\ g e. V /\ h e. V ) -> ( h ., g ) = ( R gsumg ( x e. I |-> ( ( h ` x ) .x. ( g ` x ) ) ) ) )
265	35	ralrimiva 2966	. . . . . 6 |- ( ph -> A. x e. B ( .* ` x ) = x )
266	265	3ad2ant1 1082	. . . . 5 |- ( ( ph /\ g e. V /\ h e. V ) -> A. x e. B ( .* ` x ) = x )
267		fveq2 6191	. . . . . . 7 |- ( x = ( g ., h ) -> ( .* ` x ) = ( .* ` ( g ., h ) ) )
268		id 22	. . . . . . 7 |- ( x = ( g ., h ) -> x = ( g ., h ) )
269	267, 268	eqeq12d 2637	. . . . . 6 |- ( x = ( g ., h ) -> ( ( .* ` x ) = x <-> ( .* ` ( g ., h ) ) = ( g ., h ) ) )
270	269	rspcv 3305	. . . . 5 |- ( ( g ., h ) e. B -> ( A. x e. B ( .* ` x ) = x -> ( .* ` ( g ., h ) ) = ( g ., h ) ) )
271	74, 266, 270	sylc 65	. . . 4 |- ( ( ph /\ g e. V /\ h e. V ) -> ( .* ` ( g ., h ) ) = ( g ., h ) )
272	271, 60	eqtrd 2656	. . 3 |- ( ( ph /\ g e. V /\ h e. V ) -> ( .* ` ( g ., h ) ) = ( R gsumg ( x e. I |-> ( ( g ` x ) .x. ( h ` x ) ) ) ) )
273	254, 264, 272	3eqtr4rd 2667	. 2 |- ( ( ph /\ g e. V /\ h e. V ) -> ( .* ` ( g ., h ) ) = ( h ., g ) )
274	2, 3, 4, 6, 8, 16, 18, 19, 21, 23, 25, 33, 36, 74, 248, 71, 273	isphld 19999	1 |- ( ph -> Y e. PreHil )

Syntax hints:   -> wi 4   /\ wa 384   /\ w3a 1037   = wceq 1483   e. wcel 1990   A.wral 2912   _Vcvv 3200   C_ wss 3574   class class class wbr 4653   |-> cmpt 4729   Fun wfun 5882   Fn wfn 5883   -->wf 5884   ` cfv 5888  (class class class)co 6650   |-> cmpt2 6652   oFcof 6895   supp csupp 7295   ^m cmap 7857   finSupp cfsupp 8275   Basecbs 15857   +g cplusg 15941   .rcmulr 15942   *rcstv 15943  Scalarcsca 15944   .scvsca 15945   .icip 15946   0gc0g 16100   gsum_g cgsu 16101  CMndccmn 18193   Ringcrg 18547   CRingccrg 18548   DivRingcdr 18747  Fieldcfield 18748   LModclmod 18863   LVecclvec 19102   PreHilcphl 19969   freeLMod cfrlm 20090

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-rep 4771  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949  ax-cnex 9992  ax-resscn 9993  ax-1cn 9994  ax-icn 9995  ax-addcl 9996  ax-addrcl 9997  ax-mulcl 9998  ax-mulrcl 9999  ax-mulcom 10000  ax-addass 10001  ax-mulass 10002  ax-distr 10003  ax-i2m1 10004  ax-1ne0 10005  ax-1rid 10006  ax-rnegex 10007  ax-rrecex 10008  ax-cnre 10009  ax-pre-lttri 10010  ax-pre-lttrn 10011  ax-pre-ltadd 10012  ax-pre-mulgt0 10013

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3or 1038  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-nel 2898  df-ral 2917  df-rex 2918  df-reu 2919  df-rmo 2920  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-pss 3590  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-tp 4182  df-op 4184  df-uni 4437  df-int 4476  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-tr 4753  df-id 5024  df-eprel 5029  df-po 5035  df-so 5036  df-fr 5073  df-se 5074  df-we 5075  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-pred 5680  df-ord 5726  df-on 5727  df-lim 5728  df-suc 5729  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-isom 5897  df-riota 6611  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-of 6897  df-om 7066  df-1st 7168  df-2nd 7169  df-supp 7296  df-tpos 7352  df-wrecs 7407  df-recs 7468  df-rdg 7506  df-1o 7560  df-oadd 7564  df-er 7742  df-map 7859  df-ixp 7909  df-en 7956  df-dom 7957  df-sdom 7958  df-fin 7959  df-fsupp 8276  df-sup 8348  df-oi 8415  df-card 8765  df-pnf 10076  df-mnf 10077  df-xr 10078  df-ltxr 10079  df-le 10080  df-sub 10268  df-neg 10269  df-nn 11021  df-2 11079  df-3 11080  df-4 11081  df-5 11082  df-6 11083  df-7 11084  df-8 11085  df-9 11086  df-n0 11293  df-z 11378  df-dec 11494  df-uz 11688  df-fz 12327  df-fzo 12466  df-seq 12802  df-hash 13118  df-struct 15859  df-ndx 15860  df-slot 15861  df-base 15863  df-sets 15864  df-ress 15865  df-plusg 15954  df-mulr 15955  df-sca 15957  df-vsca 15958  df-ip 15959  df-tset 15960  df-ple 15961  df-ds 15964  df-hom 15966  df-cco 15967  df-0g 16102  df-gsum 16103  df-prds 16108  df-pws 16110  df-mgm 17242  df-sgrp 17284  df-mnd 17295  df-mhm 17335  df-submnd 17336  df-grp 17425  df-minusg 17426  df-sbg 17427  df-subg 17591  df-ghm 17658  df-cntz 17750  df-cmn 18195  df-abl 18196  df-mgp 18490  df-ur 18502  df-ring 18549  df-cring 18550  df-oppr 18623  df-rnghom 18715  df-drng 18749  df-field 18750  df-subrg 18778  df-staf 18845  df-srng 18846  df-lmod 18865  df-lss 18933  df-lmhm 19022  df-lvec 19103  df-sra 19172  df-rgmod 19173  df-phl 19971  df-dsmm 20076  df-frlm 20091

This theorem is referenced by:  rrxcph  23180