Theorem heiborlem3 33612

Description: Lemma for heibor 33620. Using countable choice ax-cc 9257, we have fixed in advance a collection of finite 2 ^ -u n nets ( F ` n ) for X (note that an r-net is a set of points in X whose r -balls cover X). The set G is the subset of these points whose corresponding balls have no finite subcover (i.e. in the set K). If the theorem was false, then X would be in K, and so some ball at each level would also be in K. But we can say more than this; given a ball ( y B n ) on level n, since level n + 1 covers the space and thus also ( y B n ), using heiborlem1 33610 there is a ball on the next level whose intersection with ( y B n ) also has no finite subcover. Now since the set G is a countable union of finite sets, it is countable (which needs ax-cc 9257 via iunctb 9396), and so we can apply ax-cc 9257 to G directly to get a function from G to itself, which points from each ball in K to a ball on the next level in K, and such that the intersection between these balls is also in K. (Contributed by Jeff Madsen, 18-Jan-2014.)

Hypotheses

Ref	Expression
heibor.1	|- J = ( MetOpen ` D )
heibor.3	|- K = { u | -. E. v e. ( ~P U i^i Fin ) u C_ U. v }
heibor.4	|- G = { <. y , n >. | ( n e. NN0 /\ y e. ( F ` n ) /\ ( y B n ) e. K ) }
heibor.5	|- B = ( z e. X , m e. NN0 |-> ( z ( ball ` D ) ( 1 / ( 2 ^ m ) ) ) )
heibor.6	|- ( ph -> D e. ( CMet ` X ) )
heibor.7	|- ( ph -> F : NN0 --> ( ~P X i^i Fin ) )
heibor.8	|- ( ph -> A. n e. NN0 X = U_ y e. ( F ` n ) ( y B n ) )

Assertion

Ref	Expression
heiborlem3	|- ( ph -> E. g A. x e. G ( ( g ` x ) G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) )

Distinct variable groups:   x, n, y, u, F   x, g, G   ph, g, x   g, m, n, u, v, y, z, D, x   B, g, n, u, v, y   g, J, m, n, u, v, x, y, z   U, g, n, u, v, x, y, z   g, X, m, n, u, v, x, y, z   g, K, n, x, y, z   x, B

Allowed substitution hints:   ph( y, z, v, u, m, n)   B( z, m)   U( m)   F( z, v, g, m)   G( y, z, v, u, m, n)   K( v, u, m)

Proof of Theorem heiborlem3

Dummy variable t is distinct from all other variables.

Step	Hyp	Ref	Expression
1		nn0ex 11298	. . . . . 6 |- NN0 e. _V
2		fvex 6201	. . . . . . 7 |- ( F ` t ) e. _V
3		snex 4908	. . . . . . 7 |- { t } e. _V
4	2, 3	xpex 6962	. . . . . 6 |- ( ( F ` t ) X. { t } ) e. _V
5	1, 4	iunex 7147	. . . . 5 |- U_ t e. NN0 ( ( F ` t ) X. { t } ) e. _V
6		heibor.4	. . . . . . . . 9 |- G = { <. y , n >. | ( n e. NN0 /\ y e. ( F ` n ) /\ ( y B n ) e. K ) }
7	6	relopabi 5245	. . . . . . . 8 |- Rel G
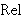8		1st2nd 7214	. . . . . . . 8 |- ( ( Rel G /\ x e. G ) -> x = <. ( 1st ` x ) , ( 2nd ` x ) >. )
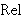9	7, 8	mpan 706	. . . . . . 7 |- ( x e. G -> x = <. ( 1st ` x ) , ( 2nd ` x ) >. )
10	9	eleq1d 2686	. . . . . . . . . . 11 |- ( x e. G -> ( x e. G <-> <. ( 1st ` x ) , ( 2nd ` x ) >. e. G ) )
11		df-br 4654	. . . . . . . . . . 11 |- ( ( 1st ` x ) G ( 2nd ` x ) <-> <. ( 1st ` x ) , ( 2nd ` x ) >. e. G )
12	10, 11	syl6bbr 278	. . . . . . . . . 10 |- ( x e. G -> ( x e. G <-> ( 1st ` x ) G ( 2nd ` x ) ) )
13		heibor.1	. . . . . . . . . . 11 |- J = ( MetOpen ` D )
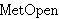14		heibor.3	. . . . . . . . . . 11 |- K = { u | -. E. v e. ( ~P U i^i Fin ) u C_ U. v }
15		fvex 6201	. . . . . . . . . . 11 |- ( 1st ` x ) e. _V
16		fvex 6201	. . . . . . . . . . 11 |- ( 2nd ` x ) e. _V
17	13, 14, 6, 15, 16	heiborlem2 33611	. . . . . . . . . 10 |- ( ( 1st ` x ) G ( 2nd ` x ) <-> ( ( 2nd ` x ) e. NN0 /\ ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) /\ ( ( 1st ` x ) B ( 2nd ` x ) ) e. K ) )
18	12, 17	syl6bb 276	. . . . . . . . 9 |- ( x e. G -> ( x e. G <-> ( ( 2nd ` x ) e. NN0 /\ ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) /\ ( ( 1st ` x ) B ( 2nd ` x ) ) e. K ) ) )
19	18	ibi 256	. . . . . . . 8 |- ( x e. G -> ( ( 2nd ` x ) e. NN0 /\ ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) /\ ( ( 1st ` x ) B ( 2nd ` x ) ) e. K ) )
20	16	snid 4208	. . . . . . . . . . . 12 |- ( 2nd ` x ) e. { ( 2nd ` x ) }
21		opelxp 5146	. . . . . . . . . . . 12 |- ( <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` ( 2nd ` x ) ) X. { ( 2nd ` x ) } ) <-> ( ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) /\ ( 2nd ` x ) e. { ( 2nd ` x ) } ) )
22	20, 21	mpbiran2 954	. . . . . . . . . . 11 |- ( <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` ( 2nd ` x ) ) X. { ( 2nd ` x ) } ) <-> ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) )
23		fveq2 6191	. . . . . . . . . . . . . 14 |- ( t = ( 2nd ` x ) -> ( F ` t ) = ( F ` ( 2nd ` x ) ) )
24		sneq 4187	. . . . . . . . . . . . . 14 |- ( t = ( 2nd ` x ) -> { t } = { ( 2nd ` x ) } )
25	23, 24	xpeq12d 5140	. . . . . . . . . . . . 13 |- ( t = ( 2nd ` x ) -> ( ( F ` t ) X. { t } ) = ( ( F ` ( 2nd ` x ) ) X. { ( 2nd ` x ) } ) )
26	25	eleq2d 2687	. . . . . . . . . . . 12 |- ( t = ( 2nd ` x ) -> ( <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` t ) X. { t } ) <-> <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` ( 2nd ` x ) ) X. { ( 2nd ` x ) } ) ) )
27	26	rspcev 3309	. . . . . . . . . . 11 |- ( ( ( 2nd ` x ) e. NN0 /\ <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` ( 2nd ` x ) ) X. { ( 2nd ` x ) } ) ) -> E. t e. NN0 <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` t ) X. { t } ) )
28	22, 27	sylan2br 493	. . . . . . . . . 10 |- ( ( ( 2nd ` x ) e. NN0 /\ ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) ) -> E. t e. NN0 <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` t ) X. { t } ) )
29		eliun 4524	. . . . . . . . . 10 |- ( <. ( 1st ` x ) , ( 2nd ` x ) >. e. U_ t e. NN0 ( ( F ` t ) X. { t } ) <-> E. t e. NN0 <. ( 1st ` x ) , ( 2nd ` x ) >. e. ( ( F ` t ) X. { t } ) )
30	28, 29	sylibr 224	. . . . . . . . 9 |- ( ( ( 2nd ` x ) e. NN0 /\ ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) ) -> <. ( 1st ` x ) , ( 2nd ` x ) >. e. U_ t e. NN0 ( ( F ` t ) X. { t } ) )
31	30	3adant3 1081	. . . . . . . 8 |- ( ( ( 2nd ` x ) e. NN0 /\ ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) /\ ( ( 1st ` x ) B ( 2nd ` x ) ) e. K ) -> <. ( 1st ` x ) , ( 2nd ` x ) >. e. U_ t e. NN0 ( ( F ` t ) X. { t } ) )
32	19, 31	syl 17	. . . . . . 7 |- ( x e. G -> <. ( 1st ` x ) , ( 2nd ` x ) >. e. U_ t e. NN0 ( ( F ` t ) X. { t } ) )
33	9, 32	eqeltrd 2701	. . . . . 6 |- ( x e. G -> x e. U_ t e. NN0 ( ( F ` t ) X. { t } ) )
34	33	ssriv 3607	. . . . 5 |- G C_ U_ t e. NN0 ( ( F ` t ) X. { t } )
35		ssdomg 8001	. . . . 5 |- ( U_ t e. NN0 ( ( F ` t ) X. { t } ) e. _V -> ( G C_ U_ t e. NN0 ( ( F ` t ) X. { t } ) -> G ~<_ U_ t e. NN0 ( ( F ` t ) X. { t } ) ) )
36	5, 34, 35	mp2 9	. . . 4 |- G ~<_ U_ t e. NN0 ( ( F ` t ) X. { t } )
37		nn0ennn 12778	. . . . . . 7 |- NN0 ~~ NN
38		nnenom 12779	. . . . . . 7 |- NN ~~ om
39	37, 38	entri 8010	. . . . . 6 |- NN0 ~~ om
40		endom 7982	. . . . . 6 |- ( NN0 ~~ om -> NN0 ~<_ om )
41	39, 40	ax-mp 5	. . . . 5 |- NN0 ~<_ om
42		vex 3203	. . . . . . . 8 |- t e. _V
43	2, 42	xpsnen 8044	. . . . . . 7 |- ( ( F ` t ) X. { t } ) ~~ ( F ` t )
44		inss2 3834	. . . . . . . . 9 |- ( ~P X i^i Fin ) C_ Fin
45		heibor.7	. . . . . . . . . 10 |- ( ph -> F : NN0 --> ( ~P X i^i Fin ) )
46	45	ffvelrnda 6359	. . . . . . . . 9 |- ( ( ph /\ t e. NN0 ) -> ( F ` t ) e. ( ~P X i^i Fin ) )
47	44, 46	sseldi 3601	. . . . . . . 8 |- ( ( ph /\ t e. NN0 ) -> ( F ` t ) e. Fin )
48		isfinite 8549	. . . . . . . . 9 |- ( ( F ` t ) e. Fin <-> ( F ` t ) ~< om )
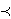49		sdomdom 7983	. . . . . . . . 9 |- ( ( F ` t ) ~< om -> ( F ` t ) ~<_ om )
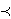
50	48, 49	sylbi 207	. . . . . . . 8 |- ( ( F ` t ) e. Fin -> ( F ` t ) ~<_ om )
51	47, 50	syl 17	. . . . . . 7 |- ( ( ph /\ t e. NN0 ) -> ( F ` t ) ~<_ om )
52		endomtr 8014	. . . . . . 7 |- ( ( ( ( F ` t ) X. { t } ) ~~ ( F ` t ) /\ ( F ` t ) ~<_ om ) -> ( ( F ` t ) X. { t } ) ~<_ om )
53	43, 51, 52	sylancr 695	. . . . . 6 |- ( ( ph /\ t e. NN0 ) -> ( ( F ` t ) X. { t } ) ~<_ om )
54	53	ralrimiva 2966	. . . . 5 |- ( ph -> A. t e. NN0 ( ( F ` t ) X. { t } ) ~<_ om )
55		iunctb 9396	. . . . 5 |- ( ( NN0 ~<_ om /\ A. t e. NN0 ( ( F ` t ) X. { t } ) ~<_ om ) -> U_ t e. NN0 ( ( F ` t ) X. { t } ) ~<_ om )
56	41, 54, 55	sylancr 695	. . . 4 |- ( ph -> U_ t e. NN0 ( ( F ` t ) X. { t } ) ~<_ om )
57		domtr 8009	. . . 4 |- ( ( G ~<_ U_ t e. NN0 ( ( F ` t ) X. { t } ) /\ U_ t e. NN0 ( ( F ` t ) X. { t } ) ~<_ om ) -> G ~<_ om )
58	36, 56, 57	sylancr 695	. . 3 |- ( ph -> G ~<_ om )
59	19	simp1d 1073	. . . . . . . . 9 |- ( x e. G -> ( 2nd ` x ) e. NN0 )
60		peano2nn0 11333	. . . . . . . . 9 |- ( ( 2nd ` x ) e. NN0 -> ( ( 2nd ` x ) + 1 ) e. NN0 )
61	59, 60	syl 17	. . . . . . . 8 |- ( x e. G -> ( ( 2nd ` x ) + 1 ) e. NN0 )
62		ffvelrn 6357	. . . . . . . 8 |- ( ( F : NN0 --> ( ~P X i^i Fin ) /\ ( ( 2nd ` x ) + 1 ) e. NN0 ) -> ( F ` ( ( 2nd ` x ) + 1 ) ) e. ( ~P X i^i Fin ) )
63	45, 61, 62	syl2an 494	. . . . . . 7 |- ( ( ph /\ x e. G ) -> ( F ` ( ( 2nd ` x ) + 1 ) ) e. ( ~P X i^i Fin ) )
64	44, 63	sseldi 3601	. . . . . 6 |- ( ( ph /\ x e. G ) -> ( F ` ( ( 2nd ` x ) + 1 ) ) e. Fin )
65		iunin2 4584	. . . . . . . 8 |- U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) = ( ( B ` x ) i^i U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) )
66		heibor.8	. . . . . . . . . . 11 |- ( ph -> A. n e. NN0 X = U_ y e. ( F ` n ) ( y B n ) )
67		oveq1 6657	. . . . . . . . . . . . . . . 16 |- ( y = t -> ( y B n ) = ( t B n ) )
68	67	cbviunv 4559	. . . . . . . . . . . . . . 15 |- U_ y e. ( F ` n ) ( y B n ) = U_ t e. ( F ` n ) ( t B n )
69		fveq2 6191	. . . . . . . . . . . . . . . 16 |- ( n = ( ( 2nd ` x ) + 1 ) -> ( F ` n ) = ( F ` ( ( 2nd ` x ) + 1 ) ) )
70	69	iuneq1d 4545	. . . . . . . . . . . . . . 15 |- ( n = ( ( 2nd ` x ) + 1 ) -> U_ t e. ( F ` n ) ( t B n ) = U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B n ) )
71	68, 70	syl5eq 2668	. . . . . . . . . . . . . 14 |- ( n = ( ( 2nd ` x ) + 1 ) -> U_ y e. ( F ` n ) ( y B n ) = U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B n ) )
72		oveq2 6658	. . . . . . . . . . . . . . 15 |- ( n = ( ( 2nd ` x ) + 1 ) -> ( t B n ) = ( t B ( ( 2nd ` x ) + 1 ) ) )
73	72	iuneq2d 4547	. . . . . . . . . . . . . 14 |- ( n = ( ( 2nd ` x ) + 1 ) -> U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B n ) = U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) )
74	71, 73	eqtrd 2656	. . . . . . . . . . . . 13 |- ( n = ( ( 2nd ` x ) + 1 ) -> U_ y e. ( F ` n ) ( y B n ) = U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) )
75	74	eqeq2d 2632	. . . . . . . . . . . 12 |- ( n = ( ( 2nd ` x ) + 1 ) -> ( X = U_ y e. ( F ` n ) ( y B n ) <-> X = U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) ) )
76	75	rspccva 3308	. . . . . . . . . . 11 |- ( ( A. n e. NN0 X = U_ y e. ( F ` n ) ( y B n ) /\ ( ( 2nd ` x ) + 1 ) e. NN0 ) -> X = U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) )
77	66, 61, 76	syl2an 494	. . . . . . . . . 10 |- ( ( ph /\ x e. G ) -> X = U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) )
78	77	ineq2d 3814	. . . . . . . . 9 |- ( ( ph /\ x e. G ) -> ( ( B ` x ) i^i X ) = ( ( B ` x ) i^i U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) ) )
79	9	fveq2d 6195	. . . . . . . . . . . . . 14 |- ( x e. G -> ( B ` x ) = ( B ` <. ( 1st ` x ) , ( 2nd ` x ) >. ) )
80		df-ov 6653	. . . . . . . . . . . . . 14 |- ( ( 1st ` x ) B ( 2nd ` x ) ) = ( B ` <. ( 1st ` x ) , ( 2nd ` x ) >. )
81	79, 80	syl6eqr 2674	. . . . . . . . . . . . 13 |- ( x e. G -> ( B ` x ) = ( ( 1st ` x ) B ( 2nd ` x ) ) )
82	81	adantl 482	. . . . . . . . . . . 12 |- ( ( ph /\ x e. G ) -> ( B ` x ) = ( ( 1st ` x ) B ( 2nd ` x ) ) )
83		inss1 3833	. . . . . . . . . . . . . . . 16 |- ( ~P X i^i Fin ) C_ ~P X
84		ffvelrn 6357	. . . . . . . . . . . . . . . . 17 |- ( ( F : NN0 --> ( ~P X i^i Fin ) /\ ( 2nd ` x ) e. NN0 ) -> ( F ` ( 2nd ` x ) ) e. ( ~P X i^i Fin ) )
85	45, 59, 84	syl2an 494	. . . . . . . . . . . . . . . 16 |- ( ( ph /\ x e. G ) -> ( F ` ( 2nd ` x ) ) e. ( ~P X i^i Fin ) )
86	83, 85	sseldi 3601	. . . . . . . . . . . . . . 15 |- ( ( ph /\ x e. G ) -> ( F ` ( 2nd ` x ) ) e. ~P X )
87	86	elpwid 4170	. . . . . . . . . . . . . 14 |- ( ( ph /\ x e. G ) -> ( F ` ( 2nd ` x ) ) C_ X )
88	19	simp2d 1074	. . . . . . . . . . . . . . 15 |- ( x e. G -> ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) )
89	88	adantl 482	. . . . . . . . . . . . . 14 |- ( ( ph /\ x e. G ) -> ( 1st ` x ) e. ( F ` ( 2nd ` x ) ) )
90	87, 89	sseldd 3604	. . . . . . . . . . . . 13 |- ( ( ph /\ x e. G ) -> ( 1st ` x ) e. X )
91	59	adantl 482	. . . . . . . . . . . . 13 |- ( ( ph /\ x e. G ) -> ( 2nd ` x ) e. NN0 )
92		oveq1 6657	. . . . . . . . . . . . . 14 |- ( z = ( 1st ` x ) -> ( z ( ball ` D ) ( 1 / ( 2 ^ m ) ) ) = ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ m ) ) ) )
93		oveq2 6658	. . . . . . . . . . . . . . . 16 |- ( m = ( 2nd ` x ) -> ( 2 ^ m ) = ( 2 ^ ( 2nd ` x ) ) )
94	93	oveq2d 6666	. . . . . . . . . . . . . . 15 |- ( m = ( 2nd ` x ) -> ( 1 / ( 2 ^ m ) ) = ( 1 / ( 2 ^ ( 2nd ` x ) ) ) )
95	94	oveq2d 6666	. . . . . . . . . . . . . 14 |- ( m = ( 2nd ` x ) -> ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ m ) ) ) = ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ ( 2nd ` x ) ) ) ) )
96		heibor.5	. . . . . . . . . . . . . 14 |- B = ( z e. X , m e. NN0 |-> ( z ( ball ` D ) ( 1 / ( 2 ^ m ) ) ) )
97		ovex 6678	. . . . . . . . . . . . . 14 |- ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ ( 2nd ` x ) ) ) ) e. _V
98	92, 95, 96, 97	ovmpt2 6796	. . . . . . . . . . . . 13 |- ( ( ( 1st ` x ) e. X /\ ( 2nd ` x ) e. NN0 ) -> ( ( 1st ` x ) B ( 2nd ` x ) ) = ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ ( 2nd ` x ) ) ) ) )
99	90, 91, 98	syl2anc 693	. . . . . . . . . . . 12 |- ( ( ph /\ x e. G ) -> ( ( 1st ` x ) B ( 2nd ` x ) ) = ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ ( 2nd ` x ) ) ) ) )
100	82, 99	eqtrd 2656	. . . . . . . . . . 11 |- ( ( ph /\ x e. G ) -> ( B ` x ) = ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ ( 2nd ` x ) ) ) ) )
101		heibor.6	. . . . . . . . . . . . . . 15 |- ( ph -> D e. ( CMet ` X ) )
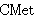102		cmetmet 23084	. . . . . . . . . . . . . . 15 |- ( D e. ( CMet ` X ) -> D e. ( Met ` X ) )
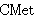
103	101, 102	syl 17	. . . . . . . . . . . . . 14 |- ( ph -> D e. ( Met ` X ) )
104		metxmet 22139	. . . . . . . . . . . . . 14 |- ( D e. ( Met ` X ) -> D e. ( *Met ` X ) )
105	103, 104	syl 17	. . . . . . . . . . . . 13 |- ( ph -> D e. ( *Met ` X ) )
106	105	adantr 481	. . . . . . . . . . . 12 |- ( ( ph /\ x e. G ) -> D e. ( *Met ` X ) )
107		2nn 11185	. . . . . . . . . . . . . . . 16 |- 2 e. NN
108		nnexpcl 12873	. . . . . . . . . . . . . . . 16 |- ( ( 2 e. NN /\ ( 2nd ` x ) e. NN0 ) -> ( 2 ^ ( 2nd ` x ) ) e. NN )
109	107, 91, 108	sylancr 695	. . . . . . . . . . . . . . 15 |- ( ( ph /\ x e. G ) -> ( 2 ^ ( 2nd ` x ) ) e. NN )
110	109	nnrpd 11870	. . . . . . . . . . . . . 14 |- ( ( ph /\ x e. G ) -> ( 2 ^ ( 2nd ` x ) ) e. RR+ )
111	110	rpreccld 11882	. . . . . . . . . . . . 13 |- ( ( ph /\ x e. G ) -> ( 1 / ( 2 ^ ( 2nd ` x ) ) ) e. RR+ )
112	111	rpxrd 11873	. . . . . . . . . . . 12 |- ( ( ph /\ x e. G ) -> ( 1 / ( 2 ^ ( 2nd ` x ) ) ) e. RR* )
113		blssm 22223	. . . . . . . . . . . 12 |- ( ( D e. ( *Met ` X ) /\ ( 1st ` x ) e. X /\ ( 1 / ( 2 ^ ( 2nd ` x ) ) ) e. RR* ) -> ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ ( 2nd ` x ) ) ) ) C_ X )
114	106, 90, 112, 113	syl3anc 1326	. . . . . . . . . . 11 |- ( ( ph /\ x e. G ) -> ( ( 1st ` x ) ( ball ` D ) ( 1 / ( 2 ^ ( 2nd ` x ) ) ) ) C_ X )
115	100, 114	eqsstrd 3639	. . . . . . . . . 10 |- ( ( ph /\ x e. G ) -> ( B ` x ) C_ X )
116		df-ss 3588	. . . . . . . . . 10 |- ( ( B ` x ) C_ X <-> ( ( B ` x ) i^i X ) = ( B ` x ) )
117	115, 116	sylib 208	. . . . . . . . 9 |- ( ( ph /\ x e. G ) -> ( ( B ` x ) i^i X ) = ( B ` x ) )
118	78, 117	eqtr3d 2658	. . . . . . . 8 |- ( ( ph /\ x e. G ) -> ( ( B ` x ) i^i U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( t B ( ( 2nd ` x ) + 1 ) ) ) = ( B ` x ) )
119	65, 118	syl5eq 2668	. . . . . . 7 |- ( ( ph /\ x e. G ) -> U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) = ( B ` x ) )
120		eqimss2 3658	. . . . . . 7 |- ( U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) = ( B ` x ) -> ( B ` x ) C_ U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) )
121	119, 120	syl 17	. . . . . 6 |- ( ( ph /\ x e. G ) -> ( B ` x ) C_ U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) )
122	19	simp3d 1075	. . . . . . . 8 |- ( x e. G -> ( ( 1st ` x ) B ( 2nd ` x ) ) e. K )
123	81, 122	eqeltrd 2701	. . . . . . 7 |- ( x e. G -> ( B ` x ) e. K )
124	123	adantl 482	. . . . . 6 |- ( ( ph /\ x e. G ) -> ( B ` x ) e. K )
125		fvex 6201	. . . . . . . 8 |- ( B ` x ) e. _V
126	125	inex1 4799	. . . . . . 7 |- ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. _V
127	13, 14, 126	heiborlem1 33610	. . . . . 6 |- ( ( ( F ` ( ( 2nd ` x ) + 1 ) ) e. Fin /\ ( B ` x ) C_ U_ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) /\ ( B ` x ) e. K ) -> E. t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K )
128	64, 121, 124, 127	syl3anc 1326	. . . . 5 |- ( ( ph /\ x e. G ) -> E. t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K )
129	83, 63	sseldi 3601	. . . . . . . . . . . 12 |- ( ( ph /\ x e. G ) -> ( F ` ( ( 2nd ` x ) + 1 ) ) e. ~P X )
130	129	elpwid 4170	. . . . . . . . . . 11 |- ( ( ph /\ x e. G ) -> ( F ` ( ( 2nd ` x ) + 1 ) ) C_ X )
131	13	mopnuni 22246	. . . . . . . . . . . . 13 |- ( D e. ( *Met ` X ) -> X = U. J )
132	105, 131	syl 17	. . . . . . . . . . . 12 |- ( ph -> X = U. J )
133	132	adantr 481	. . . . . . . . . . 11 |- ( ( ph /\ x e. G ) -> X = U. J )
134	130, 133	sseqtrd 3641	. . . . . . . . . 10 |- ( ( ph /\ x e. G ) -> ( F ` ( ( 2nd ` x ) + 1 ) ) C_ U. J )
135	134	sselda 3603	. . . . . . . . 9 |- ( ( ( ph /\ x e. G ) /\ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ) -> t e. U. J )
136	135	adantrr 753	. . . . . . . 8 |- ( ( ( ph /\ x e. G ) /\ ( t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) -> t e. U. J )
137	61	adantl 482	. . . . . . . . . 10 |- ( ( ph /\ x e. G ) -> ( ( 2nd ` x ) + 1 ) e. NN0 )
138		id 22	. . . . . . . . . 10 |- ( t e. ( F ` ( ( 2nd ` x ) + 1 ) ) -> t e. ( F ` ( ( 2nd ` x ) + 1 ) ) )
139		snfi 8038	. . . . . . . . . . . 12 |- { ( t B ( ( 2nd ` x ) + 1 ) ) } e. Fin
140		inss2 3834	. . . . . . . . . . . . 13 |- ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) C_ ( t B ( ( 2nd ` x ) + 1 ) )
141		ovex 6678	. . . . . . . . . . . . . . 15 |- ( t B ( ( 2nd ` x ) + 1 ) ) e. _V
142	141	unisn 4451	. . . . . . . . . . . . . 14 |- U. { ( t B ( ( 2nd ` x ) + 1 ) ) } = ( t B ( ( 2nd ` x ) + 1 ) )
143		uniiun 4573	. . . . . . . . . . . . . 14 |- U. { ( t B ( ( 2nd ` x ) + 1 ) ) } = U_ g e. { ( t B ( ( 2nd ` x ) + 1 ) ) } g
144	142, 143	eqtr3i 2646	. . . . . . . . . . . . 13 |- ( t B ( ( 2nd ` x ) + 1 ) ) = U_ g e. { ( t B ( ( 2nd ` x ) + 1 ) ) } g
145	140, 144	sseqtri 3637	. . . . . . . . . . . 12 |- ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) C_ U_ g e. { ( t B ( ( 2nd ` x ) + 1 ) ) } g
146		vex 3203	. . . . . . . . . . . . 13 |- g e. _V
147	13, 14, 146	heiborlem1 33610	. . . . . . . . . . . 12 |- ( ( { ( t B ( ( 2nd ` x ) + 1 ) ) } e. Fin /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) C_ U_ g e. { ( t B ( ( 2nd ` x ) + 1 ) ) } g /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) -> E. g e. { ( t B ( ( 2nd ` x ) + 1 ) ) } g e. K )
148	139, 145, 147	mp3an12 1414	. . . . . . . . . . 11 |- ( ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K -> E. g e. { ( t B ( ( 2nd ` x ) + 1 ) ) } g e. K )
149		eleq1 2689	. . . . . . . . . . . 12 |- ( g = ( t B ( ( 2nd ` x ) + 1 ) ) -> ( g e. K <-> ( t B ( ( 2nd ` x ) + 1 ) ) e. K ) )
150	141, 149	rexsn 4223	. . . . . . . . . . 11 |- ( E. g e. { ( t B ( ( 2nd ` x ) + 1 ) ) } g e. K <-> ( t B ( ( 2nd ` x ) + 1 ) ) e. K )
151	148, 150	sylib 208	. . . . . . . . . 10 |- ( ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K -> ( t B ( ( 2nd ` x ) + 1 ) ) e. K )
152		ovex 6678	. . . . . . . . . . . 12 |- ( ( 2nd ` x ) + 1 ) e. _V
153	13, 14, 6, 42, 152	heiborlem2 33611	. . . . . . . . . . 11 |- ( t G ( ( 2nd ` x ) + 1 ) <-> ( ( ( 2nd ` x ) + 1 ) e. NN0 /\ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( t B ( ( 2nd ` x ) + 1 ) ) e. K ) )
154	153	biimpri 218	. . . . . . . . . 10 |- ( ( ( ( 2nd ` x ) + 1 ) e. NN0 /\ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( t B ( ( 2nd ` x ) + 1 ) ) e. K ) -> t G ( ( 2nd ` x ) + 1 ) )
155	137, 138, 151, 154	syl3an 1368	. . . . . . . . 9 |- ( ( ( ph /\ x e. G ) /\ t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) -> t G ( ( 2nd ` x ) + 1 ) )
156	155	3expb 1266	. . . . . . . 8 |- ( ( ( ph /\ x e. G ) /\ ( t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) -> t G ( ( 2nd ` x ) + 1 ) )
157		simprr 796	. . . . . . . 8 |- ( ( ( ph /\ x e. G ) /\ ( t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) -> ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K )
158	136, 156, 157	jca32 558	. . . . . . 7 |- ( ( ( ph /\ x e. G ) /\ ( t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) -> ( t e. U. J /\ ( t G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) )
159	158	ex 450	. . . . . 6 |- ( ( ph /\ x e. G ) -> ( ( t e. ( F ` ( ( 2nd ` x ) + 1 ) ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) -> ( t e. U. J /\ ( t G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) ) )
160	159	reximdv2 3014	. . . . 5 |- ( ( ph /\ x e. G ) -> ( E. t e. ( F ` ( ( 2nd ` x ) + 1 ) ) ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K -> E. t e. U. J ( t G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) )
161	128, 160	mpd 15	. . . 4 |- ( ( ph /\ x e. G ) -> E. t e. U. J ( t G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) )
162	161	ralrimiva 2966	. . 3 |- ( ph -> A. x e. G E. t e. U. J ( t G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) )
163		fvex 6201	. . . . . 6 |- ( MetOpen ` D ) e. _V
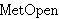164	13, 163	eqeltri 2697	. . . . 5 |- J e. _V
165	164	uniex 6953	. . . 4 |- U. J e. _V
166		breq1 4656	. . . . 5 |- ( t = ( g ` x ) -> ( t G ( ( 2nd ` x ) + 1 ) <-> ( g ` x ) G ( ( 2nd ` x ) + 1 ) ) )
167		oveq1 6657	. . . . . . 7 |- ( t = ( g ` x ) -> ( t B ( ( 2nd ` x ) + 1 ) ) = ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) )
168	167	ineq2d 3814	. . . . . 6 |- ( t = ( g ` x ) -> ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) = ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) )
169	168	eleq1d 2686	. . . . 5 |- ( t = ( g ` x ) -> ( ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K <-> ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) )
170	166, 169	anbi12d 747	. . . 4 |- ( t = ( g ` x ) -> ( ( t G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) <-> ( ( g ` x ) G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) )
171	165, 170	axcc4dom 9263	. . 3 |- ( ( G ~<_ om /\ A. x e. G E. t e. U. J ( t G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( t B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) -> E. g ( g : G --> U. J /\ A. x e. G ( ( g ` x ) G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) )
172	58, 162, 171	syl2anc 693	. 2 |- ( ph -> E. g ( g : G --> U. J /\ A. x e. G ( ( g ` x ) G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) )
173		exsimpr 1796	. 2 |- ( E. g ( g : G --> U. J /\ A. x e. G ( ( g ` x ) G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) ) -> E. g A. x e. G ( ( g ` x ) G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) )
174	172, 173	syl 17	1 |- ( ph -> E. g A. x e. G ( ( g ` x ) G ( ( 2nd ` x ) + 1 ) /\ ( ( B ` x ) i^i ( ( g ` x ) B ( ( 2nd ` x ) + 1 ) ) ) e. K ) )

Syntax hints:   -. wn 3   -> wi 4   /\ wa 384   /\ w3a 1037   = wceq 1483   E.wex 1704   e. wcel 1990   {cab 2608   A.wral 2912   E.wrex 2913   _Vcvv 3200   i^i cin 3573   C_ wss 3574   ~Pcpw 4158   {csn 4177   <.cop 4183   U.cuni 4436   U_ciun 4520   class class class wbr 4653   {copab 4712   X. cxp 5112   Rel wrel 5119   -->wf 5884   ` cfv 5888  (class class class)co 6650   |-> cmpt2 6652   omcom 7065   1stc1st 7166   2ndc2nd 7167   ~~ cen 7952   ~<_ cdom 7953   ~< csdm 7954   Fincfn 7955   1c1 9937   + caddc 9939   RR*cxr 10073   / cdiv 10684   NNcn 11020   2c2 11070   NN0cn0 11292   ^cexp 12860   *Metcxmt 19731   Metcme 19732   ballcbl 19733   MetOpencmopn 19736   CMetcms 23052

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-rep 4771  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949  ax-inf2 8538  ax-cc 9257  ax-cnex 9992  ax-resscn 9993  ax-1cn 9994  ax-icn 9995  ax-addcl 9996  ax-addrcl 9997  ax-mulcl 9998  ax-mulrcl 9999  ax-mulcom 10000  ax-addass 10001  ax-mulass 10002  ax-distr 10003  ax-i2m1 10004  ax-1ne0 10005  ax-1rid 10006  ax-rnegex 10007  ax-rrecex 10008  ax-cnre 10009  ax-pre-lttri 10010  ax-pre-lttrn 10011  ax-pre-ltadd 10012  ax-pre-mulgt0 10013  ax-pre-sup 10014

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3or 1038  df-3an 1039  df-tru 1486  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-nel 2898  df-ral 2917  df-rex 2918  df-reu 2919  df-rmo 2920  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-pss 3590  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-tp 4182  df-op 4184  df-uni 4437  df-int 4476  df-iun 4522  df-br 4654  df-opab 4713  df-mpt 4730  df-tr 4753  df-id 5024  df-eprel 5029  df-po 5035  df-so 5036  df-fr 5073  df-se 5074  df-we 5075  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-pred 5680  df-ord 5726  df-on 5727  df-lim 5728  df-suc 5729  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-isom 5897  df-riota 6611  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-om 7066  df-1st 7168  df-2nd 7169  df-wrecs 7407  df-recs 7468  df-rdg 7506  df-1o 7560  df-oadd 7564  df-er 7742  df-map 7859  df-en 7956  df-dom 7957  df-sdom 7958  df-fin 7959  df-sup 8348  df-inf 8349  df-oi 8415  df-card 8765  df-acn 8768  df-pnf 10076  df-mnf 10077  df-xr 10078  df-ltxr 10079  df-le 10080  df-sub 10268  df-neg 10269  df-div 10685  df-nn 11021  df-2 11079  df-n0 11293  df-z 11378  df-uz 11688  df-q 11789  df-rp 11833  df-xneg 11946  df-xadd 11947  df-xmul 11948  df-seq 12802  df-exp 12861  df-topgen 16104  df-psmet 19738  df-xmet 19739  df-met 19740  df-bl 19741  df-mopn 19742  df-top 20699  df-topon 20716  df-bases 20750  df-cmet 23055

This theorem is referenced by:  heiborlem10  33619