| Step | Hyp | Ref
| Expression |
|---|
| 1 | | eqid 2622 |
. . . . . . 7
   Mat Mat      Mat Mat   |
| 2 | | eqid 2622 |
. . . . . . 7
      Mat Mat          Mat Mat    |
| 3 | 1, 2 | matrcl 20218 |
. . . . . 6
         Mat Mat         
     |
| 4 | 3 | simpld 475 |
. . . . 5
         Mat Mat         |
| 5 | 4 | ad3antlr 767 |
. . . 4
       Field Field  
      Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod      
  |
| 6 | | isfld 18756 |
. . . . . . 7
    Field Field        
   |
| 7 | 6 | simplbi 476 |
. . . . . 6
    Field Field      |
| 8 | 7 | anim1i 592 |
. . . . 5
     Field Field
        Mat Mat     
    
       Mat Mat      |
| 9 | 4 | ad2antrl 764 |
. . . . . . . . . . . 12
         
     Mat Mat               |
| 10 | | simpr 477 |
. . . . . . . . . . . . . . 15
              Mat Mat     
       Mat Mat     |
| 11 | | xpfi 8231 |
. . . . . . . . . . . . . . . . . . . . 21
        
           |
| 12 | 11 | anidms 677 |
. . . . . . . . . . . . . . . . . . . 20
              |
| 13 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . 21
   freeLMod freeLMod  
       freeLMod freeLMod       |
| 14 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . 21
            |
| 15 | 13, 14 | frlmfibas 20105 |
. . . . . . . . . . . . . . . . . . . 20
                      
            freeLMod freeLMod         |
| 16 | 12, 15 | sylan2 491 |
. . . . . . . . . . . . . . . . . . 19
                               freeLMod freeLMod         |
| 17 | 1, 13 | matbas 20219 |
. . . . . . . . . . . . . . . . . . . 20
        
        freeLMod freeLMod              Mat Mat     |
| 18 | 17 | ancoms 469 |
. . . . . . . . . . . . . . . . . . 19
                 freeLMod freeLMod              Mat Mat     |
| 19 | 16, 18 | eqtrd 2656 |
. . . . . . . . . . . . . . . . . 18
                               Mat Mat     |
| 20 | 19 | eleq2d 2687 |
. . . . . . . . . . . . . . . . 17
              
        
    
       Mat Mat      |
| 21 | 4, 20 | sylan2 491 |
. . . . . . . . . . . . . . . 16
              Mat Mat     
         
      
       Mat Mat      |
| 22 | | fvex 6201 |
. . . . . . . . . . . . . . . . . 18
        |
| 23 | 4, 4, 11 | syl2anc 693 |
. . . . . . . . . . . . . . . . . 18
         Mat Mat             |
| 24 | | elmapg 7870 |
. . . . . . . . . . . . . . . . . 18
            
                
      
               |
| 25 | 22, 23, 24 | sylancr 695 |
. . . . . . . . . . . . . . . . 17
         Mat Mat              
      
               |
| 26 | 25 | adantl 482 |
. . . . . . . . . . . . . . . 16
              Mat Mat     
         
      
               |
| 27 | 21, 26 | bitr3d 270 |
. . . . . . . . . . . . . . 15
              Mat Mat     
        Mat Mat                    |
| 28 | 10, 27 | mpbid 222 |
. . . . . . . . . . . . . 14
              Mat Mat     
              |
| 29 | 28 | adantrr 753 |
. . . . . . . . . . . . 13
         
     Mat Mat                         |
| 30 | | eldifsn 4317 |
. . . . . . . . . . . . . . . 16
           
          |
| 31 | 30 | biimpri 218 |
. . . . . . . . . . . . . . 15
                      |
| 32 | 4, 31 | sylan 488 |
. . . . . . . . . . . . . 14
          Mat Mat        
           |
| 33 | 32 | adantl 482 |
. . . . . . . . . . . . 13
         
     Mat Mat                     |
| 34 | | curf 33387 |
. . . . . . . . . . . . . 14
                 
                  curry curry               |
| 35 | 22, 34 | mp3an3 1413 |
. . . . . . . . . . . . 13
                 
          curry
curry           
   |
| 36 | 29, 33, 35 | syl2anc 693 |
. . . . . . . . . . . 12
         
     Mat Mat           curry curry               |
| 37 | 9, 36 | jca 554 |
. . . . . . . . . . 11
         
     Mat Mat                curry curry                |
| 38 | 37 | ex 450 |
. . . . . . . . . 10
               Mat Mat               curry curry                 |
| 39 | 38 | imdistani 726 |
. . . . . . . . 9
         
     Mat Mat             
       curry curry                 |
| 40 | 39 | anassrs 680 |
. . . . . . . 8
         
     Mat Mat             
       curry curry                 |
| 41 | | anass 681 |
. . . . . . . 8
         
   curry curry               
    
     curry
curry           
     |
| 42 | 40, 41 | sylibr 224 |
. . . . . . 7
         
     Mat Mat                  
   curry curry                |
| 43 | | drngring 18754 |
. . . . . . . . . . . . 13
          |
| 44 | | eqid 2622 |
. . . . . . . . . . . . . 14
   unitVec unitVec      unitVec unitVec   |
| 45 | | eqid 2622 |
. . . . . . . . . . . . . 14
   freeLMod freeLMod      freeLMod freeLMod   |
| 46 | | eqid 2622 |
. . . . . . . . . . . . . 14
      freeLMod freeLMod
         freeLMod freeLMod
   |
| 47 | 44, 45, 46 | uvcff 20130 |
. . . . . . . . . . . . 13
              unitVec unitVec           freeLMod freeLMod     |
| 48 | 43, 47 | sylan 488 |
. . . . . . . . . . . 12
              unitVec unitVec           freeLMod freeLMod     |
| 49 | 48 | ffvelrnda 6359 |
. . . . . . . . . . 11
         
   
   
   unitVec unitVec            freeLMod freeLMod
    |
| 50 | 49 | ad4ant14 1293 |
. . . . . . . . . 10
         
   
 curry curry                curry curry  LIndF LIndF   freeLMod freeLMod      
      unitVec unitVec            freeLMod freeLMod     |
| 51 | | ffn 6045 |
. . . . . . . . . . . . . . . 16
  curry curry               curry curry     |
| 52 | | fnima 6010 |
. . . . . . . . . . . . . . . 16
  curry curry   
  curry curry       curry curry   |
| 53 | 51, 52 | syl 17 |
. . . . . . . . . . . . . . 15
  curry curry                curry curry       curry curry   |
| 54 | 53 | adantl 482 |
. . . . . . . . . . . . . 14
         
   curry curry                 curry curry       curry curry
  |
| 55 | 54 | fveq2d 6195 |
. . . . . . . . . . . . 13
         
   curry curry                      freeLMod freeLMod      curry curry             freeLMod freeLMod      curry curry    |
| 56 | 55 | adantr 481 |
. . . . . . . . . . . 12
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod           freeLMod freeLMod      curry curry             freeLMod freeLMod      curry curry    |
| 57 | | simplll 798 |
. . . . . . . . . . . . . 14
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod      
  |
| 58 | | simpllr 799 |
. . . . . . . . . . . . . 14
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod      
  |
| 59 | 45 | frlmlmod 20093 |
. . . . . . . . . . . . . . . . 17
              freeLMod freeLMod      |
| 60 | 43, 59 | sylan 488 |
. . . . . . . . . . . . . . . 16
              freeLMod freeLMod      |
| 61 | 60 | adantr 481 |
. . . . . . . . . . . . . . 15
         
   curry curry                  freeLMod freeLMod
     |
| 62 | | lindfrn 20160 |
. . . . . . . . . . . . . . 15
     freeLMod freeLMod
     curry
curry  LIndF LIndF   freeLMod freeLMod      curry curry    LIndS LIndS   freeLMod
freeLMod     |
| 63 | 61, 62 | sylan 488 |
. . . . . . . . . . . . . 14
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod      curry curry    LIndS LIndS   freeLMod
freeLMod     |
| 64 | 45 | frlmsca 20097 |
. . . . . . . . . . . . . . . . . . . . . 22
               Scalar Scalar   freeLMod freeLMod     |
| 65 | | drngnzr 19262 |
. . . . . . . . . . . . . . . . . . . . . . 23
        NzRing NzRing |
| 66 | 65 | adantr 481 |
. . . . . . . . . . . . . . . . . . . . . 22
              NzRing NzRing |
| 67 | 64, 66 | eqeltrrd 2702 |
. . . . . . . . . . . . . . . . . . . . 21
             Scalar Scalar   freeLMod freeLMod
    NzRing
NzRing |
| 68 | 60, 67 | jca 554 |
. . . . . . . . . . . . . . . . . . . 20
               freeLMod freeLMod
    
 Scalar Scalar   freeLMod freeLMod     NzRing NzRing  |
| 69 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . . 22
  Scalar Scalar   freeLMod
freeLMod      Scalar Scalar   freeLMod freeLMod    |
| 70 | 46, 69 | lindff1 20159 |
. . . . . . . . . . . . . . . . . . . . 21
     freeLMod freeLMod
    
 Scalar Scalar   freeLMod freeLMod     NzRing NzRing  curry curry  LIndF LIndF   freeLMod freeLMod     curry
curry    curry curry        freeLMod freeLMod     |
| 71 | 70 | 3expa 1265 |
. . . . . . . . . . . . . . . . . . . 20
      freeLMod freeLMod    
  Scalar Scalar   freeLMod freeLMod
    NzRing
NzRing  curry curry  LIndF LIndF   freeLMod freeLMod     curry curry    curry curry
       freeLMod freeLMod
    |
| 72 | 68, 71 | sylan 488 |
. . . . . . . . . . . . . . . . . . 19
         
   curry curry  LIndF LIndF   freeLMod freeLMod     curry
curry    curry curry        freeLMod freeLMod     |
| 73 | | fdm 6051 |
. . . . . . . . . . . . . . . . . . 19
  curry curry                curry curry     |
| 74 | | f1eq2 6097 |
. . . . . . . . . . . . . . . . . . . 20
   curry curry  
   curry curry    curry curry        freeLMod freeLMod     curry
curry          freeLMod freeLMod      |
| 75 | 74 | biimpac 503 |
. . . . . . . . . . . . . . . . . . 19
   curry curry    curry curry        freeLMod freeLMod      curry curry      curry curry          freeLMod freeLMod
    |
| 76 | 72, 73, 75 | syl2an 494 |
. . . . . . . . . . . . . . . . . 18
              curry curry  LIndF
LIndF   freeLMod freeLMod     curry curry                curry curry          freeLMod freeLMod     |
| 77 | 76 | an32s 846 |
. . . . . . . . . . . . . . . . 17
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod     curry curry          freeLMod freeLMod
    |
| 78 | | f1f1orn 6148 |
. . . . . . . . . . . . . . . . 17
  curry curry          freeLMod freeLMod
    curry
curry      curry curry   |
| 79 | 77, 78 | syl 17 |
. . . . . . . . . . . . . . . 16
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod     curry curry      curry curry   |
| 80 | | f1oeng 7974 |
. . . . . . . . . . . . . . . 16
       curry curry      curry curry       curry curry   |
| 81 | 58, 79, 80 | syl2anc 693 |
. . . . . . . . . . . . . . 15
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod        curry
curry   |
| 82 | 81 | ensymd 8007 |
. . . . . . . . . . . . . 14
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod      curry curry     |
| 83 | | lindsenlbs 33404 |
. . . . . . . . . . . . . 14
         
   curry curry    LIndS LIndS   freeLMod freeLMod       curry
curry     
 curry curry    LBasis LBasis   freeLMod freeLMod     |
| 84 | 57, 58, 63, 82, 83 | syl31anc 1329 |
. . . . . . . . . . . . 13
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod      curry curry    LBasis LBasis   freeLMod
freeLMod     |
| 85 | | eqid 2622 |
. . . . . . . . . . . . . 14
  LBasis LBasis   freeLMod
freeLMod      LBasis LBasis   freeLMod freeLMod    |
| 86 | | eqid 2622 |
. . . . . . . . . . . . . 14
      freeLMod freeLMod
         freeLMod freeLMod
   |
| 87 | 46, 85, 86 | lbssp 19079 |
. . . . . . . . . . . . 13
   curry curry  
 LBasis LBasis   freeLMod freeLMod           freeLMod freeLMod      curry curry         freeLMod freeLMod     |
| 88 | 84, 87 | syl 17 |
. . . . . . . . . . . 12
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod           freeLMod freeLMod      curry curry         freeLMod freeLMod     |
| 89 | 56, 88 | eqtrd 2656 |
. . . . . . . . . . 11
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod           freeLMod freeLMod      curry curry            freeLMod freeLMod
    |
| 90 | 89 | adantr 481 |
. . . . . . . . . 10
         
   
 curry curry                curry curry  LIndF LIndF   freeLMod freeLMod      
         freeLMod freeLMod      curry curry            freeLMod freeLMod
    |
| 91 | 50, 90 | eleqtrrd 2704 |
. . . . . . . . 9
         
   
 curry curry                curry curry  LIndF LIndF   freeLMod freeLMod      
      unitVec unitVec             freeLMod freeLMod      curry curry       |
| 92 | | eqid 2622 |
. . . . . . . . . . . . 13
     Scalar Scalar   freeLMod freeLMod          Scalar Scalar   freeLMod freeLMod
    |
| 93 | | eqid 2622 |
. . . . . . . . . . . . 13
     Scalar Scalar   freeLMod freeLMod          Scalar Scalar   freeLMod freeLMod
    |
| 94 | | eqid 2622 |
. . . . . . . . . . . . 13
      freeLMod freeLMod          freeLMod freeLMod    |
| 95 | 45, 14 | frlmfibas 20105 |
. . . . . . . . . . . . . . 15
                           freeLMod freeLMod     |
| 96 | 95 | feq3d 6032 |
. . . . . . . . . . . . . 14
             curry curry               curry
curry          freeLMod freeLMod      |
| 97 | 96 | biimpa 501 |
. . . . . . . . . . . . 13
             curry curry                curry curry          freeLMod freeLMod
    |
| 98 | 59 | adantr 481 |
. . . . . . . . . . . . 13
             curry curry                  freeLMod freeLMod
     |
| 99 | | simplr 792 |
. . . . . . . . . . . . 13
             curry curry                 
  |
| 100 | 86, 46, 92, 69, 93, 94, 97, 98, 99 | elfilspd 20142 |
. . . . . . . . . . . 12
             curry curry                    unitVec unitVec             freeLMod freeLMod      curry curry      
        Scalar Scalar   freeLMod freeLMod
          unitVec unitVec          freeLMod freeLMod    g g          freeLMod freeLMod    curry curry      |
| 101 | 45 | frlmsca 20097 |
. . . . . . . . . . . . . . . 16
               Scalar Scalar   freeLMod freeLMod     |
| 102 | 101 | fveq2d 6195 |
. . . . . . . . . . . . . . 15
                      Scalar Scalar   freeLMod freeLMod
     |
| 103 | 102 | oveq1d 6665 |
. . . . . . . . . . . . . 14
                           Scalar Scalar   freeLMod freeLMod
        |
| 104 | 103 | adantr 481 |
. . . . . . . . . . . . 13
             curry curry                      
        Scalar Scalar   freeLMod freeLMod         |
| 105 | | elmapi 7879 |
. . . . . . . . . . . . . . 15
             
          |
| 106 | | ffn 6045 |
. . . . . . . . . . . . . . . . . . . 20
                |
| 107 | 106 | adantl 482 |
. . . . . . . . . . . . . . . . . . 19
              curry curry           
   
               |
| 108 | 51 | ad2antlr 763 |
. . . . . . . . . . . . . . . . . . 19
              curry curry           
   
           curry curry     |
| 109 | | simpllr 799 |
. . . . . . . . . . . . . . . . . . 19
              curry curry           
   
               |
| 110 | | inidm 3822 |
. . . . . . . . . . . . . . . . . . 19
        |
| 111 | | eqidd 2623 |
. . . . . . . . . . . . . . . . . . 19
        
      curry curry                            
               |
| 112 | | eqidd 2623 |
. . . . . . . . . . . . . . . . . . 19
        
      curry curry                            
    curry curry       curry curry      |
| 113 | 107, 108,
109, 109, 110, 111, 112 | offval 6904 |
. . . . . . . . . . . . . . . . . 18
              curry curry           
   
                    freeLMod freeLMod    curry curry                    freeLMod freeLMod     curry curry        |
| 114 | | simp-4r 807 |
. . . . . . . . . . . . . . . . . . . . 21
        
      curry curry                            
       |
| 115 | | ffvelrn 6357 |
. . . . . . . . . . . . . . . . . . . . . 22
                              |
| 116 | 115 | adantll 750 |
. . . . . . . . . . . . . . . . . . . . 21
        
      curry curry                            
               |
| 117 | | ffvelrn 6357 |
. . . . . . . . . . . . . . . . . . . . . . 23
   curry curry                     curry curry            
   |
| 118 | 117 | ad4ant24 1298 |
. . . . . . . . . . . . . . . . . . . . . 22
        
      curry curry                            
    curry curry                |
| 119 | 95 | ad3antrrr 766 |
. . . . . . . . . . . . . . . . . . . . . 22
        
      curry curry                            
         
        freeLMod freeLMod     |
| 120 | 118, 119 | eleqtrd 2703 |
. . . . . . . . . . . . . . . . . . . . 21
        
      curry curry                            
    curry curry           freeLMod freeLMod
    |
| 121 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . 21
            |
| 122 | 45, 46, 14, 114, 116, 120, 94, 121 | frlmvscafval 20109 |
. . . . . . . . . . . . . . . . . . . 20
        
      curry curry                            
              freeLMod freeLMod     curry curry                           curry curry       |
| 123 | | fvex 6201 |
. . . . . . . . . . . . . . . . . . . . . 22
        |
| 124 | | fnconstg 6093 |
. . . . . . . . . . . . . . . . . . . . . 22
                        |
| 125 | 123, 124 | mp1i 13 |
. . . . . . . . . . . . . . . . . . . . 21
        
      curry curry                            
                 |
| 126 | | elmapfn 7880 |
. . . . . . . . . . . . . . . . . . . . . . 23
   curry curry            
    curry curry        |
| 127 | 117, 126 | syl 17 |
. . . . . . . . . . . . . . . . . . . . . 22
   curry curry                     curry curry        |
| 128 | 127 | ad4ant24 1298 |
. . . . . . . . . . . . . . . . . . . . 21
        
      curry curry                            
    curry curry        |
| 129 | 123 | fvconst2 6469 |
. . . . . . . . . . . . . . . . . . . . . 22
                            |
| 130 | 129 | adantl 482 |
. . . . . . . . . . . . . . . . . . . . 21
                curry curry                            
   
   
                      |
| 131 | | eqidd 2623 |
. . . . . . . . . . . . . . . . . . . . 21
                curry curry                            
   
   
  curry curry           curry curry         |
| 132 | 125, 128,
114, 114, 110, 130, 131 | offval 6904 |
. . . . . . . . . . . . . . . . . . . 20
        
      curry curry                            
                       curry curry                         curry curry           |
| 133 | 122, 132 | eqtrd 2656 |
. . . . . . . . . . . . . . . . . . 19
        
      curry curry                            
              freeLMod freeLMod     curry curry                         curry curry           |
| 134 | 133 | mpteq2dva 4744 |
. . . . . . . . . . . . . . . . . 18
              curry curry           
   
                           freeLMod
freeLMod     curry curry                               curry curry            |
| 135 | 113, 134 | eqtrd 2656 |
. . . . . . . . . . . . . . . . 17
              curry curry           
   
                    freeLMod freeLMod    curry curry                           curry curry            |
| 136 | 135 | oveq2d 6666 |
. . . . . . . . . . . . . . . 16
              curry curry           
   
              freeLMod freeLMod
   g g          freeLMod freeLMod    curry curry        freeLMod freeLMod
   g g                        curry curry             |
| 137 | | eqid 2622 |
. . . . . . . . . . . . . . . . 17
      freeLMod freeLMod          freeLMod freeLMod    |
| 138 | | simplll 798 |
. . . . . . . . . . . . . . . . 17
              curry curry           
   
               |
| 139 | | simp-5l 808 |
. . . . . . . . . . . . . . . . . . . 20
                curry curry                            
   
   
    |
| 140 | 115 | ad4ant23 1297 |
. . . . . . . . . . . . . . . . . . . 20
                curry curry                            
   
   
            |
| 141 | | simplr 792 |
. . . . . . . . . . . . . . . . . . . . 21
              curry curry           
   
           curry curry               |
| 142 | | elmapi 7879 |
. . . . . . . . . . . . . . . . . . . . . . 23
   curry curry            
    curry curry              |
| 143 | 117, 142 | syl 17 |
. . . . . . . . . . . . . . . . . . . . . 22
   curry curry                     curry curry              |
| 144 | 143 | ffvelrnda 6359 |
. . . . . . . . . . . . . . . . . . . . 21
    curry curry           
   
   
       curry curry               |
| 145 | 141, 144 | sylanl1 682 |
. . . . . . . . . . . . . . . . . . . 20
                curry curry                            
   
   
  curry curry               |
| 146 | 14, 121 | ringcl 18561 |
. . . . . . . . . . . . . . . . . . . 20
                  
  curry curry               
             curry curry                |
| 147 | 139, 140,
145, 146 | syl3anc 1326 |
. . . . . . . . . . . . . . . . . . 19
                curry curry                            
   
   
             curry curry                |
| 148 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . 19
                   curry curry                             curry curry          |
| 149 | 147, 148 | fmptd 6385 |
. . . . . . . . . . . . . . . . . 18
        
      curry curry                            
                     curry curry                   |
| 150 | | elmapg 7870 |
. . . . . . . . . . . . . . . . . . . . . 22
            
                      curry curry                 
                     curry curry                    |
| 151 | 22, 150 | mpan 706 |
. . . . . . . . . . . . . . . . . . . . 21
                         curry curry                 
                     curry curry                    |
| 152 | 151 | adantl 482 |
. . . . . . . . . . . . . . . . . . . 20
                               curry curry                 
                     curry curry                    |
| 153 | 95 | eleq2d 2687 |
. . . . . . . . . . . . . . . . . . . 20
                               curry curry                 
                     curry curry                freeLMod freeLMod      |
| 154 | 152, 153 | bitr3d 270 |
. . . . . . . . . . . . . . . . . . 19
                               curry curry                                     curry curry                freeLMod freeLMod      |
| 155 | 154 | ad3antrrr 766 |
. . . . . . . . . . . . . . . . . 18
        
      curry curry                            
                      curry curry                                     curry curry                freeLMod freeLMod      |
| 156 | 149, 155 | mpbid 222 |
. . . . . . . . . . . . . . . . 17
        
      curry curry                            
                     curry curry                freeLMod freeLMod     |
| 157 | | mptexg 6484 |
. . . . . . . . . . . . . . . . . . . . 21
                        curry curry             |
| 158 | 157 | ralrimivw 2967 |
. . . . . . . . . . . . . . . . . . . 20
         
   
              curry curry             |
| 159 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . 21
                        curry curry                                   curry curry           |
| 160 | 159 | fnmpt 6020 |
. . . . . . . . . . . . . . . . . . . 20
    
                   curry curry                                    curry curry              |
| 161 | 158, 160 | syl 17 |
. . . . . . . . . . . . . . . . . . 19
                             curry curry              |
| 162 | | id 22 |
. . . . . . . . . . . . . . . . . . 19
          |
| 163 | | fvexd 6203 |
. . . . . . . . . . . . . . . . . . 19
           freeLMod freeLMod       |
| 164 | 161, 162,
163 | fndmfifsupp 8288 |
. . . . . . . . . . . . . . . . . 18
                             curry curry           finSupp finSupp      freeLMod
freeLMod     |
| 165 | 164 | ad3antlr 767 |
. . . . . . . . . . . . . . . . 17
              curry curry           
   
                                  curry curry           finSupp finSupp      freeLMod
freeLMod     |
| 166 | 45, 46, 137, 109, 109, 138, 156, 165 | frlmgsum 20111 |
. . . . . . . . . . . . . . . 16
              curry curry           
   
              freeLMod freeLMod
   g g                        curry curry                     g g                   curry curry             |
| 167 | 136, 166 | eqtr2d 2657 |
. . . . . . . . . . . . . . 15
              curry curry           
   
                   g g                   curry curry                freeLMod freeLMod    g g          freeLMod freeLMod    curry curry     |
| 168 | 105, 167 | sylan2 491 |
. . . . . . . . . . . . . 14
              curry curry           
   
        
   
   
    g g                   curry curry                freeLMod freeLMod    g g          freeLMod freeLMod    curry curry     |
| 169 | 168 | eqeq2d 2632 |
. . . . . . . . . . . . 13
              curry curry           
   
        
   
    unitVec unitVec
              g g                   curry curry                unitVec unitVec          freeLMod freeLMod    g g          freeLMod freeLMod    curry curry      |
| 170 | 104, 169 | rexeqbidva 3155 |
. . . . . . . . . . . 12
             curry curry                          
     unitVec unitVec               g g                   curry curry                     Scalar Scalar   freeLMod freeLMod
          unitVec unitVec          freeLMod freeLMod    g g          freeLMod freeLMod    curry curry      |
| 171 | 100, 170 | bitr4d 271 |
. . . . . . . . . . 11
             curry curry                    unitVec unitVec         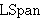    freeLMod freeLMod      curry curry      
         
     unitVec unitVec               g g                   curry curry              |
| 172 | 43, 171 | sylanl1 682 |
. . . . . . . . . 10
         
   curry curry                    unitVec unitVec         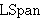    freeLMod freeLMod      curry curry      
         
     unitVec unitVec               g g                   curry curry              |
| 173 | 172 | ad2antrr 762 |
. . . . . . . . 9
         
   
 curry curry                curry curry  LIndF LIndF   freeLMod freeLMod      
       unitVec unitVec         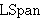    freeLMod freeLMod      curry curry      
         
     unitVec unitVec               g g                   curry curry              |
| 174 | 91, 173 | mpbid 222 |
. . . . . . . 8
         
   
 curry curry                curry curry  LIndF LIndF   freeLMod freeLMod      
            
     unitVec unitVec               g g                   curry curry             |
| 175 | 174 | ralrimiva 2966 |
. . . . . . 7
              curry curry           
    curry
curry  LIndF LIndF   freeLMod freeLMod      
   
             unitVec unitVec               g g                   curry curry             |
| 176 | 42, 175 | sylan 488 |
. . . . . 6
                Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod
   
   
         
     unitVec unitVec               g g                   curry curry             |
| 177 | 10, 21 | mpbird 247 |
. . . . . . . . 9
              Mat Mat     
        
       |
| 178 | | elmapfn 7880 |
. . . . . . . . 9
                 
   
    |
| 179 | 177, 178 | syl 17 |
. . . . . . . 8
              Mat Mat     
   
    |
| 180 | 4 | adantl 482 |
. . . . . . . 8
              Mat Mat     
    |
| 181 | | an32 839 |
. . . . . . . . . . . . . . . . . . 19
                
    
   
                |
| 182 | | df-3an 1039 |
. . . . . . . . . . . . . . . . . . 19
            
   
                 
     |
| 183 | 181, 182 | bitr4i 267 |
. . . . . . . . . . . . . . . . . 18
                
    
        
         |
| 184 | | curfv 33389 |
. . . . . . . . . . . . . . . . . 18
                
   
   
   curry curry               |
| 185 | 183, 184 | sylanb 489 |
. . . . . . . . . . . . . . . . 17
        
   
                 curry curry               |
| 186 | 185 | an32s 846 |
. . . . . . . . . . . . . . . 16
        
   
                 curry curry               |
| 187 | 186 | oveq2d 6666 |
. . . . . . . . . . . . . . 15
        
   
                            curry curry                            |
| 188 | 187 | mpteq2dva 4744 |
. . . . . . . . . . . . . 14
                
   
                   curry curry                                   |
| 189 | 188 | an32s 846 |
. . . . . . . . . . . . 13
                     
   
              curry curry                                   |
| 190 | 189 | oveq2d 6666 |
. . . . . . . . . . . 12
                     
   g g                   curry curry               g g                          |
| 191 | 190 | mpteq2dva 4744 |
. . . . . . . . . . 11
            
           g g                   curry curry                     g g                           |
| 192 | 191 | eqeq2d 2632 |
. . . . . . . . . 10
            
       unitVec unitVec               g g                   curry curry                unitVec unitVec               g g                            |
| 193 | 192 | rexbidv 3052 |
. . . . . . . . 9
            
             
     unitVec unitVec               g g                   curry curry                      
     unitVec unitVec               g g                            |
| 194 | 193 | ralbidv 2986 |
. . . . . . . 8
            
                 
     unitVec unitVec               g g                   curry curry                  
             unitVec unitVec               g g                            |
| 195 | 179, 180,
194 | syl2anc 693 |
. . . . . . 7
              Mat Mat     
   
   
            unitVec unitVec               g g                   curry curry              
   
             unitVec unitVec               g g                            |
| 196 | 195 | ad2antrr 762 |
. . . . . 6
                Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod
   
   
   
            unitVec unitVec               g g                   curry curry              
   
             unitVec unitVec               g g                            |
| 197 | 176, 196 | mpbid 222 |
. . . . 5
                Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod
   
   
         
     unitVec unitVec               g g                           |
| 198 | 8, 197 | sylanl1 682 |
. . . 4
       Field Field  
      Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod      
   
             unitVec unitVec               g g                           |
| 199 | | fveq1 6190 |
. . . . . . . . . . 11
                          |
| 200 | | vex 3203 |
. . . . . . . . . . . 12
    |
| 201 | | vex 3203 |
. . . . . . . . . . . 12
    |
| 202 | | uncov 33390 |
. . . . . . . . . . . 12
        
     uncurry uncurry               |
| 203 | 200, 201,
202 | mp2an 708 |
. . . . . . . . . . 11
   uncurry uncurry              |
| 204 | 199, 203 | syl6eqr 2674 |
. . . . . . . . . 10
                  uncurry uncurry     |
| 205 | 204 | oveq1d 6665 |
. . . . . . . . 9
                               uncurry uncurry                |
| 206 | 205 | mpteq2dv 4745 |
. . . . . . . 8
                                          uncurry uncurry                 |
| 207 | 206 | oveq2d 6666 |
. . . . . . 7
             g g                             g g         uncurry uncurry                  |
| 208 | 207 | mpteq2dv 4745 |
. . . . . 6
                  g g                                   g g         uncurry uncurry                   |
| 209 | 208 | eqeq2d 2632 |
. . . . 5
              unitVec unitVec               g g                          
   unitVec unitVec               g g         uncurry uncurry                    |
| 210 | 209 | ac6sfi 8204 |
. . . 4
             
            unitVec unitVec               g g                           
             
          unitVec unitVec               g g         uncurry uncurry                    |
| 211 | 5, 198, 210 | syl2anc 693 |
. . 3
       Field Field  
      Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod                     
   
   unitVec unitVec               g g         uncurry uncurry                    |
| 212 | | uncf 33388 |
. . . . . . 7
                uncurry uncurry               |
| 213 | 13, 14 | frlmfibas 20105 |
. . . . . . . . . . . . . . . 16
     Field Field
                
            freeLMod freeLMod         |
| 214 | 12, 213 | sylan2 491 |
. . . . . . . . . . . . . . 15
     Field Field
                         freeLMod freeLMod         |
| 215 | 1, 13 | matbas 20219 |
. . . . . . . . . . . . . . . 16
         Field
Field       freeLMod freeLMod              Mat Mat     |
| 216 | 215 | ancoms 469 |
. . . . . . . . . . . . . . 15
     Field Field
           freeLMod freeLMod              Mat Mat     |
| 217 | 214, 216 | eqtrd 2656 |
. . . . . . . . . . . . . 14
     Field Field
                         Mat Mat     |
| 218 | 4, 217 | sylan2 491 |
. . . . . . . . . . . . 13
     Field Field
        Mat Mat     
        
          Mat Mat     |
| 219 | 218 | eleq2d 2687 |
. . . . . . . . . . . 12
     Field Field
        Mat Mat     
 uncurry uncurry         
       uncurry
uncurry        Mat Mat      |
| 220 | | elmapg 7870 |
. . . . . . . . . . . . . 14
            
        uncurry uncurry         
       uncurry
uncurry                |
| 221 | 22, 23, 220 | sylancr 695 |
. . . . . . . . . . . . 13
         Mat Mat      uncurry uncurry
        
       uncurry
uncurry                |
| 222 | 221 | adantl 482 |
. . . . . . . . . . . 12
     Field Field
        Mat Mat     
 uncurry uncurry         
       uncurry
uncurry                |
| 223 | 219, 222 | bitr3d 270 |
. . . . . . . . . . 11
     Field Field
        Mat Mat     
 uncurry uncurry        Mat Mat     uncurry uncurry                |
| 224 | 223 | biimpar 502 |
. . . . . . . . . 10
      Field Field         Mat Mat      uncurry
uncurry                uncurry uncurry        Mat Mat     |
| 225 | 224 | adantr 481 |
. . . . . . . . 9
       Field Field  
      Mat Mat      uncurry uncurry                       unitVec unitVec
              g g         uncurry uncurry                    uncurry
uncurry        Mat Mat     |
| 226 | | nfv 1843 |
. . . . . . . . . . . . . 14
        Field Field 
       Mat Mat      uncurry uncurry                    |
| 227 | | nfmpt1 4747 |
. . . . . . . . . . . . . . 15
           g g         uncurry uncurry                  |
| 228 | 227 | nfeq2 2780 |
. . . . . . . . . . . . . 14
      unitVec unitVec               g g         uncurry uncurry                  |
| 229 | | fveq1 6190 |
. . . . . . . . . . . . . . . . 17
     unitVec unitVec
              g g         uncurry uncurry                       unitVec unitVec                   g g         uncurry uncurry                      |
| 230 | 7, 43 | syl 17 |
. . . . . . . . . . . . . . . . . . . . 21
    Field Field      |
| 231 | 230, 4 | anim12i 590 |
. . . . . . . . . . . . . . . . . . . 20
     Field Field
        Mat Mat     
    
     |
| 232 | 231 | adantr 481 |
. . . . . . . . . . . . . . . . . . 19
      Field Field         Mat Mat      uncurry
uncurry                  
       |
| 233 | | equcom 1945 |
. . . . . . . . . . . . . . . . . . . . 21
     
    |
| 234 | | ifbi 4107 |
. . . . . . . . . . . . . . . . . . . . 21
      
     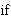                                      |
| 235 | 233, 234 | ax-mp 5 |
. . . . . . . . . . . . . . . . . . . 20
                                      |
| 236 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . 21
            |
| 237 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . 21
            |
| 238 | | simpllr 799 |
. . . . . . . . . . . . . . . . . . . . 21
                  
         |
| 239 | | simplll 798 |
. . . . . . . . . . . . . . . . . . . . 21
                  
         |
| 240 | | simplr 792 |
. . . . . . . . . . . . . . . . . . . . 21
                  
         |
| 241 | | simpr 477 |
. . . . . . . . . . . . . . . . . . . . 21
                  
         |
| 242 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . 21
      Mat Mat          Mat Mat    |
| 243 | 1, 236, 237, 238, 239, 240, 241, 242 | mat1ov 20254 |
. . . . . . . . . . . . . . . . . . . 20
                  
            Mat Mat
                         |
| 244 | | df-3an 1039 |
. . . . . . . . . . . . . . . . . . . . 21
               
   
            |
| 245 | 44, 236, 237 | uvcvval 20125 |
. . . . . . . . . . . . . . . . . . . . 21
             
   
   
    unitVec unitVec
         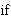                   |
| 246 | 244, 245 | sylanbr 490 |
. . . . . . . . . . . . . . . . . . . 20
                  
         unitVec unitVec          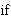                   |
| 247 | 235, 243,
246 | 3eqtr4a 2682 |
. . . . . . . . . . . . . . . . . . 19
                  
            Mat Mat
          unitVec unitVec          |
| 248 | 232, 247 | sylanl1 682 |
. . . . . . . . . . . . . . . . . 18
        Field Field 
       Mat Mat      uncurry uncurry                                 Mat Mat
          unitVec unitVec          |
| 249 | | ovex 6678 |
. . . . . . . . . . . . . . . . . . . . 21
    g g         uncurry uncurry                   |
| 250 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . . . 22
         g g         uncurry uncurry                           g g         uncurry uncurry                  |
| 251 | 250 | fvmpt2 6291 |
. . . . . . . . . . . . . . . . . . . . 21
        
 g g         uncurry uncurry                              g g         uncurry uncurry                         g g         uncurry uncurry                  |
| 252 | 249, 251 | mpan2 707 |
. . . . . . . . . . . . . . . . . . . 20
               g g         uncurry uncurry                         g g         uncurry uncurry                  |
| 253 | 252 | adantl 482 |
. . . . . . . . . . . . . . . . . . 19
        Field Field 
       Mat Mat      uncurry uncurry                                   g g         uncurry uncurry                         g g         uncurry uncurry                  |
| 254 | | eqid 2622 |
. . . . . . . . . . . . . . . . . . . 20
   maMul maMul            maMul maMul         |
| 255 | | simp-4l 806 |
. . . . . . . . . . . . . . . . . . . 20
        Field Field 
       Mat Mat      uncurry uncurry                            Field Field |
| 256 | 4 | ad4antlr 769 |
. . . . . . . . . . . . . . . . . . . 20
        Field Field 
       Mat Mat      uncurry uncurry                              |
| 257 | 221 | biimpar 502 |
. . . . . . . . . . . . . . . . . . . . 21
          Mat Mat     uncurry
uncurry                uncurry uncurry         
       |
| 258 | 257 | ad5ant23 1304 |
. . . . . . . . . . . . . . . . . . . 20
        Field Field 
       Mat Mat      uncurry uncurry                          uncurry uncurry         
       |
| 259 | | simpr 477 |
. . . . . . . . . . . . . . . . . . . . . 22
     Field Field
        Mat Mat     
       Mat Mat     |
| 260 | 259, 218 | eleqtrrd 2704 |
. . . . . . . . . . . . . . . . . . . . 21
     Field Field
        Mat Mat     
        
       |
| 261 | 260 | ad3antrrr 766 |
. . . . . . . . . . . . . . . . . . . 20
        Field Field 
       Mat Mat      uncurry uncurry                                  
       |
| 262 | | simplr 792 |
. . . . . . . . . . . . . . . . . . . 20
        Field Field 
       Mat Mat      uncurry uncurry                              |
| 263 | | simpr 477 |
. . . . . . . . . . . . . . . . . . . 20
        Field Field 
       Mat Mat      uncurry uncurry                              |
| 264 | 254, 14, 121, 255, 256, 256, 256, 258, 261, 262, 263 | mamufv 20193 |
. . . . . . . . . . . . . . . . . . 19
        Field Field 
       Mat Mat      uncurry uncurry                             uncurry uncurry
   maMul maMul                 g g         uncurry uncurry                  |
| 265 | 1, 254 | matmulr 20244 |
. . . . . . . . . . . . . . . . . . . . . . . 24
         Field
Field    maMul maMul               Mat Mat     |
| 266 | 265 | ancoms 469 |
. . . . . . . . . . . . . . . . . . . . . . 23
     Field Field
        maMul maMul               Mat Mat     |
| 267 | 266 | oveqd 6667 |
. . . . . . . . . . . . . . . . . . . . . 22
     Field Field
       uncurry uncurry    maMul maMul             uncurry uncurry       Mat Mat
      |
| 268 | 267 | oveqd 6667 |
. . . . . . . . . . . . . . . . . . . . 21
     Field Field
         uncurry uncurry
   maMul maMul                 uncurry uncurry       Mat Mat         |
| 269 | 4, 268 | sylan2 491 |
. . . . . . . . . . . . . . . . . . . 20
     Field Field
        Mat Mat     
   uncurry uncurry    maMul maMul                 uncurry uncurry       Mat Mat
        |
| 270 | 269 | ad3antrrr 766 |
. . . . . . . . . . . . . . . . . . 19
        Field Field 
       Mat Mat      uncurry uncurry                             uncurry uncurry
   maMul maMul                 uncurry uncurry       Mat Mat         |
| 271 | 253, 264,
270 | 3eqtr2rd 2663 |
. . . . . . . . . . . . . . . . . 18
        Field Field 
       Mat Mat      uncurry uncurry                             uncurry uncurry
      Mat Mat                  g g         uncurry uncurry                      |
| 272 | 248, 271 | eqeq12d 2637 |
. . . . . . . . . . . . . . . . 17
        Field Field 
       Mat Mat      uncurry uncurry                                  Mat Mat          uncurry uncurry       Mat Mat
            unitVec unitVec                   g g         uncurry uncurry                       |
| 273 | 229, 272 | syl5ibr 236 |
. . . . . . . . . . . . . . . 16
        Field Field 
       Mat Mat      uncurry uncurry                              unitVec unitVec               g g         uncurry uncurry                          Mat Mat
         uncurry uncurry       Mat Mat          |
| 274 | 273 | ex 450 |
. . . . . . . . . . . . . . 15
       Field Field  
      Mat Mat      uncurry uncurry                 
            unitVec unitVec               g g         uncurry uncurry                          Mat Mat          uncurry uncurry       Mat Mat           |
| 275 | 274 | com23 86 |
. . . . . . . . . . . . . 14
       Field Field  
      Mat Mat      uncurry uncurry                 
       unitVec unitVec               g g         uncurry uncurry                       
       Mat Mat          uncurry uncurry       Mat Mat           |
| 276 | 226, 228,
275 | ralrimd 2959 |
. . . . . . . . . . . . 13
       Field Field  
      Mat Mat      uncurry uncurry                 
       unitVec unitVec               g g         uncurry uncurry                              Mat Mat          uncurry uncurry       Mat Mat
         |
| 277 | 276 | ralimdva 2962 |
. . . . . . . . . . . 12
      Field Field         Mat Mat      uncurry
uncurry                  
     unitVec unitVec               g g         uncurry uncurry                      
   
       Mat Mat          uncurry uncurry       Mat Mat          |
| 278 | 1, 2, 242 | mat1bas 20255 |
. . . . . . . . . . . . . . . . 17
                 Mat Mat          Mat Mat     |
| 279 | 13, 14 | frlmfibas 20105 |
. . . . . . . . . . . . . . . . . . 19
                      
            freeLMod freeLMod         |
| 280 | 12, 279 | sylan2 491 |
. . . . . . . . . . . . . . . . . 18
                               freeLMod freeLMod         |
| 281 | 1, 13 | matbas 20219 |
. . . . . . . . . . . . . . . . . . 19
        
        freeLMod freeLMod              Mat Mat     |
| 282 | 281 | ancoms 469 |
. . . . . . . . . . . . . . . . . 18
                 freeLMod freeLMod              Mat Mat     |
| 283 | 280, 282 | eqtrd 2656 |
. . . . . . . . . . . . . . . . 17
                               Mat Mat     |
| 284 | 278, 283 | eleqtrrd 2704 |
. . . . . . . . . . . . . . . 16
                 Mat Mat           
       |
| 285 | | elmapfn 7880 |
. . . . . . . . . . . . . . . 16
       Mat Mat           
            Mat Mat           |
| 286 | 284, 285 | syl 17 |
. . . . . . . . . . . . . . 15
                 Mat Mat           |
| 287 | 230, 4, 286 | syl2an 494 |
. . . . . . . . . . . . . 14
     Field Field
        Mat Mat     
     Mat Mat           |
| 288 | 287 | adantr 481 |
. . . . . . . . . . . . 13
      Field Field         Mat Mat      uncurry
uncurry                     Mat Mat           |
| 289 | 1 | matring 20249 |
. . . . . . . . . . . . . . . . . 18
        
     Mat Mat      |
| 290 | 4, 230, 289 | syl2anr 495 |
. . . . . . . . . . . . . . . . 17
     Field Field
        Mat Mat     
  Mat Mat      |
| 291 | 290 | adantr 481 |
. . . . . . . . . . . . . . . 16
      Field Field         Mat Mat      uncurry
uncurry                  Mat Mat      |
| 292 | | simplr 792 |
. . . . . . . . . . . . . . . 16
      Field Field         Mat Mat      uncurry
uncurry                       Mat Mat     |
| 293 | | eqid 2622 |
. . . . . . . . . . . . . . . . 17
      Mat Mat          Mat Mat    |
| 294 | 2, 293 | ringcl 18561 |
. . . . . . . . . . . . . . . 16
     Mat Mat    
 uncurry uncurry        Mat Mat            Mat Mat       uncurry uncurry
      Mat Mat            Mat Mat     |
| 295 | 291, 224,
292, 294 | syl3anc 1326 |
. . . . . . . . . . . . . . 15
      Field Field         Mat Mat      uncurry
uncurry                 uncurry uncurry       Mat Mat            Mat Mat     |
| 296 | 218 | adantr 481 |
. . . . . . . . . . . . . . 15
      Field Field         Mat Mat      uncurry
uncurry                                   Mat Mat     |
| 297 | 295, 296 | eleqtrrd 2704 |
. . . . . . . . . . . . . 14
      Field Field         Mat Mat      uncurry
uncurry                 uncurry uncurry       Mat Mat             
       |
| 298 | | elmapfn 7880 |
. . . . . . . . . . . . . 14
   uncurry uncurry       Mat Mat
            
        uncurry uncurry       Mat Mat
            |
| 299 | 297, 298 | syl 17 |
. . . . . . . . . . . . 13
      Field Field         Mat Mat      uncurry
uncurry                 uncurry uncurry       Mat Mat             |
| 300 | | eqfnov2 6767 |
. . . . . . . . . . . . 13
        Mat Mat            uncurry uncurry       Mat Mat             
      Mat Mat      uncurry uncurry       Mat Mat
     
   
   
       Mat Mat          uncurry uncurry       Mat Mat          |
| 301 | 288, 299,
300 | syl2anc 693 |
. . . . . . . . . . . 12
      Field Field         Mat Mat      uncurry
uncurry                      Mat Mat      uncurry uncurry       Mat Mat            
         Mat Mat          uncurry uncurry       Mat Mat
         |
| 302 | 277, 301 | sylibrd 249 |
. . . . . . . . . . 11
      Field Field         Mat Mat      uncurry
uncurry                  
     unitVec unitVec               g g         uncurry uncurry                        Mat Mat      uncurry uncurry       Mat Mat
       |
| 303 | 302 | imp 445 |
. . . . . . . . . 10
       Field Field  
      Mat Mat      uncurry uncurry                       unitVec unitVec
              g g         uncurry uncurry                   
     Mat Mat      uncurry uncurry       Mat Mat       |
| 304 | 303 | eqcomd 2628 |
. . . . . . . . 9
       Field Field  
      Mat Mat      uncurry uncurry                       unitVec unitVec
              g g         uncurry uncurry                   
 uncurry uncurry       Mat Mat            Mat Mat     |
| 305 | | oveq1 6657 |
. . . . . . . . . . 11
    uncurry uncurry          Mat Mat
       uncurry uncurry       Mat Mat       |
| 306 | 305 | eqeq1d 2624 |
. . . . . . . . . 10
    uncurry uncurry           Mat Mat            Mat Mat
     uncurry uncurry       Mat Mat            Mat Mat
     |
| 307 | 306 | rspcev 3309 |
. . . . . . . . 9
   uncurry uncurry        Mat Mat      uncurry uncurry       Mat Mat            Mat Mat              Mat Mat           Mat Mat
           Mat Mat     |
| 308 | 225, 304,
307 | syl2anc 693 |
. . . . . . . 8
       Field Field  
      Mat Mat      uncurry uncurry                       unitVec unitVec
              g g         uncurry uncurry                   
        Mat Mat           Mat Mat            Mat Mat
    |
| 309 | 308 | expl 648 |
. . . . . . 7
     Field Field
        Mat Mat     
  uncurry uncurry                 
    unitVec unitVec
              g g         uncurry uncurry                   
        Mat Mat           Mat Mat            Mat Mat
     |
| 310 | 212, 309 | sylani 686 |
. . . . . 6
     Field Field
        Mat Mat     
                  
    unitVec unitVec
              g g         uncurry uncurry                   
        Mat Mat           Mat Mat            Mat Mat
     |
| 311 | 310 | exlimdv 1861 |
. . . . 5
     Field Field
        Mat Mat     
                 
   
   unitVec unitVec               g g         uncurry uncurry                   
        Mat Mat           Mat Mat            Mat Mat
     |
| 312 | 311 | imp 445 |
. . . 4
      Field Field         Mat Mat                   
          unitVec unitVec               g g         uncurry uncurry                             Mat Mat           Mat Mat
           Mat Mat     |
| 313 | 312 | adantlr 751 |
. . 3
       Field Field  
      Mat Mat                           
   
   unitVec unitVec               g g         uncurry uncurry                             Mat Mat           Mat Mat            Mat Mat
    |
| 314 | 211, 313 | syldan 487 |
. 2
       Field Field  
      Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod      
      Mat Mat           Mat Mat            Mat Mat     |
| 315 | 6 | simprbi 480 |
. . . 4
    Field Field      |
| 316 | | eqid 2622 |
. . . . . . . . . 10
   maDet maDet      maDet maDet   |
| 317 | 316, 1, 2, 14 | mdetcl 20402 |
. . . . . . . . 9
              Mat Mat     
   maDet maDet             |
| 318 | 316, 1, 2, 14 | mdetcl 20402 |
. . . . . . . . 9
              Mat Mat     
   maDet maDet             |
| 319 | | eqid 2622 |
. . . . . . . . . 10
   r r      r r   |
| 320 | 14, 319, 121 | dvdsrmul 18648 |
. . . . . . . . 9
      maDet maDet            
   maDet maDet                 maDet maDet        r r       maDet maDet              maDet maDet        |
| 321 | 317, 318,
320 | syl2an 494 |
. . . . . . . 8
               Mat Mat        
         Mat Mat          maDet maDet
       r r       maDet maDet              maDet maDet        |
| 322 | 321 | anandis 873 |
. . . . . . 7
         
     Mat Mat            Mat Mat          maDet maDet
       r r       maDet maDet              maDet maDet        |
| 323 | 322 | anassrs 680 |
. . . . . 6
               Mat Mat             Mat Mat     
   maDet maDet        r r       maDet maDet              maDet maDet        |
| 324 | 323 | adantrr 753 |
. . . . 5
               Mat Mat              Mat Mat            Mat Mat            Mat Mat      
   maDet maDet        r r       maDet maDet              maDet maDet        |
| 325 | | fveq2 6191 |
. . . . . . . . 9
         Mat Mat
           Mat Mat        maDet maDet           Mat Mat
          maDet maDet         Mat Mat      |
| 326 | 1, 2, 316, 121, 293 | mdetmul 20429 |
. . . . . . . . . . . 12
              Mat Mat            Mat Mat         maDet maDet           Mat Mat
           maDet maDet              maDet maDet        |
| 327 | 326 | 3expa 1265 |
. . . . . . . . . . 11
               Mat Mat             Mat Mat     
   maDet maDet           Mat Mat
           maDet maDet              maDet maDet        |
| 328 | 327 | an32s 846 |
. . . . . . . . . 10
               Mat Mat             Mat Mat     
   maDet maDet           Mat Mat
           maDet maDet              maDet maDet        |
| 329 | 316, 1, 242, 236 | mdet1 20407 |
. . . . . . . . . . . 12
               maDet maDet
        Mat Mat            |
| 330 | 4, 329 | sylan2 491 |
. . . . . . . . . . 11
              Mat Mat     
   maDet maDet         Mat Mat            |
| 331 | 330 | adantr 481 |
. . . . . . . . . 10
               Mat Mat             Mat Mat     
   maDet maDet         Mat Mat            |
| 332 | 328, 331 | eqeq12d 2637 |
. . . . . . . . 9
               Mat Mat             Mat Mat     
    maDet maDet
          Mat Mat           maDet maDet         Mat Mat          maDet maDet              maDet maDet               |
| 333 | 325, 332 | syl5ib 234 |
. . . . . . . 8
               Mat Mat             Mat Mat     
        Mat Mat
           Mat Mat         maDet maDet              maDet maDet               |
| 334 | 333 | impr 649 |
. . . . . . 7
               Mat Mat              Mat Mat            Mat Mat            Mat Mat      
    maDet maDet
             maDet maDet              |
| 335 | 334 | breq2d 4665 |
. . . . . 6
               Mat Mat              Mat Mat            Mat Mat            Mat Mat      
    maDet maDet
       r r       maDet maDet              maDet maDet           maDet maDet        r r          |
| 336 | | eqid 2622 |
. . . . . . . 8
  Unit Unit     Unit Unit   |
| 337 | 336, 236,
319 | crngunit 18662 |
. . . . . . 7
     
    maDet maDet
       Unit Unit   
   maDet maDet        r r          |
| 338 | 337 | ad2antrr 762 |
. . . . . 6
               Mat Mat              Mat Mat            Mat Mat            Mat Mat      
    maDet maDet
       Unit Unit   
   maDet maDet        r r          |
| 339 | 335, 338 | bitr4d 271 |
. . . . 5
               Mat Mat              Mat Mat            Mat Mat            Mat Mat      
    maDet maDet
       r r       maDet maDet              maDet maDet           maDet maDet        Unit Unit     |
| 340 | 324, 339 | mpbid 222 |
. . . 4
               Mat Mat              Mat Mat            Mat Mat            Mat Mat      
   maDet maDet        Unit Unit    |
| 341 | 315, 340 | sylanl1 682 |
. . 3
      Field Field         Mat Mat              Mat Mat            Mat Mat            Mat Mat          maDet maDet        Unit Unit    |
| 342 | 341 | ad4ant14 1293 |
. 2
        Field Field 
       Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod
   
        Mat Mat            Mat Mat            Mat Mat          maDet maDet        Unit Unit    |
| 343 | 314, 342 | rexlimddv 3035 |
1
       Field Field  
      Mat Mat           curry curry  LIndF LIndF   freeLMod freeLMod        maDet maDet        Unit Unit    |