Theorem ablfaclem3 18486

Description: Lemma for ablfac 18487. (Contributed by Mario Carneiro, 27-Apr-2016.) (Revised by Mario Carneiro, 3-May-2016.)

Hypotheses

Ref	Expression
ablfac.b	|- B = ( Base ` G )
ablfac.c	|- C = { r e. (SubGrp ` G ) | ( G ↾s r ) e. (CycGrp i^i ran pGrp ) }
ablfac.1	|- ( ph -> G e. Abel )
ablfac.2	|- ( ph -> B e. Fin )
ablfac.o	|- O = ( od ` G )
ablfac.a	|- A = { w e. Prime | w || ( # ` B ) }
ablfac.s	|- S = ( p e. A |-> { x e. B | ( O ` x ) || ( p ^ ( p pCnt ( # ` B ) ) ) } )
ablfac.w	|- W = ( g e. (SubGrp ` G ) |-> { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = g ) } )

Assertion

Ref	Expression
ablfaclem3	|- ( ph -> ( W ` B ) =/= (/) )

Distinct variable groups:   s, p, x, A   g, r, s, S   g, p, w, x, B, r, s   O, p, x   C, g, p, s, w, x   W, p, w, x   ph, p, s, w, x   g, G, p, r, s, w, x

Allowed substitution hints:   ph( g, r)   A( w, g, r)   C( r)   S( x, w, p)   O( w, g, s, r)   W( g, s, r)

Proof of Theorem ablfaclem3

Dummy variables a b c f h q t y are mutually distinct and distinct from all other variables.

Step	Hyp	Ref	Expression
1		fzfid 12772	. . . 4 |- ( ph -> ( 1 ... ( # ` B ) ) e. Fin )
2		ablfac.a	. . . . 5 |- A = { w e. Prime | w || ( # ` B ) }
3		prmnn 15388	. . . . . . . 8 |- ( w e. Prime -> w e. NN )
4	3	3ad2ant2 1083	. . . . . . 7 |- ( ( ph /\ w e. Prime /\ w || ( # ` B ) ) -> w e. NN )
5		prmz 15389	. . . . . . . . 9 |- ( w e. Prime -> w e. ZZ )
6		ablfac.1	. . . . . . . . . . 11 |- ( ph -> G e. Abel )
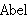7		ablgrp 18198	. . . . . . . . . . 11 |- ( G e. Abel -> G e. Grp )
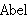
8		ablfac.b	. . . . . . . . . . . 12 |- B = ( Base ` G )
9	8	grpbn0 17451	. . . . . . . . . . 11 |- ( G e. Grp -> B =/= (/) )
10	6, 7, 9	3syl 18	. . . . . . . . . 10 |- ( ph -> B =/= (/) )
11		ablfac.2	. . . . . . . . . . 11 |- ( ph -> B e. Fin )
12		hashnncl 13157	. . . . . . . . . . 11 |- ( B e. Fin -> ( ( # ` B ) e. NN <-> B =/= (/) ) )
13	11, 12	syl 17	. . . . . . . . . 10 |- ( ph -> ( ( # ` B ) e. NN <-> B =/= (/) ) )
14	10, 13	mpbird 247	. . . . . . . . 9 |- ( ph -> ( # ` B ) e. NN )
15		dvdsle 15032	. . . . . . . . 9 |- ( ( w e. ZZ /\ ( # ` B ) e. NN ) -> ( w || ( # ` B ) -> w <_ ( # ` B ) ) )
16	5, 14, 15	syl2anr 495	. . . . . . . 8 |- ( ( ph /\ w e. Prime ) -> ( w || ( # ` B ) -> w <_ ( # ` B ) ) )
17	16	3impia 1261	. . . . . . 7 |- ( ( ph /\ w e. Prime /\ w || ( # ` B ) ) -> w <_ ( # ` B ) )
18	14	nnzd 11481	. . . . . . . . 9 |- ( ph -> ( # ` B ) e. ZZ )
19	18	3ad2ant1 1082	. . . . . . . 8 |- ( ( ph /\ w e. Prime /\ w || ( # ` B ) ) -> ( # ` B ) e. ZZ )
20		fznn 12408	. . . . . . . 8 |- ( ( # ` B ) e. ZZ -> ( w e. ( 1 ... ( # ` B ) ) <-> ( w e. NN /\ w <_ ( # ` B ) ) ) )
21	19, 20	syl 17	. . . . . . 7 |- ( ( ph /\ w e. Prime /\ w || ( # ` B ) ) -> ( w e. ( 1 ... ( # ` B ) ) <-> ( w e. NN /\ w <_ ( # ` B ) ) ) )
22	4, 17, 21	mpbir2and 957	. . . . . 6 |- ( ( ph /\ w e. Prime /\ w || ( # ` B ) ) -> w e. ( 1 ... ( # ` B ) ) )
23	22	rabssdv 3682	. . . . 5 |- ( ph -> { w e. Prime | w || ( # ` B ) } C_ ( 1 ... ( # ` B ) ) )
24	2, 23	syl5eqss 3649	. . . 4 |- ( ph -> A C_ ( 1 ... ( # ` B ) ) )
25		ssfi 8180	. . . 4 |- ( ( ( 1 ... ( # ` B ) ) e. Fin /\ A C_ ( 1 ... ( # ` B ) ) ) -> A e. Fin )
26	1, 24, 25	syl2anc 693	. . 3 |- ( ph -> A e. Fin )
27		dfin5 3582	. . . . . . . 8 |- (Word C i^i ( W ` ( S ` q ) ) ) = { y e. Word C | y e. ( W ` ( S ` q ) ) }
28		ablfac.o	. . . . . . . . . . . . . 14 |- O = ( od ` G )
29		ablfac.s	. . . . . . . . . . . . . 14 |- S = ( p e. A |-> { x e. B | ( O ` x ) || ( p ^ ( p pCnt ( # ` B ) ) ) } )
30		ssrab2 3687	. . . . . . . . . . . . . . . 16 |- { w e. Prime | w || ( # ` B ) } C_ Prime
31	2, 30	eqsstri 3635	. . . . . . . . . . . . . . 15 |- A C_ Prime
32	31	a1i 11	. . . . . . . . . . . . . 14 |- ( ph -> A C_ Prime )
33	8, 28, 29, 6, 11, 32	ablfac1b 18469	. . . . . . . . . . . . 13 |- ( ph -> G dom DProd S )
34		fvex 6201	. . . . . . . . . . . . . . . . 17 |- ( Base ` G ) e. _V
35	8, 34	eqeltri 2697	. . . . . . . . . . . . . . . 16 |- B e. _V
36	35	rabex 4813	. . . . . . . . . . . . . . 15 |- { x e. B | ( O ` x ) || ( p ^ ( p pCnt ( # ` B ) ) ) } e. _V
37	36, 29	dmmpti 6023	. . . . . . . . . . . . . 14 |- dom S = A
38	37	a1i 11	. . . . . . . . . . . . 13 |- ( ph -> dom S = A )
39	33, 38	dprdf2 18406	. . . . . . . . . . . 12 |- ( ph -> S : A --> (SubGrp ` G ) )
40	39	ffvelrnda 6359	. . . . . . . . . . 11 |- ( ( ph /\ q e. A ) -> ( S ` q ) e. (SubGrp ` G ) )
41		ablfac.c	. . . . . . . . . . . 12 |- C = { r e. (SubGrp ` G ) | ( G ↾s r ) e. (CycGrp i^i ran pGrp ) }
42		ablfac.w	. . . . . . . . . . . 12 |- W = ( g e. (SubGrp ` G ) |-> { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = g ) } )
43	8, 41, 6, 11, 28, 2, 29, 42	ablfaclem1 18484	. . . . . . . . . . 11 |- ( ( S ` q ) e. (SubGrp ` G ) -> ( W ` ( S ` q ) ) = { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) } )
44	40, 43	syl 17	. . . . . . . . . 10 |- ( ( ph /\ q e. A ) -> ( W ` ( S ` q ) ) = { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) } )
45		ssrab2 3687	. . . . . . . . . 10 |- { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) } C_ Word C
46	44, 45	syl6eqss 3655	. . . . . . . . 9 |- ( ( ph /\ q e. A ) -> ( W ` ( S ` q ) ) C_ Word C )
47		sseqin2 3817	. . . . . . . . 9 |- ( ( W ` ( S ` q ) ) C_ Word C <-> (Word C i^i ( W ` ( S ` q ) ) ) = ( W ` ( S ` q ) ) )
48	46, 47	sylib 208	. . . . . . . 8 |- ( ( ph /\ q e. A ) -> (Word C i^i ( W ` ( S ` q ) ) ) = ( W ` ( S ` q ) ) )
49	27, 48	syl5eqr 2670	. . . . . . 7 |- ( ( ph /\ q e. A ) -> { y e. Word C | y e. ( W ` ( S ` q ) ) } = ( W ` ( S ` q ) ) )
50	49, 44	eqtrd 2656	. . . . . 6 |- ( ( ph /\ q e. A ) -> { y e. Word C | y e. ( W ` ( S ` q ) ) } = { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) } )
51		eqid 2622	. . . . . . . . 9 |- ( Base ` ( G ↾s ( S ` q ) ) ) = ( Base ` ( G ↾s ( S ` q ) ) )
52		eqid 2622	. . . . . . . . 9 |- { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } = { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) }
53	6	adantr 481	. . . . . . . . . 10 |- ( ( ph /\ q e. A ) -> G e. Abel )
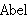54		eqid 2622	. . . . . . . . . . 11 |- ( G ↾s ( S ` q ) ) = ( G ↾s ( S ` q ) )
55	54	subgabl 18241	. . . . . . . . . 10 |- ( ( G e. Abel /\ ( S ` q ) e. (SubGrp ` G ) ) -> ( G ↾s ( S ` q ) ) e. Abel )
56	53, 40, 55	syl2anc 693	. . . . . . . . 9 |- ( ( ph /\ q e. A ) -> ( G ↾s ( S ` q ) ) e. Abel )
57	32	sselda 3603	. . . . . . . . . 10 |- ( ( ph /\ q e. A ) -> q e. Prime )
58	54	subgbas 17598	. . . . . . . . . . . . . 14 |- ( ( S ` q ) e. (SubGrp ` G ) -> ( S ` q ) = ( Base ` ( G ↾s ( S ` q ) ) ) )
59	40, 58	syl 17	. . . . . . . . . . . . 13 |- ( ( ph /\ q e. A ) -> ( S ` q ) = ( Base ` ( G ↾s ( S ` q ) ) ) )
60	59	fveq2d 6195	. . . . . . . . . . . 12 |- ( ( ph /\ q e. A ) -> ( # ` ( S ` q ) ) = ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) )
61	8, 28, 29, 6, 11, 32	ablfac1a 18468	. . . . . . . . . . . 12 |- ( ( ph /\ q e. A ) -> ( # ` ( S ` q ) ) = ( q ^ ( q pCnt ( # ` B ) ) ) )
62	60, 61	eqtr3d 2658	. . . . . . . . . . 11 |- ( ( ph /\ q e. A ) -> ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) = ( q ^ ( q pCnt ( # ` B ) ) ) )
63	62	oveq2d 6666	. . . . . . . . . . . . 13 |- ( ( ph /\ q e. A ) -> ( q pCnt ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) ) = ( q pCnt ( q ^ ( q pCnt ( # ` B ) ) ) ) )
64	14	adantr 481	. . . . . . . . . . . . . . . 16 |- ( ( ph /\ q e. A ) -> ( # ` B ) e. NN )
65	57, 64	pccld 15555	. . . . . . . . . . . . . . 15 |- ( ( ph /\ q e. A ) -> ( q pCnt ( # ` B ) ) e. NN0 )
66	65	nn0zd 11480	. . . . . . . . . . . . . 14 |- ( ( ph /\ q e. A ) -> ( q pCnt ( # ` B ) ) e. ZZ )
67		pcid 15577	. . . . . . . . . . . . . 14 |- ( ( q e. Prime /\ ( q pCnt ( # ` B ) ) e. ZZ ) -> ( q pCnt ( q ^ ( q pCnt ( # ` B ) ) ) ) = ( q pCnt ( # ` B ) ) )
68	57, 66, 67	syl2anc 693	. . . . . . . . . . . . 13 |- ( ( ph /\ q e. A ) -> ( q pCnt ( q ^ ( q pCnt ( # ` B ) ) ) ) = ( q pCnt ( # ` B ) ) )
69	63, 68	eqtrd 2656	. . . . . . . . . . . 12 |- ( ( ph /\ q e. A ) -> ( q pCnt ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) ) = ( q pCnt ( # ` B ) ) )
70	69	oveq2d 6666	. . . . . . . . . . 11 |- ( ( ph /\ q e. A ) -> ( q ^ ( q pCnt ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) ) ) = ( q ^ ( q pCnt ( # ` B ) ) ) )
71	62, 70	eqtr4d 2659	. . . . . . . . . 10 |- ( ( ph /\ q e. A ) -> ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) = ( q ^ ( q pCnt ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) ) ) )
72	54	subggrp 17597	. . . . . . . . . . . 12 |- ( ( S ` q ) e. (SubGrp ` G ) -> ( G ↾s ( S ` q ) ) e. Grp )
73	40, 72	syl 17	. . . . . . . . . . 11 |- ( ( ph /\ q e. A ) -> ( G ↾s ( S ` q ) ) e. Grp )
74	11	adantr 481	. . . . . . . . . . . . 13 |- ( ( ph /\ q e. A ) -> B e. Fin )
75	8	subgss 17595	. . . . . . . . . . . . . 14 |- ( ( S ` q ) e. (SubGrp ` G ) -> ( S ` q ) C_ B )
76	40, 75	syl 17	. . . . . . . . . . . . 13 |- ( ( ph /\ q e. A ) -> ( S ` q ) C_ B )
77		ssfi 8180	. . . . . . . . . . . . 13 |- ( ( B e. Fin /\ ( S ` q ) C_ B ) -> ( S ` q ) e. Fin )
78	74, 76, 77	syl2anc 693	. . . . . . . . . . . 12 |- ( ( ph /\ q e. A ) -> ( S ` q ) e. Fin )
79	59, 78	eqeltrrd 2702	. . . . . . . . . . 11 |- ( ( ph /\ q e. A ) -> ( Base ` ( G ↾s ( S ` q ) ) ) e. Fin )
80	51	pgpfi2 18021	. . . . . . . . . . 11 |- ( ( ( G ↾s ( S ` q ) ) e. Grp /\ ( Base ` ( G ↾s ( S ` q ) ) ) e. Fin ) -> ( q pGrp ( G ↾s ( S ` q ) ) <-> ( q e. Prime /\ ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) = ( q ^ ( q pCnt ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) ) ) ) ) )
81	73, 79, 80	syl2anc 693	. . . . . . . . . 10 |- ( ( ph /\ q e. A ) -> ( q pGrp ( G ↾s ( S ` q ) ) <-> ( q e. Prime /\ ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) = ( q ^ ( q pCnt ( # ` ( Base ` ( G ↾s ( S ` q ) ) ) ) ) ) ) ) )
82	57, 71, 81	mpbir2and 957	. . . . . . . . 9 |- ( ( ph /\ q e. A ) -> q pGrp ( G ↾s ( S ` q ) ) )
83	51, 52, 56, 82, 79	pgpfac 18483	. . . . . . . 8 |- ( ( ph /\ q e. A ) -> E. s e. Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } ( ( G ↾s ( S ` q ) ) dom DProd s /\ ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) ) )
84		ssrab2 3687	. . . . . . . . . . . . . 14 |- { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ (SubGrp ` ( G ↾s ( S ` q ) ) )
85		sswrd 13313	. . . . . . . . . . . . . 14 |- ( { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ (SubGrp ` ( G ↾s ( S ` q ) ) ) -> Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ Word (SubGrp ` ( G ↾s ( S ` q ) ) ) )
86	84, 85	ax-mp 5	. . . . . . . . . . . . 13 |- Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ Word (SubGrp ` ( G ↾s ( S ` q ) ) )
87	86	sseli 3599	. . . . . . . . . . . 12 |- ( s e. Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } -> s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) )
88	40	adantr 481	. . . . . . . . . . . . . . . . . 18 |- ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) -> ( S ` q ) e. (SubGrp ` G ) )
89	88	adantr 481	. . . . . . . . . . . . . . . . 17 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ( S ` q ) e. (SubGrp ` G ) )
90	54	subgdmdprd 18433	. . . . . . . . . . . . . . . . . . 19 |- ( ( S ` q ) e. (SubGrp ` G ) -> ( ( G ↾s ( S ` q ) ) dom DProd s <-> ( G dom DProd s /\ ran s C_ ~P ( S ` q ) ) ) )
91	88, 90	syl 17	. . . . . . . . . . . . . . . . . 18 |- ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) -> ( ( G ↾s ( S ` q ) ) dom DProd s <-> ( G dom DProd s /\ ran s C_ ~P ( S ` q ) ) ) )
92	91	simprbda 653	. . . . . . . . . . . . . . . . 17 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> G dom DProd s )
93	91	simplbda 654	. . . . . . . . . . . . . . . . 17 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ran s C_ ~P ( S ` q ) )
94	54, 89, 92, 93	subgdprd 18434	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ( ( G ↾s ( S ` q ) ) DProd s ) = ( G DProd s ) )
95	59	ad2antrr 762	. . . . . . . . . . . . . . . . 17 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ( S ` q ) = ( Base ` ( G ↾s ( S ` q ) ) ) )
96	95	eqcomd 2628	. . . . . . . . . . . . . . . 16 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ( Base ` ( G ↾s ( S ` q ) ) ) = ( S ` q ) )
97	94, 96	eqeq12d 2637	. . . . . . . . . . . . . . 15 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ( ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) <-> ( G DProd s ) = ( S ` q ) ) )
98	97	biimpd 219	. . . . . . . . . . . . . 14 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ( ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) -> ( G DProd s ) = ( S ` q ) ) )
99	98, 92	jctild 566	. . . . . . . . . . . . 13 |- ( ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) /\ ( G ↾s ( S ` q ) ) dom DProd s ) -> ( ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) -> ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) ) )
100	99	expimpd 629	. . . . . . . . . . . 12 |- ( ( ( ph /\ q e. A ) /\ s e. Word (SubGrp ` ( G ↾s ( S ` q ) ) ) ) -> ( ( ( G ↾s ( S ` q ) ) dom DProd s /\ ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) ) -> ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) ) )
101	87, 100	sylan2 491	. . . . . . . . . . 11 |- ( ( ( ph /\ q e. A ) /\ s e. Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } ) -> ( ( ( G ↾s ( S ` q ) ) dom DProd s /\ ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) ) -> ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) ) )
102		oveq2 6658	. . . . . . . . . . . . . . . 16 |- ( r = y -> ( ( G ↾s ( S ` q ) ) ↾s r ) = ( ( G ↾s ( S ` q ) ) ↾s y ) )
103	102	eleq1d 2686	. . . . . . . . . . . . . . 15 |- ( r = y -> ( ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) <-> ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) )
104	103	cbvrabv 3199	. . . . . . . . . . . . . 14 |- { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } = { y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) }
105	54	subsubg 17617	. . . . . . . . . . . . . . . . . . 19 |- ( ( S ` q ) e. (SubGrp ` G ) -> ( y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) <-> ( y e. (SubGrp ` G ) /\ y C_ ( S ` q ) ) ) )
106	40, 105	syl 17	. . . . . . . . . . . . . . . . . 18 |- ( ( ph /\ q e. A ) -> ( y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) <-> ( y e. (SubGrp ` G ) /\ y C_ ( S ` q ) ) ) )
107	106	simprbda 653	. . . . . . . . . . . . . . . . 17 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) ) -> y e. (SubGrp ` G ) )
108	107	3adant3 1081	. . . . . . . . . . . . . . . 16 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) /\ ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) -> y e. (SubGrp ` G ) )
109	40	3ad2ant1 1082	. . . . . . . . . . . . . . . . . 18 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) /\ ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) -> ( S ` q ) e. (SubGrp ` G ) )
110	106	simplbda 654	. . . . . . . . . . . . . . . . . . 19 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) ) -> y C_ ( S ` q ) )
111	110	3adant3 1081	. . . . . . . . . . . . . . . . . 18 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) /\ ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) -> y C_ ( S ` q ) )
112		ressabs 15939	. . . . . . . . . . . . . . . . . 18 |- ( ( ( S ` q ) e. (SubGrp ` G ) /\ y C_ ( S ` q ) ) -> ( ( G ↾s ( S ` q ) ) ↾s y ) = ( G ↾s y ) )
113	109, 111, 112	syl2anc 693	. . . . . . . . . . . . . . . . 17 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) /\ ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) -> ( ( G ↾s ( S ` q ) ) ↾s y ) = ( G ↾s y ) )
114		simp3 1063	. . . . . . . . . . . . . . . . 17 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) /\ ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) -> ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) )
115	113, 114	eqeltrrd 2702	. . . . . . . . . . . . . . . 16 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) /\ ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) -> ( G ↾s y ) e. (CycGrp i^i ran pGrp ) )
116		oveq2 6658	. . . . . . . . . . . . . . . . . 18 |- ( r = y -> ( G ↾s r ) = ( G ↾s y ) )
117	116	eleq1d 2686	. . . . . . . . . . . . . . . . 17 |- ( r = y -> ( ( G ↾s r ) e. (CycGrp i^i ran pGrp ) <-> ( G ↾s y ) e. (CycGrp i^i ran pGrp ) ) )
118	117, 41	elrab2 3366	. . . . . . . . . . . . . . . 16 |- ( y e. C <-> ( y e. (SubGrp ` G ) /\ ( G ↾s y ) e. (CycGrp i^i ran pGrp ) ) )
119	108, 115, 118	sylanbrc 698	. . . . . . . . . . . . . . 15 |- ( ( ( ph /\ q e. A ) /\ y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) /\ ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) ) -> y e. C )
120	119	rabssdv 3682	. . . . . . . . . . . . . 14 |- ( ( ph /\ q e. A ) -> { y e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s y ) e. (CycGrp i^i ran pGrp ) } C_ C )
121	104, 120	syl5eqss 3649	. . . . . . . . . . . . 13 |- ( ( ph /\ q e. A ) -> { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ C )
122		sswrd 13313	. . . . . . . . . . . . 13 |- ( { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ C -> Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ Word C )
123	121, 122	syl 17	. . . . . . . . . . . 12 |- ( ( ph /\ q e. A ) -> Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } C_ Word C )
124	123	sselda 3603	. . . . . . . . . . 11 |- ( ( ( ph /\ q e. A ) /\ s e. Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } ) -> s e. Word C )
125	101, 124	jctild 566	. . . . . . . . . 10 |- ( ( ( ph /\ q e. A ) /\ s e. Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } ) -> ( ( ( G ↾s ( S ` q ) ) dom DProd s /\ ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) ) -> ( s e. Word C /\ ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) ) ) )
126	125	expimpd 629	. . . . . . . . 9 |- ( ( ph /\ q e. A ) -> ( ( s e. Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } /\ ( ( G ↾s ( S ` q ) ) dom DProd s /\ ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) ) ) -> ( s e. Word C /\ ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) ) ) )
127	126	reximdv2 3014	. . . . . . . 8 |- ( ( ph /\ q e. A ) -> ( E. s e. Word { r e. (SubGrp ` ( G ↾s ( S ` q ) ) ) | ( ( G ↾s ( S ` q ) ) ↾s r ) e. (CycGrp i^i ran pGrp ) } ( ( G ↾s ( S ` q ) ) dom DProd s /\ ( ( G ↾s ( S ` q ) ) DProd s ) = ( Base ` ( G ↾s ( S ` q ) ) ) ) -> E. s e. Word C ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) ) )
128	83, 127	mpd 15	. . . . . . 7 |- ( ( ph /\ q e. A ) -> E. s e. Word C ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) )
129		rabn0 3958	. . . . . . 7 |- ( { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) } =/= (/) <-> E. s e. Word C ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) )
130	128, 129	sylibr 224	. . . . . 6 |- ( ( ph /\ q e. A ) -> { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = ( S ` q ) ) } =/= (/) )
131	50, 130	eqnetrd 2861	. . . . 5 |- ( ( ph /\ q e. A ) -> { y e. Word C | y e. ( W ` ( S ` q ) ) } =/= (/) )
132		rabn0 3958	. . . . 5 |- ( { y e. Word C | y e. ( W ` ( S ` q ) ) } =/= (/) <-> E. y e. Word C y e. ( W ` ( S ` q ) ) )
133	131, 132	sylib 208	. . . 4 |- ( ( ph /\ q e. A ) -> E. y e. Word C y e. ( W ` ( S ` q ) ) )
134	133	ralrimiva 2966	. . 3 |- ( ph -> A. q e. A E. y e. Word C y e. ( W ` ( S ` q ) ) )
135		eleq1 2689	. . . 4 |- ( y = ( f ` q ) -> ( y e. ( W ` ( S ` q ) ) <-> ( f ` q ) e. ( W ` ( S ` q ) ) ) )
136	135	ac6sfi 8204	. . 3 |- ( ( A e. Fin /\ A. q e. A E. y e. Word C y e. ( W ` ( S ` q ) ) ) -> E. f ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) )
137	26, 134, 136	syl2anc 693	. 2 |- ( ph -> E. f ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) )
138		sneq 4187	. . . . . . . . 9 |- ( q = y -> { q } = { y } )
139		fveq2 6191	. . . . . . . . . 10 |- ( q = y -> ( f ` q ) = ( f ` y ) )
140	139	dmeqd 5326	. . . . . . . . 9 |- ( q = y -> dom ( f ` q ) = dom ( f ` y ) )
141	138, 140	xpeq12d 5140	. . . . . . . 8 |- ( q = y -> ( { q } X. dom ( f ` q ) ) = ( { y } X. dom ( f ` y ) ) )
142	141	cbviunv 4559	. . . . . . 7 |- U_ q e. A ( { q } X. dom ( f ` q ) ) = U_ y e. A ( { y } X. dom ( f ` y ) )
143	26	adantr 481	. . . . . . . 8 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> A e. Fin )
144		snfi 8038	. . . . . . . . . 10 |- { y } e. Fin
145		simprl 794	. . . . . . . . . . . . 13 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> f : A -->Word C )
146	145	ffvelrnda 6359	. . . . . . . . . . . 12 |- ( ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) /\ y e. A ) -> ( f ` y ) e. Word C )
147		wrdf 13310	. . . . . . . . . . . 12 |- ( ( f ` y ) e. Word C -> ( f ` y ) : ( 0..^ ( # ` ( f ` y ) ) ) --> C )
148		fdm 6051	. . . . . . . . . . . 12 |- ( ( f ` y ) : ( 0..^ ( # ` ( f ` y ) ) ) --> C -> dom ( f ` y ) = ( 0..^ ( # ` ( f ` y ) ) ) )
149	146, 147, 148	3syl 18	. . . . . . . . . . 11 |- ( ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) /\ y e. A ) -> dom ( f ` y ) = ( 0..^ ( # ` ( f ` y ) ) ) )
150		fzofi 12773	. . . . . . . . . . 11 |- ( 0..^ ( # ` ( f ` y ) ) ) e. Fin
151	149, 150	syl6eqel 2709	. . . . . . . . . 10 |- ( ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) /\ y e. A ) -> dom ( f ` y ) e. Fin )
152		xpfi 8231	. . . . . . . . . 10 |- ( ( { y } e. Fin /\ dom ( f ` y ) e. Fin ) -> ( { y } X. dom ( f ` y ) ) e. Fin )
153	144, 151, 152	sylancr 695	. . . . . . . . 9 |- ( ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) /\ y e. A ) -> ( { y } X. dom ( f ` y ) ) e. Fin )
154	153	ralrimiva 2966	. . . . . . . 8 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> A. y e. A ( { y } X. dom ( f ` y ) ) e. Fin )
155		iunfi 8254	. . . . . . . 8 |- ( ( A e. Fin /\ A. y e. A ( { y } X. dom ( f ` y ) ) e. Fin ) -> U_ y e. A ( { y } X. dom ( f ` y ) ) e. Fin )
156	143, 154, 155	syl2anc 693	. . . . . . 7 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> U_ y e. A ( { y } X. dom ( f ` y ) ) e. Fin )
157	142, 156	syl5eqel 2705	. . . . . 6 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> U_ q e. A ( { q } X. dom ( f ` q ) ) e. Fin )
158		hashcl 13147	. . . . . 6 |- ( U_ q e. A ( { q } X. dom ( f ` q ) ) e. Fin -> ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) e. NN0 )
159		hashfzo0 13217	. . . . . 6 |- ( ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) e. NN0 -> ( # ` ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ) = ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) )
160	157, 158, 159	3syl 18	. . . . 5 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> ( # ` ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ) = ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) )
161		fzofi 12773	. . . . . 6 |- ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) e. Fin
162		hashen 13135	. . . . . 6 |- ( ( ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) e. Fin /\ U_ q e. A ( { q } X. dom ( f ` q ) ) e. Fin ) -> ( ( # ` ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ) = ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) <-> ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ~~ U_ q e. A ( { q } X. dom ( f ` q ) ) ) )
163	161, 157, 162	sylancr 695	. . . . 5 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> ( ( # ` ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ) = ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) <-> ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ~~ U_ q e. A ( { q } X. dom ( f ` q ) ) ) )
164	160, 163	mpbid 222	. . . 4 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ~~ U_ q e. A ( { q } X. dom ( f ` q ) ) )
165		bren 7964	. . . 4 |- ( ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) ~~ U_ q e. A ( { q } X. dom ( f ` q ) ) <-> E. h h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) )
166	164, 165	sylib 208	. . 3 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> E. h h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) )
167	6	adantr 481	. . . . . 6 |- ( ( ph /\ ( ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) /\ h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -> G e. Abel )
168	11	adantr 481	. . . . . 6 |- ( ( ph /\ ( ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) /\ h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -> B e. Fin )
169		breq1 4656	. . . . . . . 8 |- ( w = a -> ( w || ( # ` B ) <-> a || ( # ` B ) ) )
170	169	cbvrabv 3199	. . . . . . 7 |- { w e. Prime | w || ( # ` B ) } = { a e. Prime | a || ( # ` B ) }
171	2, 170	eqtri 2644	. . . . . 6 |- A = { a e. Prime | a || ( # ` B ) }
172		fveq2 6191	. . . . . . . . . . 11 |- ( x = c -> ( O ` x ) = ( O ` c ) )
173	172	breq1d 4663	. . . . . . . . . 10 |- ( x = c -> ( ( O ` x ) || ( p ^ ( p pCnt ( # ` B ) ) ) <-> ( O ` c ) || ( p ^ ( p pCnt ( # ` B ) ) ) ) )
174	173	cbvrabv 3199	. . . . . . . . 9 |- { x e. B | ( O ` x ) || ( p ^ ( p pCnt ( # ` B ) ) ) } = { c e. B | ( O ` c ) || ( p ^ ( p pCnt ( # ` B ) ) ) }
175		id 22	. . . . . . . . . . . 12 |- ( p = b -> p = b )
176		oveq1 6657	. . . . . . . . . . . 12 |- ( p = b -> ( p pCnt ( # ` B ) ) = ( b pCnt ( # ` B ) ) )
177	175, 176	oveq12d 6668	. . . . . . . . . . 11 |- ( p = b -> ( p ^ ( p pCnt ( # ` B ) ) ) = ( b ^ ( b pCnt ( # ` B ) ) ) )
178	177	breq2d 4665	. . . . . . . . . 10 |- ( p = b -> ( ( O ` c ) || ( p ^ ( p pCnt ( # ` B ) ) ) <-> ( O ` c ) || ( b ^ ( b pCnt ( # ` B ) ) ) ) )
179	178	rabbidv 3189	. . . . . . . . 9 |- ( p = b -> { c e. B | ( O ` c ) || ( p ^ ( p pCnt ( # ` B ) ) ) } = { c e. B | ( O ` c ) || ( b ^ ( b pCnt ( # ` B ) ) ) } )
180	174, 179	syl5eq 2668	. . . . . . . 8 |- ( p = b -> { x e. B | ( O ` x ) || ( p ^ ( p pCnt ( # ` B ) ) ) } = { c e. B | ( O ` c ) || ( b ^ ( b pCnt ( # ` B ) ) ) } )
181	180	cbvmptv 4750	. . . . . . 7 |- ( p e. A |-> { x e. B | ( O ` x ) || ( p ^ ( p pCnt ( # ` B ) ) ) } ) = ( b e. A |-> { c e. B | ( O ` c ) || ( b ^ ( b pCnt ( # ` B ) ) ) } )
182	29, 181	eqtri 2644	. . . . . 6 |- S = ( b e. A |-> { c e. B | ( O ` c ) || ( b ^ ( b pCnt ( # ` B ) ) ) } )
183		breq2 4657	. . . . . . . . . 10 |- ( s = t -> ( G dom DProd s <-> G dom DProd t ) )
184		oveq2 6658	. . . . . . . . . . 11 |- ( s = t -> ( G DProd s ) = ( G DProd t ) )
185	184	eqeq1d 2624	. . . . . . . . . 10 |- ( s = t -> ( ( G DProd s ) = g <-> ( G DProd t ) = g ) )
186	183, 185	anbi12d 747	. . . . . . . . 9 |- ( s = t -> ( ( G dom DProd s /\ ( G DProd s ) = g ) <-> ( G dom DProd t /\ ( G DProd t ) = g ) ) )
187	186	cbvrabv 3199	. . . . . . . 8 |- { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = g ) } = { t e. Word C | ( G dom DProd t /\ ( G DProd t ) = g ) }
188	187	mpteq2i 4741	. . . . . . 7 |- ( g e. (SubGrp ` G ) |-> { s e. Word C | ( G dom DProd s /\ ( G DProd s ) = g ) } ) = ( g e. (SubGrp ` G ) |-> { t e. Word C | ( G dom DProd t /\ ( G DProd t ) = g ) } )
189	42, 188	eqtri 2644	. . . . . 6 |- W = ( g e. (SubGrp ` G ) |-> { t e. Word C | ( G dom DProd t /\ ( G DProd t ) = g ) } )
190		simprll 802	. . . . . 6 |- ( ( ph /\ ( ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) /\ h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -> f : A -->Word C )
191		simprlr 803	. . . . . . 7 |- ( ( ph /\ ( ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) /\ h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -> A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) )
192		fveq2 6191	. . . . . . . . . 10 |- ( q = y -> ( S ` q ) = ( S ` y ) )
193	192	fveq2d 6195	. . . . . . . . 9 |- ( q = y -> ( W ` ( S ` q ) ) = ( W ` ( S ` y ) ) )
194	139, 193	eleq12d 2695	. . . . . . . 8 |- ( q = y -> ( ( f ` q ) e. ( W ` ( S ` q ) ) <-> ( f ` y ) e. ( W ` ( S ` y ) ) ) )
195	194	cbvralv 3171	. . . . . . 7 |- ( A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) <-> A. y e. A ( f ` y ) e. ( W ` ( S ` y ) ) )
196	191, 195	sylib 208	. . . . . 6 |- ( ( ph /\ ( ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) /\ h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -> A. y e. A ( f ` y ) e. ( W ` ( S ` y ) ) )
197		simprr 796	. . . . . 6 |- ( ( ph /\ ( ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) /\ h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -> h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) )
198	8, 41, 167, 168, 28, 171, 182, 189, 190, 196, 142, 197	ablfaclem2 18485	. . . . 5 |- ( ( ph /\ ( ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) /\ h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -> ( W ` B ) =/= (/) )
199	198	expr 643	. . . 4 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> ( h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) -> ( W ` B ) =/= (/) ) )
200	199	exlimdv 1861	. . 3 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> ( E. h h : ( 0..^ ( # ` U_ q e. A ( { q } X. dom ( f ` q ) ) ) ) -1-1-onto-> U_ q e. A ( { q } X. dom ( f ` q ) ) -> ( W ` B ) =/= (/) ) )
201	166, 200	mpd 15	. 2 |- ( ( ph /\ ( f : A -->Word C /\ A. q e. A ( f ` q ) e. ( W ` ( S ` q ) ) ) ) -> ( W ` B ) =/= (/) )
202	137, 201	exlimddv 1863	1 |- ( ph -> ( W ` B ) =/= (/) )

Syntax hints:   -> wi 4   <-> wb 196   /\ wa 384   /\ w3a 1037   = wceq 1483   E.wex 1704   e. wcel 1990   =/= wne 2794   A.wral 2912   E.wrex 2913   {crab 2916   _Vcvv 3200   i^i cin 3573   C_ wss 3574   (/)c0 3915   ~Pcpw 4158   {csn 4177   U_ciun 4520   class class class wbr 4653   |-> cmpt 4729   X. cxp 5112   dom cdm 5114   ran crn 5115   -->wf 5884   -1-1-onto->wf1o 5887   ` cfv 5888  (class class class)co 6650   ~~ cen 7952   Fincfn 7955   0cc0 9936   1c1 9937   <_ cle 10075   NNcn 11020   NN0cn0 11292   ZZcz 11377   ...cfz 12326  ..^cfzo 12465   ^cexp 12860   #chash 13117  Word cword 13291   || cdvds 14983   Primecprime 15385   pCnt cpc 15541   Basecbs 15857   ↾_s cress 15858   Grpcgrp 17422  SubGrpcsubg 17588   odcod 17944   pGrp cpgp 17946   Abelcabl 18194  CycGrpccyg 18279   DProd cdprd 18392

This theorem was proved from axioms:  ax-mp 5  ax-1 6  ax-2 7  ax-3 8  ax-gen 1722  ax-4 1737  ax-5 1839  ax-6 1888  ax-7 1935  ax-8 1992  ax-9 1999  ax-10 2019  ax-11 2034  ax-12 2047  ax-13 2246  ax-ext 2602  ax-rep 4771  ax-sep 4781  ax-nul 4789  ax-pow 4843  ax-pr 4906  ax-un 6949  ax-inf2 8538  ax-cnex 9992  ax-resscn 9993  ax-1cn 9994  ax-icn 9995  ax-addcl 9996  ax-addrcl 9997  ax-mulcl 9998  ax-mulrcl 9999  ax-mulcom 10000  ax-addass 10001  ax-mulass 10002  ax-distr 10003  ax-i2m1 10004  ax-1ne0 10005  ax-1rid 10006  ax-rnegex 10007  ax-rrecex 10008  ax-cnre 10009  ax-pre-lttri 10010  ax-pre-lttrn 10011  ax-pre-ltadd 10012  ax-pre-mulgt0 10013  ax-pre-sup 10014

This theorem depends on definitions:  df-bi 197  df-or 385  df-an 386  df-3or 1038  df-3an 1039  df-tru 1486  df-fal 1489  df-ex 1705  df-nf 1710  df-sb 1881  df-eu 2474  df-mo 2475  df-clab 2609  df-cleq 2615  df-clel 2618  df-nfc 2753  df-ne 2795  df-nel 2898  df-ral 2917  df-rex 2918  df-reu 2919  df-rmo 2920  df-rab 2921  df-v 3202  df-sbc 3436  df-csb 3534  df-dif 3577  df-un 3579  df-in 3581  df-ss 3588  df-pss 3590  df-nul 3916  df-if 4087  df-pw 4160  df-sn 4178  df-pr 4180  df-tp 4182  df-op 4184  df-uni 4437  df-int 4476  df-iun 4522  df-iin 4523  df-disj 4621  df-br 4654  df-opab 4713  df-mpt 4730  df-tr 4753  df-id 5024  df-eprel 5029  df-po 5035  df-so 5036  df-fr 5073  df-se 5074  df-we 5075  df-xp 5120  df-rel 5121  df-cnv 5122  df-co 5123  df-dm 5124  df-rn 5125  df-res 5126  df-ima 5127  df-pred 5680  df-ord 5726  df-on 5727  df-lim 5728  df-suc 5729  df-iota 5851  df-fun 5890  df-fn 5891  df-f 5892  df-f1 5893  df-fo 5894  df-f1o 5895  df-fv 5896  df-isom 5897  df-riota 6611  df-ov 6653  df-oprab 6654  df-mpt2 6655  df-of 6897  df-rpss 6937  df-om 7066  df-1st 7168  df-2nd 7169  df-supp 7296  df-tpos 7352  df-wrecs 7407  df-recs 7468  df-rdg 7506  df-1o 7560  df-2o 7561  df-oadd 7564  df-omul 7565  df-er 7742  df-ec 7744  df-qs 7748  df-map 7859  df-pm 7860  df-ixp 7909  df-en 7956  df-dom 7957  df-sdom 7958  df-fin 7959  df-fsupp 8276  df-sup 8348  df-inf 8349  df-oi 8415  df-card 8765  df-acn 8768  df-cda 8990  df-pnf 10076  df-mnf 10077  df-xr 10078  df-ltxr 10079  df-le 10080  df-sub 10268  df-neg 10269  df-div 10685  df-nn 11021  df-2 11079  df-3 11080  df-n0 11293  df-xnn0 11364  df-z 11378  df-uz 11688  df-q 11789  df-rp 11833  df-fz 12327  df-fzo 12466  df-fl 12593  df-mod 12669  df-seq 12802  df-exp 12861  df-fac 13061  df-bc 13090  df-hash 13118  df-word 13299  df-concat 13301  df-s1 13302  df-cj 13839  df-re 13840  df-im 13841  df-sqrt 13975  df-abs 13976  df-clim 14219  df-sum 14417  df-dvds 14984  df-gcd 15217  df-prm 15386  df-pc 15542  df-ndx 15860  df-slot 15861  df-base 15863  df-sets 15864  df-ress 15865  df-plusg 15954  df-0g 16102  df-gsum 16103  df-mre 16246  df-mrc 16247  df-acs 16249  df-mgm 17242  df-sgrp 17284  df-mnd 17295  df-mhm 17335  df-submnd 17336  df-grp 17425  df-minusg 17426  df-sbg 17427  df-mulg 17541  df-subg 17591  df-eqg 17593  df-ghm 17658  df-gim 17701  df-ga 17723  df-cntz 17750  df-oppg 17776  df-od 17948  df-gex 17949  df-pgp 17950  df-lsm 18051  df-pj1 18052  df-cmn 18195  df-abl 18196  df-cyg 18280  df-dprd 18394

This theorem is referenced by:  ablfac  18487